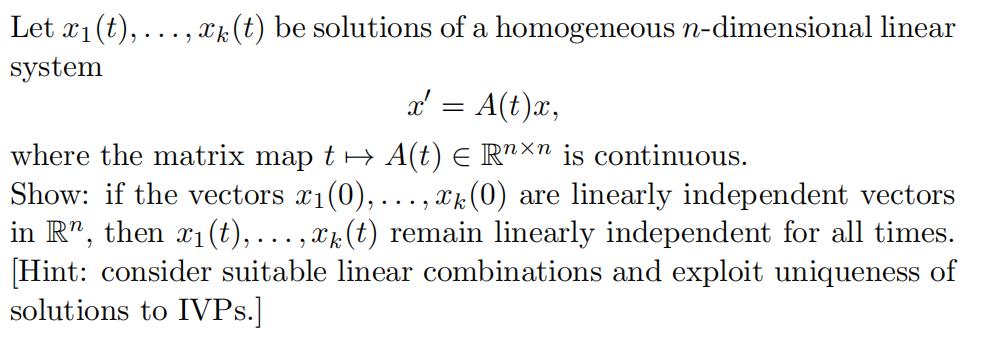
Let x (t),..., xk (t) be solutions of a homogeneous n-dimensional linear system x' = A(t)x, where the matrix map t A(t) = Rnn is continuous. Show: if the vectors x1(0), ..., xk (0) are linearly independent vectors in R", then x(t),...,xk (t) remain linearly independent for all times. [Hint: consider suitable linear combinations and exploit uniqueness of solutions to IVPs.]