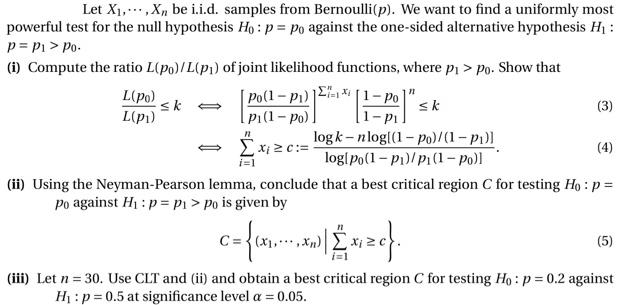
Let X, X, be i.i.d. samples from Bernoulli(p). We want to find a uniformly most powerful test for the null hypothesis Ho: p = po against the one-sided alternative hypothesis H: P= P1 > Po. (i) Compute the ratio L(po)/L(p) of joint likelihood functions, where p> po. Show that 11- Po L(po) L(p) sk Po(1-P) pi(1-po). Exzc: i=1 sk logk-nlog[(1-Po)/(1-P)] log[po(1-P)/pi(1-po)] (3) (4) (ii) Using the Neyman-Pearson lemma, conclude that a best critical region C for testing Ho: p = Po against H : p = P > Po is given by c={(x,x) | Ex=c}. C= (iii) Let n = 30. Use CLT and (ii) and obtain a best critical region C for testing Ho: p = 0.2 against H: p=0.5 at significance level a = 0.05. (5)