Question: Let X1, X2, ..., X, be a random sample from the exponential distribution with rate X. We will consider estimating the mean 7(A) =

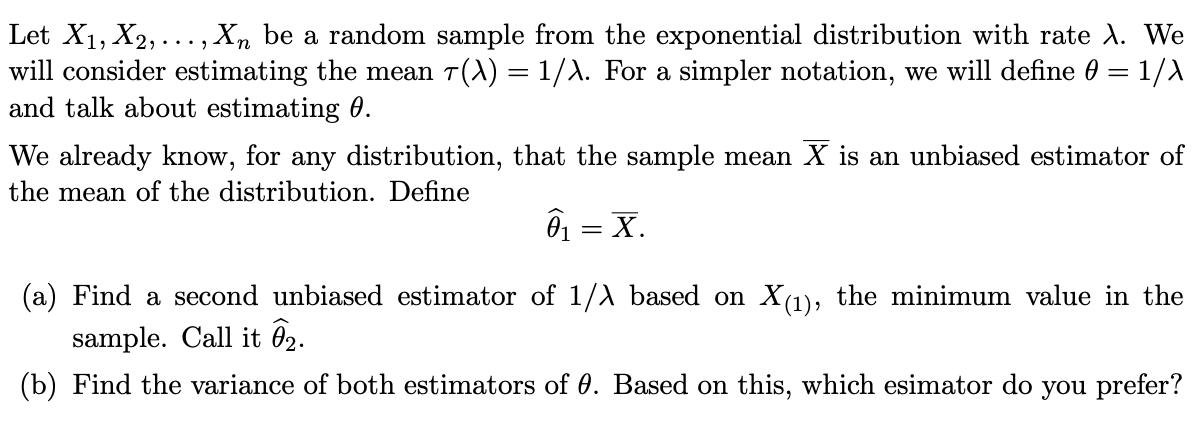
Let X1, X2, ..., X, be a random sample from the exponential distribution with rate X. We will consider estimating the mean 7(A) = 1/A. For a simpler notation, we will define 0 = 1/A and talk about estimating 0. We already know, for any distribution, that the sample mean X is an unbiased estimator of the mean of the distribution. Define 01 = X. %3D (a) Find a second unbiased estimator of 1/A based on X(1), the minimum value in the sample. Call it 02. (b) Find the variance of both estimators of 0. Based on this, which esimator do you prefer?

Step by Step Solution

★★★★★

3.39 Rating (146 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Let X1,..., Xn be a random sample from the pdf f(x|) = e-(x-) where -

Let X1,..., Xn be a random sample from the pdf f(x|) = x-2, 0

Let X1,.., Xn be a random sample from a population with mean Î¼ and variance Ï2. (a) Show that the estimator is an unbiased estimator of Î¼ if (b) Among all unbiased...

Help me out of these queries 1 A random sample from a Poisson(//) distribution is as follows: 4, 2, 7, 3, 1, 2, 5, 4, 0, 2 Calculate the method of moments estimate for /. 2 Using the method of...

Set Student Name: 1. Answer true or false for each part, and if false, explain your answer. a. The point estimate for the population mean, , of an x distribution is x-bar, computed from a random...

Describing Data Once we have collected data from surveys or experiments, we need to summarize and present the data in a way that will be meaningful to the reader. We will begin with graphical...

You own a small storefront retail business and are interested in determining the average amount of money a typical customer spends per visit to your store. You take a random sample over the course of...

1 2.3 Definition of a Discrete Probability Function Definition: Let S be a discrete sample space from some experiment. A function P, defined on all events in S, is said to be a probability function...

Probability and Statistics - Problem Set c Keith M. Chugg October 2, 2015 1 Preliminaries, Combinatorics, Set Probability 1.1. A number of bats are in a cave. 2 bats can see out of their left eye. 3...

Algorithms in Artificial Intelligence (or, the old name: Introduction to Algorithmic Decision Making) Part 1 Based on slides by David Sarne and Lirong Xia Course Tentative Schedule Introduction...

Suppose that investment demand in a given economy is predicted to be weak next year, say 10 percent below this year's level, because of an exogenous shock. All other components of aggregate demand...

What is the optimal length of time over which to take an average of historic sales when using the average approach?

Which of the following represents the formula for WACC? Option A WACC = ( E / V ) re + ( D / V ) rd Option B WACC = ( E / V ) re + ( D / V ) rd ( 1 Tc ) Option C WACC = ( E / V ) re ( D / V ) rd...

You participated in a brainstorm to gather information for an update to your CIO. Consider your findings of vendor options and the process for a health care information system as you begin your...

Purpose ACCT 207 PROJECT Adjusting Journal Entries and Financial Statements Improve your understanding of the relationships between the financial statements; increase your confidence in preparing and...

Ron Mason's Problem Ron Mason would like to determine how many Cushion - Air - Pro units to manufacture each month, and how many units to ship to the distribution centers each month in order to...

Question: Forecasting with the Parsimonious Method and Estimating Share Value Using the DCF Model Following are the income statement and balance sheet for Cisco Systems for the year ended July 30,...

Your goal is to set up a Texas statewide distribution network. Your company, Bearkat Inc., has recently expanded their operations and has decided to move from a single warehouse setup to a...

How to Quickly Buy X Twitter Accounts for Immediate Traffic Increase Top 4 sites to B-u-y Twitter Accounts Tone: Professional, actionable & user-friendly Target audience: Individuals, small...

(a) Find the moment estimator A of A. (b) Show that A is a biased estimator of A for a finite sample of size n, but becomes unbiased as # -Do. [Hint: You should be able to find the exact distribution...

(a) Find the moment estimator A of A. (b) Show that A is a biased estimator of A for a finite sample of size n, but becomes unbiased as # -+ 00. Hint: You should be able to find the exact...

1. A player throws a fair die and simultaneously flips a fair coin. If the coin lands heads, then she wins twice, and if tails, then one-half of the value that appears on the die. Determine her...

INFERENCE STATISTICS NEED HELP FROM QUESTION F TO QUESTION I (c) Show that f (x L8) belongs to the regular l-paranieter exponential family by showing that the probability density function can be...

(a) How does the use of condition codes complicate the implementation of a superscalar processor that supports out-of-order execution? [4 marks] (b) A branch predictor with a high prediction accuracy...

Question: Check you are in charge of the design of both hardware and software for a new (but fairly conventional) workstation which will have its peripherals (for example a disc drive and a printer)...