Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Let x(Omega )=2pi sum_(k=-infty )^(infty ) delta (Omega -(pi )/(4)-2pi k) . Here delta is the continuous impulse function. (a) Use x[n]=(1)/(2pi )int_(2pi ) x(Omega
Let
x(\\\\Omega )=2\\\\pi \\\\sum_(k=-\\\\infty )^(\\\\infty ) \\\\delta (\\\\Omega -(\\\\pi )/(4)-2\\\\pi k). Here
\\\\delta is the continuous impulse function.\ (a) Use
x[n]=(1)/(2\\\\pi )\\\\int_(2\\\\pi ) x(\\\\Omega )e^(j\\\\Omega n)d\\\\Omega to find
x[n]. Show the steps.\ (b) Compute
\\\\sum_(n=-\\\\infty )^(\\\\infty ) |x[n]|^(2). Compute
(1)/(2\\\\pi )\\\\int_0^(2\\\\pi ) |x(\\\\Omega )|^(2)d\\\\Omega . What do you observe?
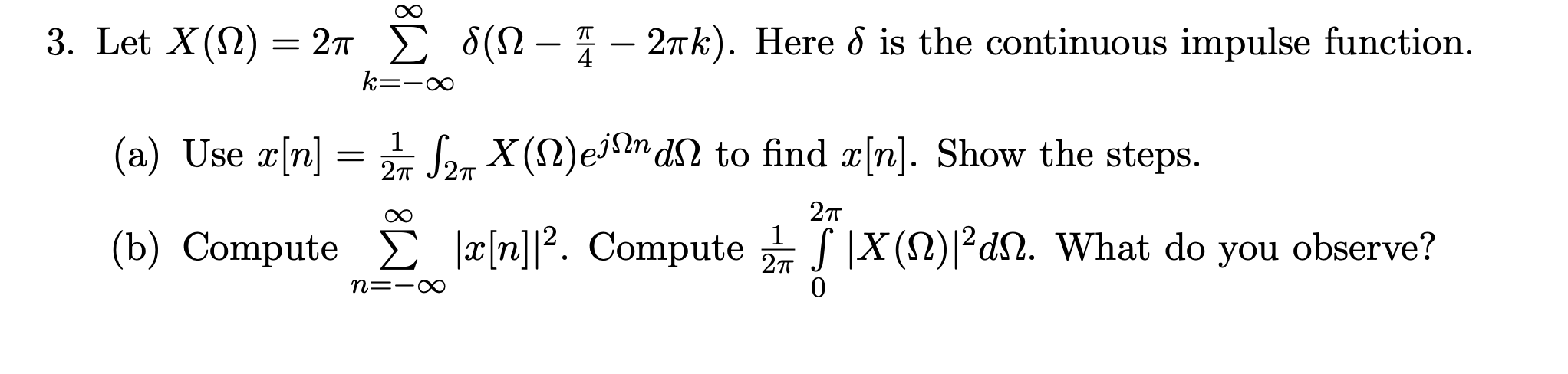
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


