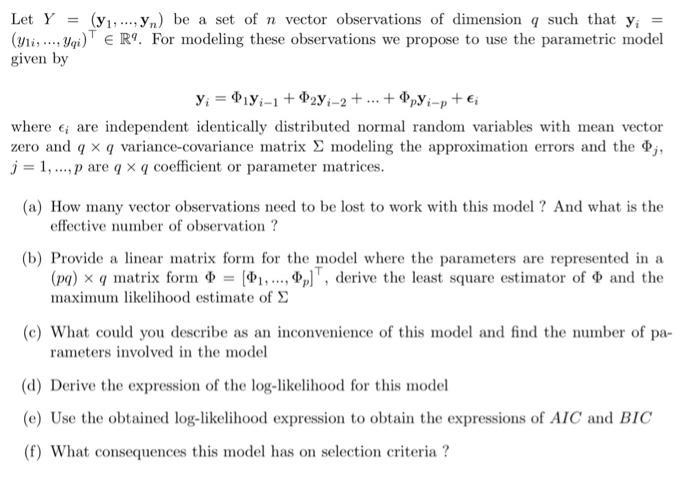
Let Y = (y Yn) be a set of n vector observations of dimension q such that y = R. For modeling these observations we propose to use the parametric model (yli qi) given by **** Y = Pyi-1 + P2Y-2 + ... + $pyi-p+ where are independent identically distributed normal random variables with mean vector zero and qx q variance-covariance matrix E modeling the approximation errors and the $, j= 1,..., p are qx q coefficient or parameter matrices. (a) How many vector observations need to be lost to work with this model? And what is the effective number of observation ? (b) Provide a linear matrix form for the model where the parameters are represented in a (pq) xq matrix form = [,], derive the least square estimator of and the maximum likelihood estimate of E (c) What could you describe as an inconvenience of this model and find the number of pa- rameters involved in the model (d) Derive the expression of the log-likelihood for this model (e) Use the obtained log-likelihood expression to obtain the expressions of AIC and BIC (f) What consequences this model has on selection criteria ?