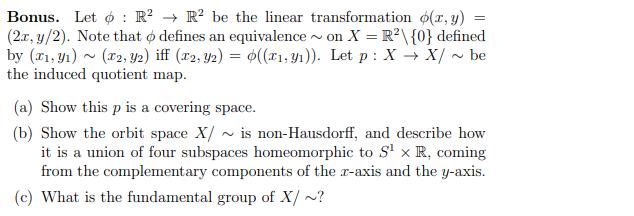
Leto: R R be the linear transformation (x, y) (2x, y/2). Note that o defines an equivalence on X = R\{0} defined by (x, y)~ (x2, y2) iff (2, 2) = ((1,91)). Let p: X X/ ~ be the induced quotient map. = (a) Show this p is a covering space. (b) Show the orbit space X/ is non-Hausdorff, and describe how it is a union of four subspaces homeomorphic to S x R. coming from the complementary components of the z-axis and the y-axis. (c) What is the fundamental group of X/~?