Question: Let's prove the theorem (MN)T = NT MT. Note: the following is a common technique for proving matrix identities. (a) Let M = (m)

Let

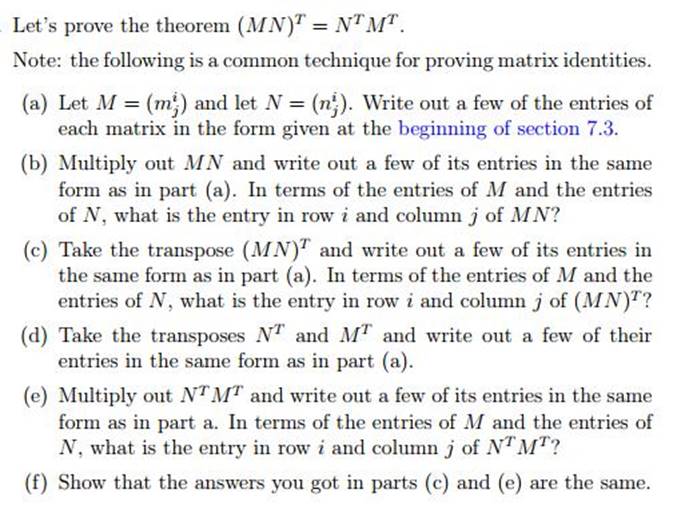
Let's prove the theorem (MN)T = NT MT. Note: the following is a common technique for proving matrix identities. (a) Let M = (m) and let N = (n). Write out a few of the entries of each matrix in the form given at the beginning of section 7.3. (b) Multiply out MN and write out a few of its entries in the same form as in part (a). In terms of the entries of M and the entries of N, what is the entry in row i and column j of MN? (c) Take the transpose (MN) and write out a few of its entries in the same form as in part (a). In terms of the entries of M and the entries of N, what is the entry in row i and column j of (MN)T? (d) Take the transposes NT and MT and write out a few of their entries in the same form as in part (a). (e) Multiply out NT MT and write out a few of its entries in the same form as in part a. In terms of the entries of M and the entries of N, what is the entry in row i and column j of NTMT? (f) Show that the answers you got in parts (c) and (e) are the same.

Step by Step Solution

★★★★★

3.45 Rating (158 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Accounting Questions!

The weighted average cost-flow assumption is a common technique used to value inventory and determine cost of goods sold. It falls between LIFO and FIFO as to the differential effect on inventory and...

Prove Theorem 3 as follows: Given an m x n matrix A, an element in Col A has the form Ax for some x in Rn. Let Ax and Aw represent any two vectors in Col A. a. Explain why the zero vector is in Col...

Prove Theorem 9.13. Theorem 9.13 An n n matrix A is similar to a diagonal matrix D if and only if A has n linearly independent eigenvectors. In this case, D = S1 AS, where the columns of S consist...

Define F on R3 by F(x) = (0, 0, cx3)x and let M be a compact three-dimensional manifold-with-boundary with MC {x: x3

(Multiple Choice) 1. Which of the following is not a fraud symptom related to journal entries? a. Unexplained adjustments to receivables, payables, revenues, or expenses. b. Journal entries that do...

Suppose that a company fails to make the proper adjusting journal entries associated with the following expenses and revenues: 1. Prepaid insurance that has expired 2. Interest that has accrued on...

Koko Chocolate Company makes dark chocolate and light chocolate. Both products require cocoa and sugar. The following planning information has been made available: Koko Chocolate does not expect...

Given the following functions F(s), find f (t). F(s) = s2 + 5s + 4 / (s + 2) (s + 4) (s + 6) F(s) = (s + 3) (s + 6) / s (s2 + 8s + 12)

When beats occur at a rate higher than about 20 per second, they are not heard individually but rather as a steady hum, called a combination tone. The player of a typical pipe organ can press a...

Sketch the graph of an example of a function f that satisfies all of the following conditions: lim f(x) = -2, lim f(x) = 1. f(0) = -1. %3D lim f(x) = 0. lim f(x) = -, lim f(x) = 3. lim f(x) = 4

The beach in Asbury Park is roughly 3 miles long. Each day there are 1000 people spread uniformly across the beach. On each end of the beach there is a hoagie shop. People travel up and down the...

Healthcare Providers and Nonbinary Patients The importance for adherence by those professionals to that code; Choose two principles from the code you chose to illustrate the values these...

Brewer Corporation. has the following information for August: The Decorating Department had 600 wreaths in process at the beginning of the month that were 80 percent complete s to material and 90...

All products and services move through the PRODUCT LIFE CYCLE What are the phases of the PRODUCT LIFE CYCLE? Chose a product or a service of a company in the sporting goods industry. Designate the...

Access to GPS tracking data from a smart phone to verify an alibi may be obtained from the service provider: at any time by accessing on-line databases through a formal request or subpoena only with...

Technician A says RAM contains calibration tables and look-up tables for the general make and model of the vehicle. Technician B says ROM contains permanent data that cannot be removed from memory....

You are asked to make a decision to invest in one of two established supermarkets. latest financial statements include the following details: Balance Sheet at December 31, 2018 Their Shoppers Fair...

Feller Company purchased a site for a limestone quarry for $100,000 on January 2, 2019. It estimate that the quarry will yield 400,000 tons of limestone. It estimates that its retirement obligation...

Discuss when each of the following types of businesses is likely to recognize revenues and expenses. a. A bank lends money for home mortgages. b. A travel agency books hotels, transportation, and...

Effective financial statement analysis requires an understanding of a firms economic characteristics. The relations between various financial statement items provide evidence of many of these...

Presented below is an excerpt from Starbucks Note 1, Summary of Significant Accounting Policies, in its September 28, 2008 Annual Report. a. The above passages indicate that Starbucks generates...

Recently, a group of adults who swim regularly for exercise were evaluated for depression. It turned out that these swimmers were less likely to be depressed than the general population. The...

Do cars get better gas mileage with premium instead of regular unleaded gasoline? It might be possible to test some engines in a laboratory, but we'd rather use real cars and real drivers in real...

A study published in the New England Journal of Medicine (Aug. 2001) suggests that it's dangerous to enter a hospital on a weekend. During a 10-year period, researchers tracked over 4 million...