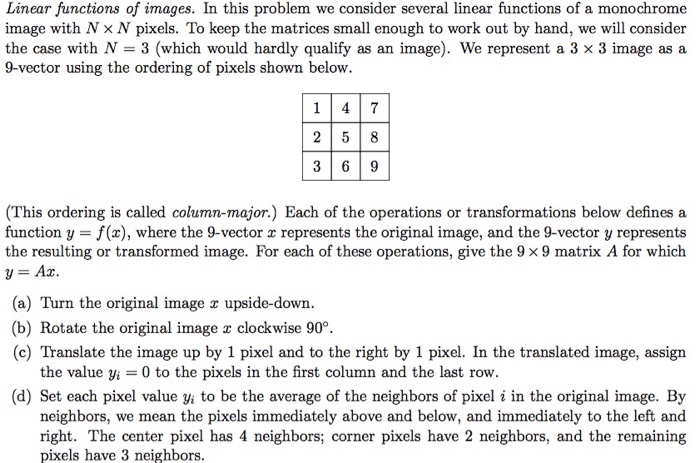
Linear functions of images. In this problem we consider several linear functions of a monochrome image with Nx N pixels. To keep the matrices small enough to work out by hand, we will consider the case with N = 3 (which would hardly qualify as an image). We represent a 3 x 3 image as a 9-vector using the ordering of pixels shown below. 147 258 369 (This ordering is called column-major.) Each of the operations or transformations below defines a function y = f(x), where the 9-vector a represents the original image, and the 9-vector y represents the resulting or transformed image. For each of these operations, give the 9 x 9 matrix A for which y = Ax. (a) Turn the original image x upside-down. (b) Rotate the original image x clockwise 90. (c) Translate the image up by 1 pixel and to the right by 1 pixel. In the translated image, assign the value y = 0 to the pixels in the first column and the last row. (d) Set each pixel value y; to be the average of the neighbors of pixel i in the original image. By neighbors, we mean the pixels immediately above and below, and immediately to the left and right. The center pixel has 4 neighbors; corner pixels have 2 neighbors, and the remaining pixels have 3 neighbors.