Question
a) The system consists of N >> 1 oscillator of total energy E in thermodynamic equilibrium. Determine the probability of finding the system in
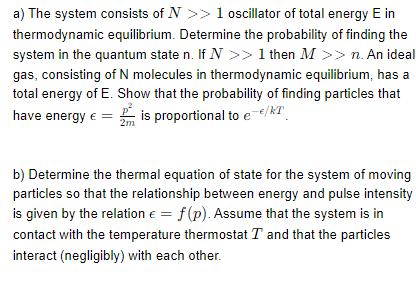
a) The system consists of N >> 1 oscillator of total energy E in thermodynamic equilibrium. Determine the probability of finding the system in the quantum state n. If N >> 1 then M >> n. An ideal gas, consisting of N molecules in thermodynamic equilibrium, has a total energy of E. Show that the probability of finding particles that have energy = is proportional to e-/kT 2m b) Determine the thermal equation of state for the system of moving particles so that the relationship between energy and pulse intensity is given by the relation = f(p). Assume that the system is in contact with the temperature thermostat T and that the particles interact (negligibly) with each other.
Step by Step Solution
3.35 Rating (158 Votes )
There are 3 Steps involved in it
Step: 1
Sol To calculate the probability that a is in the quantum state level n ...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get StartedRecommended Textbook for
Financial Accounting
Authors: LibbyShort
7th Edition
78111021, 978-0078111020
Students also viewed these General Management questions
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
View Answer in SolutionInn App



