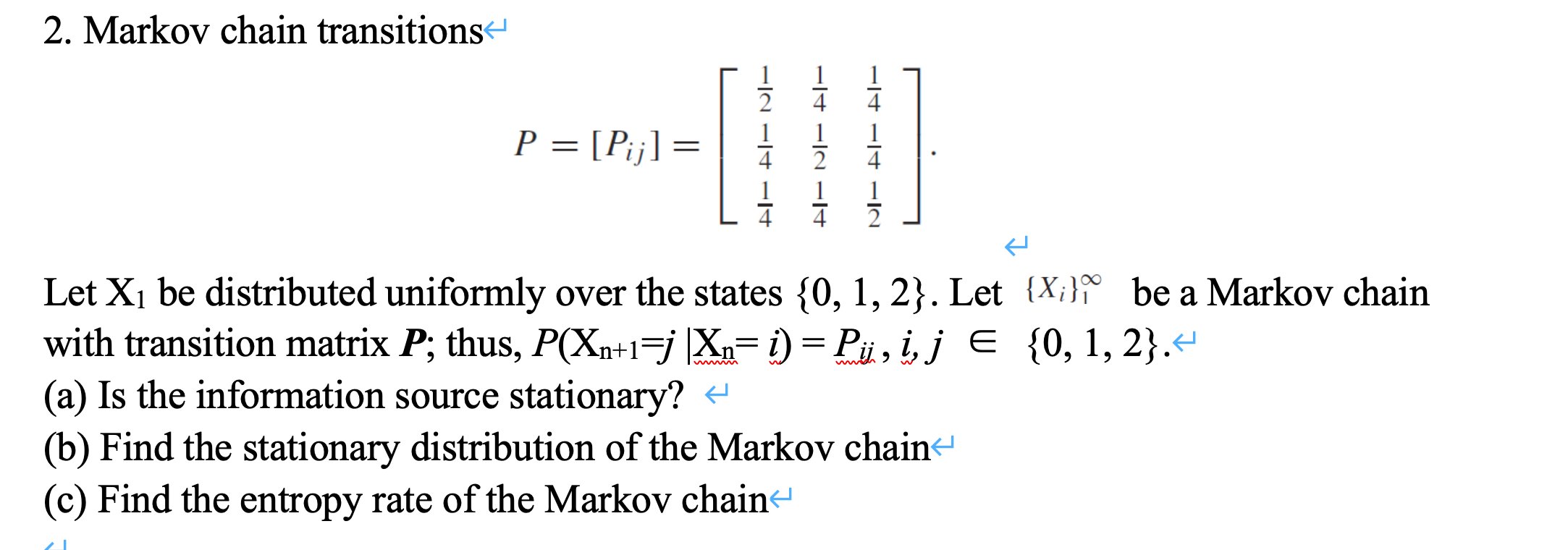
Markov chain transition

Which of the following is an advantage of multivariate correlational research over bivariate correlational research? O Bivariate correlational studies help address internal validity, whereas multivariate correlational studies do not. Multivariate correlational studies establish covariance, whereas bivariate correlational studies do not. O Some multivariate correlational studies help to address temporal precedence, whereas bivariate correlational studies do not. Multivariate correlational studies help to address external validity, whereas bivariate correlational studies do not.1. (a) Explain what is meant by the transition probability matrix of a homogeneous Markov chain. [5 marks] (b) Explain what is meant by the stationary distribution of a Markov chain? [5 marks] (c) A Markov chain has transition probability matrix, A, with entries Ouj; and stationary distribution . Write down an expression for the entries of the reverse Markov chain. [5 marks (d) Consider the following transition probability matrix of a homogo- neous Markov chain, with three states i,j and k (the TPM is in that order). If the stationary vector of the chain is (1/9, 2/9, 2/3), determine whether the Markov chain is reversible. 1 /0.2 0.2 0.6 0.1 0.6 0.3 4 \\0.1 0.1 0.8 [5 marks] (e) Let X1, X2, Xa be a sequence of random variables resulting from the above Markov chain. If X1 = i and Xs = j what is the probability that X2 = k? [5 marks]For each of the following transition matrices, determine whether the Markov chain with that transition matrix is regular: (1) Is the Markov chain whose transition matrix whose transition matrix is 1 0.2 0.8 0 0 regular? (Yes or No) (2) Is the Markov chain whose transition matrix whose transition matrix is 0 1 0.1 0.4 0.5 0 1 regular? (Yes or No) (3) Is the Markov chain whose transition matrix whose transition matrix is 0 0 0.8 0 0.2 0.8 0.2 0 regular? (Yes or No) (4) Is the Markov chain whose transition matrix whose transition matrix is 0 1 0.8 0 0.2 0 1 0 regular? (Yes or No)2. Markov chain transitions P = [P/j] = Al- AI- NI- Al- NI- AI- NI- AI- Al- Let X1 be distributed uniformly over the states {0, 1, 2}. Let (Xill be a Markov chain with transition matrix P; thus, P(Xn+1=j \\Xn= i) = Pu , i, j E {0, 1, 2}. (a) Is the information source stationary? ~ (b) Find the stationary distribution of the Markov chain (c) Find the entropy rate of the Markov chain