Question
Martina has a bag with 8 balls numbered 1 through 8. She is playing a game of chance. This game is this: Martina chooses one
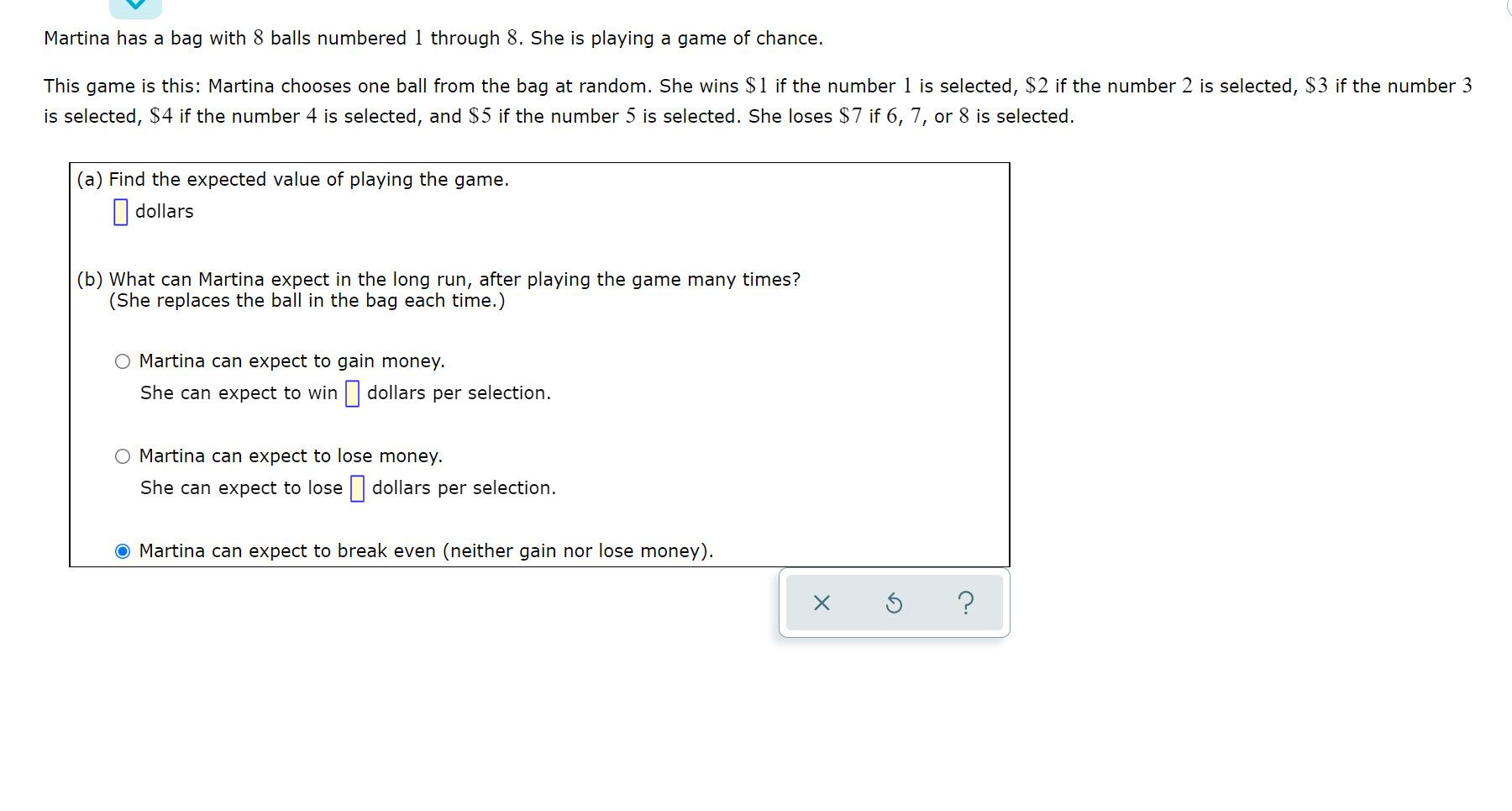
Martina has a bag with 8 balls numbered 1 through 8. She is playing a game of chance.
This game is this: Martina chooses one ball from the bag at random. She wins $1 if the number 1 is selected,$2 if the number 2 is selected, $3 if the number 3 is selected, $4 if the number 4 is selected, and $5 if the number 5 is selected. She loses $7 if 6, 7, or 8 is selected.
(a) Find the expected value of playing the game? __ dollars (b) What can Martina expect in the long run, after playing the game many times? (She replaces the ball in the bag each time.)
__Martina can expect to gain money. She can except to win __ dollars per selection
__Martina can expect to lose money. She can except to lose __ dollars per selection
__Martina can expect to break even (neither gain nor lose money).
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


