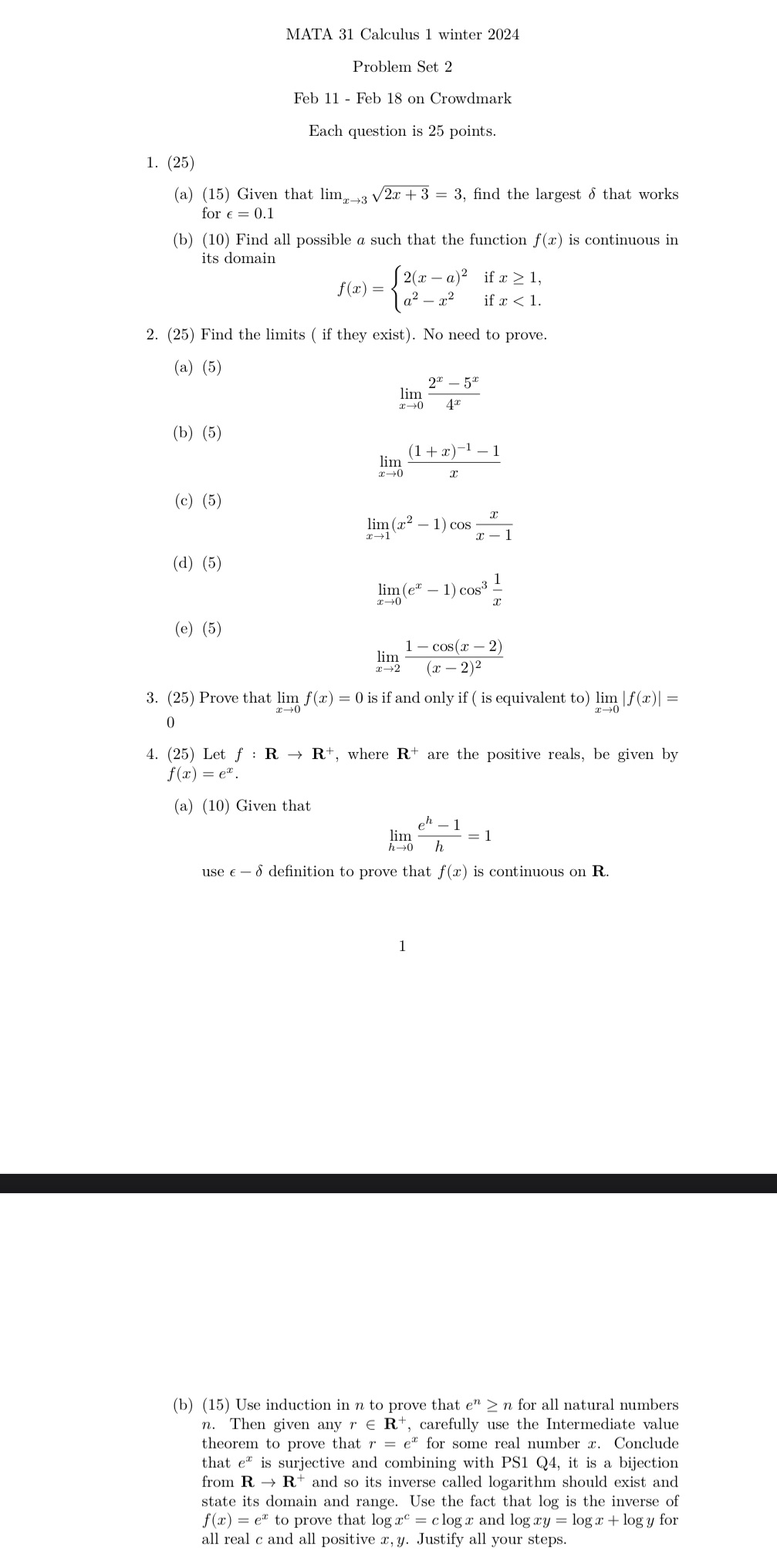
MATA 31 Calculus 1 winter 2024 Problem Set 2 Feb 11 Feb 18 on Crowdmark Each question is 25 points. 1. (25) (a) (15) Given that lim 3 2x + 3 for = 0.1 = 3, find the largest & that works (b) (10) Find all possible a such that the function f(x) is continuous in its domain 2(x-a)2 if x 1, f(x) = la. - x if x < 1. 2. (25) Find the limits (if they exist). No need to prove. (a) (5) (b) (5) lim 0+x 2-5 lim x-0 4 (1+x)-1-1 x (c) (5) x lim (x- 1) cos x-1 x 1 (d) (5) (e) (5) lim (e- x-0 1) cos 1 X 1 lim x-2 cos(x2) (x-2) 3. (25) Prove that lim f(x) = 0 is if and only if ( is equivalent to) lim |f(x)| = 0 x->0 x->0 = 4. (25) Let f RR+, where R+ are the positive reals, be given by f(x) = ex. (a) (10) Given that lim eh - 1 = 1 h0 h use - definition to prove that f(x) is continuous on R. 1 (b) (15) Use induction in n to prove that e" >n for all natural numbers n. Then given any r R, carefully use the Intermediate value. theorem to prove that r = e for some real number x. Conclude that is surjective and combining with PS1 Q4, it is a bijection from R R and so its inverse called logarithm should exist and state its domain and range. Use the fact that log is the inverse of f(x)=e to prove that log rc=clog x and logxy = log x + log y for all real c and all positive x, y. Justify all your steps.