Matlab (Linear algebra)

function C = cofactor(A, i, j) % cofactor Matrix of cofactors. % % C = cofactor(A) returns the matrix of cofactors of A. % If A is invertible, then inv(A) = C' / det(A). % % C = cofactor(A, i, j) returns the cofactor of % row i, column j of A. if nargin == 3 % Remove row i and column j to produce the minor. M = A; M(i,:) = []; M(:,j) = []; C = (-1)^(i+j) * det(M); else [n,n] = size(A); for i = 1:n for j = 1:n C(i,j) = cofactor(A, i, j); end end end 

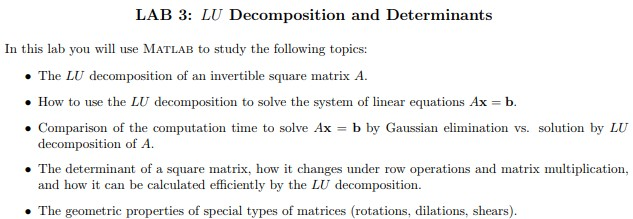
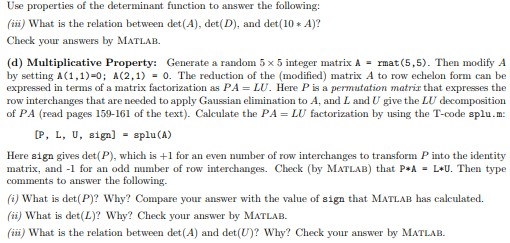
function [P, L, U, sign] = splu(A) % splu Square PA=LU factorization *with row exchanges*. % % [P, L, U] = splu(A), for a square, invertible matrix A, % uses Gaussian elimination to compute a permutation % matrix P, a lower triangular matrix L and % an upper triangular matrix U so that P*A = L*U. % P, L and U are the same size as A. % sign = det(P); it is 1 or -1. % % See also slu, lu, rref, partic, nulbasis, colbasis. [m, n] = size(A); if m ~= n error('Matrix must be square.') end P = eye(n, n); L = eye(n, n); U = zeros(n, n); tol = sqrt(eps); sign = 1; for k = 1:n if abs(A(k, k)) = tol break end if r == n if nargout == 4 sign = 0; return else disp('A is singular within tolerance.') error(['No pivot in column ' int2str(k) '.']) end end end A([r k], 1:n) = A([k r], 1:n); if k > 1, L([r k], 1:k-1) = L([k r], 1:k-1); end P([r k], 1:n) = P([k r], 1:n); sign = -sign; end for i = k+1:n L(i, k) = A(i, k) / A(k, k); for j = k+1:n A(i, j) = A(i, j) - L(i, k)*A(k, j); end end for j = k:n U(k, j) = A(k, j) * (abs(A(k, j)) >= tol); end end if nargout   LAB 3: LU Decomposition and Determinants In this lab you wil use MATLAB to study the following topics: The LU decomposition of an invertible square matrix A. . How to use the LU decomposition to solve the system of linear equations Ax b. Comparison of the computation time to solve Ax - b by Gaussian elimination vs. solution by LU decomposition of A. . The determinant of a square matrix, how it changes under row operations and matrix multiplication, and how it can be calculated efficiently by the LU decomposition . The geometric properties of special types of matrices (rotations, dilations, shears)