MatLab please using recursion/ one function only. Thank you!

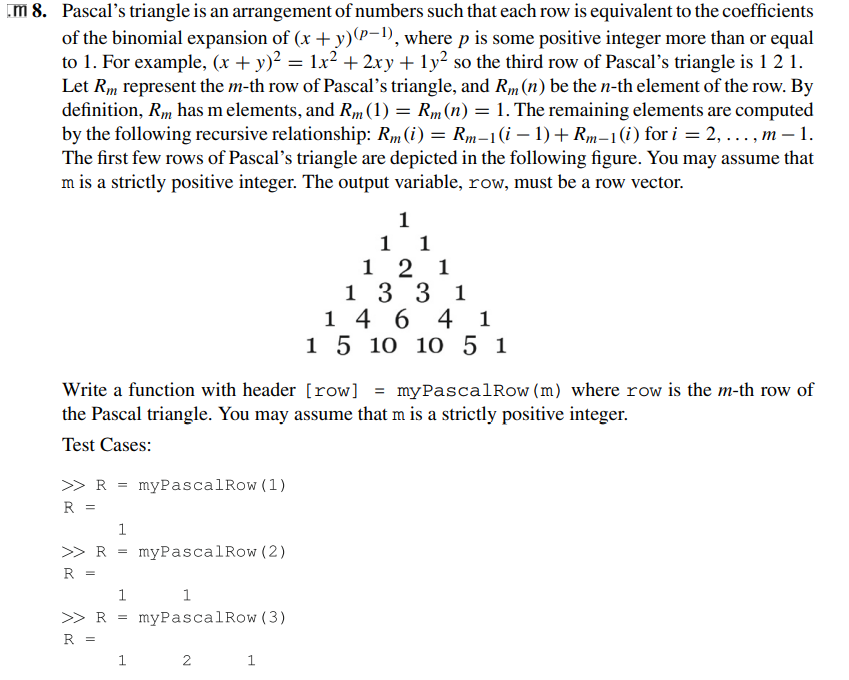
Pascal's triangle is an arrangement of numbers such that each row is equivalent to the coefficients of the binomial expansion of (x+y)(p1), where p is some positive integer more than or equal to 1 . For example, (x+y)2=1x2+2xy+1y2 so the third row of Pascal's triangle is 121 . Let Rm represent the m-th row of Pascal's triangle, and Rm(n) be the n-th element of the row. By definition, Rm has m elements, and Rm(1)=Rm(n)=1. The remaining elements are computed by the following recursive relationship: Rm(i)=Rm1(i1)+Rm1(i) for i=2,,m1. The first few rows of Pascal's triangle are depicted in the following figure. You may assume that m is a strictly positive integer. The output variable, row, must be a row vector. \[ \begin{array}{cccccc} \multicolumn{6}{c}{1} \\ & & \\ & 1 & 2 & 1 \\ & 1 & 3 & 3 & 1 \\ 1 & 4 & 6 & 4 & 1 \\ 1 & 5 & 10 & 10 & 5 & 1 \end{array} \] Write a function with header [ row ]= myPascalRow (m) where row is the m-th row of the Pascal triangle. You may assume that m is a strictly positive integer. Test Cases