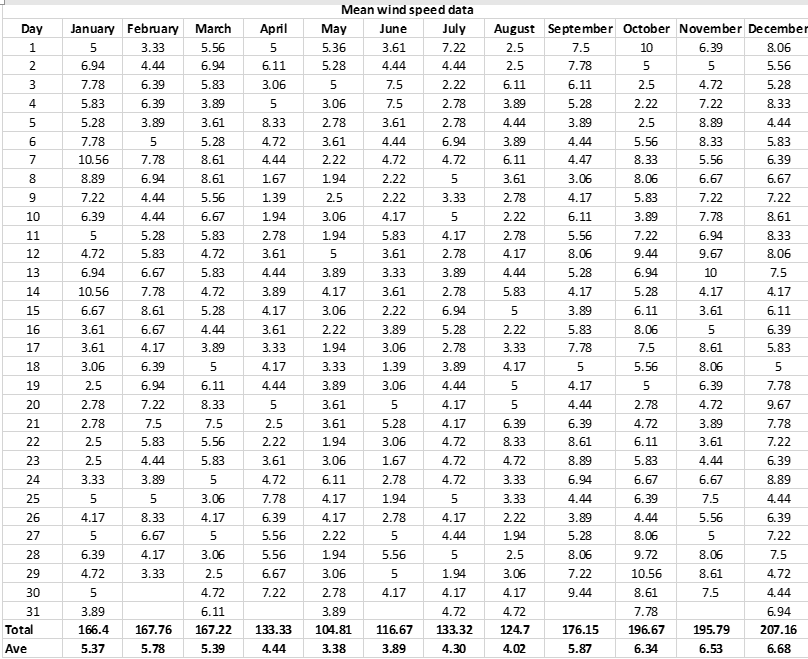
MAY YOU USE THE GIVEN EQUATIONS AT THE TOP TO MAKE TABLES (i) Wind speed data transformed into frequency distribution format (ii)Measured Wind speed Data arranged in frequency and cumulative distribution format of equal width of 1 m/s (iii)Monthly mean wind speeds and standard deviations for time-series and frequency formats

WITH WIND SPEED DATA GIVEN AND SHOW HOW TO CALCULATE THE STANDARD GAMMA FUNCTION FROM THE DATA

THOSE TABLES ARE FOR GUIDELINE. MAY YOU AT LEAST SHOW A LITTLE BIT OF WORKING TO SHOW AT LEAST HOW SOME VALUES ARE ACQUIRED FOR THE TABLE.

May Day 1 2 3 April 5 6.11 3.06 4 5 5 8.33 6 7 8 4.72 4.44 1.67 9 1.39 10 August 2.5 2.5 6.11 3.89 4.44 3.89 6.11 3.61 2.78 2.22 2.78 4.17 4.44 5.83 5 2.22 3.33 4.17 5 11 12 January February March 5 3.33 5.56 6.94 4.44 6.94 7.78 6.39 5.83 5.83 6.39 3.89 5.28 3.89 3.61 7.78 5 5.28 10.56 7.78 8.61 8.89 6.94 8.61 7.22 4.44 5.56 6.39 4.44 6.67 5 5.28 5.83 4.72 5.83 4.72 6.94 6.67 5.83 10.56 7.78 4.72 6.67 8.61 5.28 3.61 6.67 4.44 3.61 4.17 3.89 3.06 6.39 5 2.5 6.94 6.11 2.78 7.22 8.33 2.78 7.5 7.5 2.5 5.83 5.56 2.5 4.44 5.83 3.33 3.89 5 5 5 3.06 1.94 2.78 3.61 13 4.44 14 15 16 17 3.89 4.17 3.61 3.33 4.17 4.44 5 Mean wind speed data June July 5.36 3.61 7.22 5.28 4.44 4.44 5 7.5 2.22 3.06 7.5 2.78 2.78 3.61 2.78 3.61 4.44 6.94 2.22 4.72 4.72 1.94 2.22 5 2.5 2.22 3.33 3.06 4.17 5 1.94 5.83 4.17 5 3.61 2.78 3.89 3.33 3.89 4.17 3.61 2.78 3.06 2.22 6.94 2.22 3.89 5.28 1.94 3.06 2.78 3.33 1.39 3.89 3.89 3.06 4.44 3.61 5 4.17 3.61 5.28 4.17 1.94 3.06 4.72 3.06 1.67 4.72 6.11 2.78 4.72 4.17 1.94 5 4.17 2.78 4.17 2.22 5 4.44 1.94 5.56 3.06 5 1.94 2.78 4.17 4.17 3.89 4.72 104.81 116.67 133.32 3.38 3.89 4.30 September October November December 7.5 10 6.39 8.06 7.78 5 5 5.56 6.11 2.5 4.72 5.28 5.28 2.22 7.22 8.33 3.89 2.5 8.89 4.44 4.44 5.56 8.33 5.83 4.47 8.33 5.56 6.39 3.06 8.06 6.67 6.67 4.17 5.83 7.22 7.22 6.11 3.89 7.78 8.61 5.56 7.22 6.94 8.33 8.06 9.44 9.67 8.06 5.28 6.94 10 7.5 4.17 5.28 4.17 4.17 3.89 6.11 3.61 6.11 5.83 8.06 5 6.39 7.78 7.5 8.61 5.83 5 5.56 8.06 5 4.17 5 6.39 7.78 4.44 2.78 4.72 9.67 6.39 4.72 3.89 7.78 8.61 6.11 3.61 7.22 8.89 5.83 4.44 6.39 6.94 6.67 6.67 8.89 4.44 6.39 7.5 4.44 3.89 4.44 5.56 6.39 5.28 8.06 5 7.22 8.06 9.72 8.06 7.5 7.22 10.56 8.61 4.72 9.44 8.61 7.5 4.44 7.78 6.94 176.15 196.67 195.79 207.16 5.87 6.34 6.53 6.68 5 18 19 20 5 21 2.5 22 23 24 2.22 3.61 4.72 7.78 6.39 8.33 4.72 3.33 3.33 2.22 88 25 8.33 26 27 4.17 5 4.17 5 5 UT UT 6.67 4.17 1.94 2.5 28 6.39 5.56 5.56 6.67 7.22 6.39 un 3.33 4.72 5 29 30 31 Total Ave 3.06 2.5 4.72 6.11 167.22 5.39 3.89 166.4 5.37 3.06 4.17 4.72 124.7 4.02 167.76 5.78 133.33 4.44 Table 2: Wind speed data transformed into frequency distribution format 0.1 1-2 2.3 3.44 4.5 5.6 6.7 7-8 8.9 9.10 10-11 The monthly mean wind speed Vm and the standard deviation of the time-series of measured hourly wind speed data are determined using the Eq. (1)) and Eq. (2), [12, 16, 22, 23) 0.191 column). The fourth column gives the frequency of occurrence of each speed class (fi). The fifth column presents the probability of the measured wind speed based on the Eq. (3)) as given [12, 19): f(vi) (3) (3 , f, The mean wind speed and its standard deviation are calculated using the following equations, respectively, as given in, -N Vm ) 1 (1) 0.001 0.000 N21 Bins Period! January February March April May Jung July August September October November December Whole Year 0.0000 /2 0.315 0.401 0.309 0.401 0.154 0.359 0.071 0.267 0.083 0.308 0.091 0.314 0.113 0.362 0.192 0457 0.259 0.481 0.281 0.483 0389 0420 0.459 0.399 0.226 0.389 0.000 --[-21,0 mx]" - ) 0.199 0.068 0,015 0.002 0.000 0.067 0.016 0.003 0.000 0.288 0.148 0.043 0.007 0.001 0.313 0.189 0.091 0.042 0.016 0.006 0.003 0.310 0.174 0.08 0.031 0.011 0.002 0.001 0.302 0.171 0.075 0.030 0.01 0.004 0.001 0.283 0.132 0.067 0.028 0.011 0.003 0.001 0.226 0083 0.000 0.009 0.002 0.000 0.187 0.053 0.015 0.004 0.000 0.151 0.055 0021 0.007 0.001 0.000 0.129 0.044 0.014 0003 0.000 0.115 0.024 0.004 0000 0.225 0.101 0.039 0.014 0.005 0.001 0.000 IN (2) N-12=(-vm)? Vm2 V (4) N f; N 1/2 Where, Vm, mean wind speed, m/s; o, standard deviation of the observed data, m/s; V;, hourly wind speed, m/s; N, number of measured hourly wind speed data. 0.000 0.000 -[n- 27-16:40 .) ., , - 5 (5) N1 i 1 2 3 4 5 6 7 8 9 10 11 Table 3: Measured Wind speed Data arranged in frequency and cumulative distribution format of equal width of 1 m/s V Vi fi f(vi) 0-1 0.686 11866 0.226 1-2 1.471 20430 0.389 2-3 2.440 11809 0.225 3-4 3.427 5303 0.101 4-5 4.421 2071 0.039 5-6 5.416 735 0.014 6-7 6.409 243 0.005 7-8 7.393 72 0.001 8-9 8.491 32 0.000 9-10 9.332 6 0.000 10-11 10.467 1 0.000 N N fi= N = 52560 f () f(vi) = 1000 i=1 i=1 f(V) (v 0.226 0..614 0.839 0.940 0.979 0.993 0.998 0.999 1.000 1.000 1.000 Table 5: Monthly mean wind speeds and standard deviations for time-series and frequency formats Time-Series format Frequency format Relative error Period Vm Ots V Of EV EO January 1.585 0.920 1.585 0.881 0.000% 4.170% February 1.588 0.930 1.588 0.893 0.000% 4.078% March 2.093 1.057 2.093 1.022 0.000% 3.392% April 2.711 1.385 2.711 1.357 0.000% 2.038% May 2.511 1.256 2.511 1.225 0.000% 2.474% June 2.492 1.296 2.192 1.266 0.000% 2.302% July 2.333 1.287 2.333 1.258 0.000% 2.324% August 1.840 1.017 1.840 0.981 0.000% 3.559% September 1.603 0.884 1.603 0.843 0.000% 4.543% October 1.583 0.949 1.583 0.913 0.000% 3.857% November 1.395 0.870 1.395 0.832 0.000% 4.379% December 1.237 0.733 1.237 0.688 0.000% 6.134% Whole Year 1.915 1.168 1.915 1.136 0.000% 2.704% May Day 1 2 3 April 5 6.11 3.06 4 5 5 8.33 6 7 8 4.72 4.44 1.67 9 1.39 10 August 2.5 2.5 6.11 3.89 4.44 3.89 6.11 3.61 2.78 2.22 2.78 4.17 4.44 5.83 5 2.22 3.33 4.17 5 11 12 January February March 5 3.33 5.56 6.94 4.44 6.94 7.78 6.39 5.83 5.83 6.39 3.89 5.28 3.89 3.61 7.78 5 5.28 10.56 7.78 8.61 8.89 6.94 8.61 7.22 4.44 5.56 6.39 4.44 6.67 5 5.28 5.83 4.72 5.83 4.72 6.94 6.67 5.83 10.56 7.78 4.72 6.67 8.61 5.28 3.61 6.67 4.44 3.61 4.17 3.89 3.06 6.39 5 2.5 6.94 6.11 2.78 7.22 8.33 2.78 7.5 7.5 2.5 5.83 5.56 2.5 4.44 5.83 3.33 3.89 5 5 5 3.06 1.94 2.78 3.61 13 4.44 14 15 16 17 3.89 4.17 3.61 3.33 4.17 4.44 5 Mean wind speed data June July 5.36 3.61 7.22 5.28 4.44 4.44 5 7.5 2.22 3.06 7.5 2.78 2.78 3.61 2.78 3.61 4.44 6.94 2.22 4.72 4.72 1.94 2.22 5 2.5 2.22 3.33 3.06 4.17 5 1.94 5.83 4.17 5 3.61 2.78 3.89 3.33 3.89 4.17 3.61 2.78 3.06 2.22 6.94 2.22 3.89 5.28 1.94 3.06 2.78 3.33 1.39 3.89 3.89 3.06 4.44 3.61 5 4.17 3.61 5.28 4.17 1.94 3.06 4.72 3.06 1.67 4.72 6.11 2.78 4.72 4.17 1.94 5 4.17 2.78 4.17 2.22 5 4.44 1.94 5.56 3.06 5 1.94 2.78 4.17 4.17 3.89 4.72 104.81 116.67 133.32 3.38 3.89 4.30 September October November December 7.5 10 6.39 8.06 7.78 5 5 5.56 6.11 2.5 4.72 5.28 5.28 2.22 7.22 8.33 3.89 2.5 8.89 4.44 4.44 5.56 8.33 5.83 4.47 8.33 5.56 6.39 3.06 8.06 6.67 6.67 4.17 5.83 7.22 7.22 6.11 3.89 7.78 8.61 5.56 7.22 6.94 8.33 8.06 9.44 9.67 8.06 5.28 6.94 10 7.5 4.17 5.28 4.17 4.17 3.89 6.11 3.61 6.11 5.83 8.06 5 6.39 7.78 7.5 8.61 5.83 5 5.56 8.06 5 4.17 5 6.39 7.78 4.44 2.78 4.72 9.67 6.39 4.72 3.89 7.78 8.61 6.11 3.61 7.22 8.89 5.83 4.44 6.39 6.94 6.67 6.67 8.89 4.44 6.39 7.5 4.44 3.89 4.44 5.56 6.39 5.28 8.06 5 7.22 8.06 9.72 8.06 7.5 7.22 10.56 8.61 4.72 9.44 8.61 7.5 4.44 7.78 6.94 176.15 196.67 195.79 207.16 5.87 6.34 6.53 6.68 5 18 19 20 5 21 2.5 22 23 24 2.22 3.61 4.72 7.78 6.39 8.33 4.72 3.33 3.33 2.22 88 25 8.33 26 27 4.17 5 4.17 5 5 UT UT 6.67 4.17 1.94 2.5 28 6.39 5.56 5.56 6.67 7.22 6.39 un 3.33 4.72 5 29 30 31 Total Ave 3.06 2.5 4.72 6.11 167.22 5.39 3.89 166.4 5.37 3.06 4.17 4.72 124.7 4.02 167.76 5.78 133.33 4.44 Table 2: Wind speed data transformed into frequency distribution format 0.1 1-2 2.3 3.44 4.5 5.6 6.7 7-8 8.9 9.10 10-11 The monthly mean wind speed Vm and the standard deviation of the time-series of measured hourly wind speed data are determined using the Eq. (1)) and Eq. (2), [12, 16, 22, 23) 0.191 column). The fourth column gives the frequency of occurrence of each speed class (fi). The fifth column presents the probability of the measured wind speed based on the Eq. (3)) as given [12, 19): f(vi) (3) (3 , f, The mean wind speed and its standard deviation are calculated using the following equations, respectively, as given in, -N Vm ) 1 (1) 0.001 0.000 N21 Bins Period! January February March April May Jung July August September October November December Whole Year 0.0000 /2 0.315 0.401 0.309 0.401 0.154 0.359 0.071 0.267 0.083 0.308 0.091 0.314 0.113 0.362 0.192 0457 0.259 0.481 0.281 0.483 0389 0420 0.459 0.399 0.226 0.389 0.000 --[-21,0 mx]" - ) 0.199 0.068 0,015 0.002 0.000 0.067 0.016 0.003 0.000 0.288 0.148 0.043 0.007 0.001 0.313 0.189 0.091 0.042 0.016 0.006 0.003 0.310 0.174 0.08 0.031 0.011 0.002 0.001 0.302 0.171 0.075 0.030 0.01 0.004 0.001 0.283 0.132 0.067 0.028 0.011 0.003 0.001 0.226 0083 0.000 0.009 0.002 0.000 0.187 0.053 0.015 0.004 0.000 0.151 0.055 0021 0.007 0.001 0.000 0.129 0.044 0.014 0003 0.000 0.115 0.024 0.004 0000 0.225 0.101 0.039 0.014 0.005 0.001 0.000 IN (2) N-12=(-vm)? Vm2 V (4) N f; N 1/2 Where, Vm, mean wind speed, m/s; o, standard deviation of the observed data, m/s; V;, hourly wind speed, m/s; N, number of measured hourly wind speed data. 0.000 0.000 -[n- 27-16:40 .) ., , - 5 (5) N1 i 1 2 3 4 5 6 7 8 9 10 11 Table 3: Measured Wind speed Data arranged in frequency and cumulative distribution format of equal width of 1 m/s V Vi fi f(vi) 0-1 0.686 11866 0.226 1-2 1.471 20430 0.389 2-3 2.440 11809 0.225 3-4 3.427 5303 0.101 4-5 4.421 2071 0.039 5-6 5.416 735 0.014 6-7 6.409 243 0.005 7-8 7.393 72 0.001 8-9 8.491 32 0.000 9-10 9.332 6 0.000 10-11 10.467 1 0.000 N N fi= N = 52560 f () f(vi) = 1000 i=1 i=1 f(V) (v 0.226 0..614 0.839 0.940 0.979 0.993 0.998 0.999 1.000 1.000 1.000 Table 5: Monthly mean wind speeds and standard deviations for time-series and frequency formats Time-Series format Frequency format Relative error Period Vm Ots V Of EV EO January 1.585 0.920 1.585 0.881 0.000% 4.170% February 1.588 0.930 1.588 0.893 0.000% 4.078% March 2.093 1.057 2.093 1.022 0.000% 3.392% April 2.711 1.385 2.711 1.357 0.000% 2.038% May 2.511 1.256 2.511 1.225 0.000% 2.474% June 2.492 1.296 2.192 1.266 0.000% 2.302% July 2.333 1.287 2.333 1.258 0.000% 2.324% August 1.840 1.017 1.840 0.981 0.000% 3.559% September 1.603 0.884 1.603 0.843 0.000% 4.543% October 1.583 0.949 1.583 0.913 0.000% 3.857% November 1.395 0.870 1.395 0.832 0.000% 4.379% December 1.237 0.733 1.237 0.688 0.000% 6.134% Whole Year 1.915 1.168 1.915 1.136 0.000% 2.704%