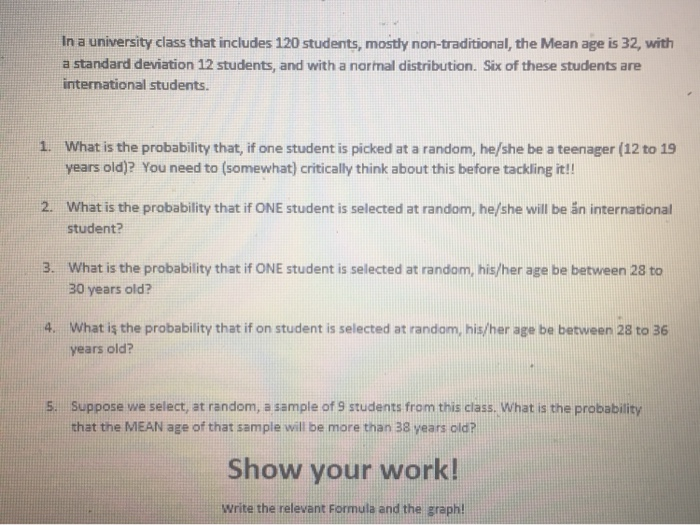


Medication STUDENT NAME MEDICATION hydromorphone REVIEW MODULE CHAPTER CATEGORY CLASS PURPOSE OF MEDICATION Expected Pharmacological Action Therapeutic Use Complications Medication Administration Contraindications/Precautions Nursing Interventions Interactions Client Education Evaluation of Medication EffectivenessIn a university class that includes 120 students, mostly non-traditional, the Mean age is 32, with a standard deviation 12 students, and with a normal distribution. Six of these students are international students. 1. What is the probability that, if one student is picked at a random, he/she be a teenager (12 to 19 years old)? You need to (somewhat] critically think about this before tackling it!! 2. What is the probability that if ONE student is selected at random, he/she will be an international student? 3. What is the probability that if ONE student is selected at random, his/her age be between 28 to 30 years old? 4. What is the probability that if on student is selected at random, his/her age be between 28 to 36 years old? 5. Suppose we select, at random, a sample of 9 students from this class. What is the probability that the MEAN age of that sample will be more than 38 years old? Show your work! Write the relevant Formula and the graph!Statistics Book: Sullivan: Fundamentals of Statistics. 16. The probability distribution of the random variable X represents the number of hits a baseball player obtained in a game for the 2012 baseball season. 0 2 3 4 5 P(x) 0.1663 0.3287 0.2815 0.1489 0.0383 0.0363 The probability distribution was used along with statistical software to simulate 25 repetitions of the experiment (25 games). The number of hits was recorded. Approximate the mean and standard deviation of the random variable X based on the simulation. The simulation was repeated by performing 50 repetitions of the experiment. Approximate the mean and standard deviation of the random variable. Compare your results to the theoretical mean and standard deviation. What property is being illustrated? Click the icon to view the data tables. Compute the theoretical mean of the random variable X for the given probability distribution. 1x -(Round to three decimal places as needed.) 1,4 73 Compute the theoretical standard deviation of the random variable X for the given probability distribution. a, = (Round to three decimal places as needed.) 1.23| Approximate the mean of the random variable X based on the simulation for 25 games. * = (Round to three decimal places as needed.) Approximate the standard deviation of the random variable X based on the simulation for 25 games, s=(Round to three decimal places as needed.) Approximate the mean of the random variable X based on the simulation for 50 games. x = (Round to three decimal places as needed.) Approximate the standard deviation of the random variable X based on the simulation for 50 games. s -(Round to three decimal places as needed.) What property is being illustrated? O Chebyshev's inequality The Law of Large Numbers O The empirical rule Data Table Page 101. We are going to test the \"Law of Large Numbers". A. To simulate the outcomes of rolling a die 10 times we will create a list of randomly generated integers. Enter: > L sample(1:6,10,replace=TRUE) B. Create a table and a barplot with this list. Enter: > tabletL) > barplot[table{L)} Question: What is the (empirical) probability of getting a \"five\" according to your table? C. Repeat part A and B above by simulating 100 rolls and based on these simulations calculate the (empirical) probability of getting a \"five". D. Repeat part A and E for 1,000 and 10,000 simulations. Report how far is the (empirical) probability of getting a \"five\" based on your 10,000 simulations from the theoretical probability (which equals 1/6)













