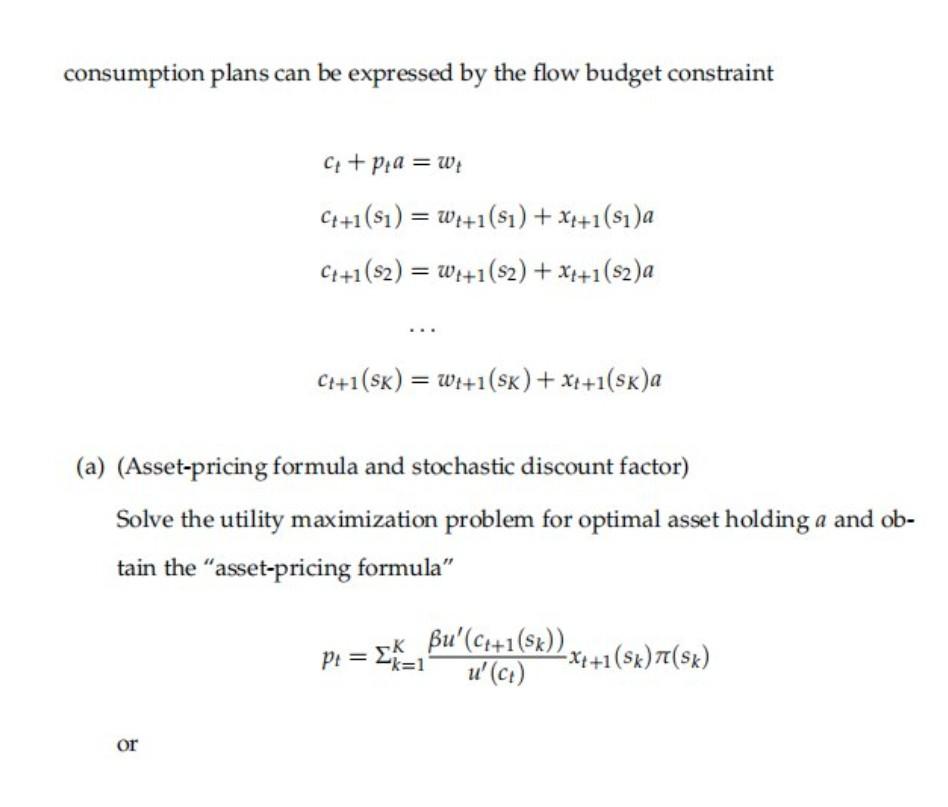
Microeconomic theory .book mwg.

1. (Asset Pricing) Consider a consumer who decides on a consumption plan of pe- riods t (today) and t +1 (the next period). While the contingency on period t is certain, what would happen in period t +1 is uncertain, which is expressed by the states S1,S2,..., Sk. Suppose that the consumer follows the axioms of the (subjective) expected utility and her utility function is given by u(c) = u(ct) + BEK=1u (C++1 (sk))(sk) = u(ct) + BE[u(C++1)], where c denotes the consumption plan, the (subjective) discount factor, a con- sumption in period t, C++1(sk) consumption in period t +1 under state Sk, and t(sk) the (subjective) probability of state Sk. Suppose that there is a financial market which trades an asset which is sold today at price p/ and bares the payoffs xx+1(sk) in period t +1. The consumer's income in period t +1 is also uncertain and is denoted by wr+1(Sk). Her period-t income is certain and is given by wr. Therefore, if she holds a units of the asset, her consumption plans can be expressed by the flow budget constraint C + Pa = w = C++1 (si) = W++1(si) + x++1(si)a C++1(S2) = wf+1(82) + x++1(82)a C++1(SK) = wi+1(SK) + x++1(sk)a Wf+ ( (a) (Asset-pricing formula and stochastic discount factor) Solve the utility maximization problem for optimal asset holding a and ob- tain the "asset-pricing formula" Bu'(c[+1 (Sk)) x2 +1 (5x)(sk) = =1 u' (c) -K or (a) (Asset-pricing formula and stochastic discount factor) Solve the utility maximization problem for optimal asset holding a and ob- tain the "asset-pricing formula" P: = k, Bu'(@+1(84)) k= -x++1 (sk) (sk) u' (c) or P = E [M4 +1X4+1], where my+1 (sk) := Bu'(C:+1(s)) is called "stochastic discount factor (SDF). u'(C) Intuitively, the SDF expresses the "value" of money for the individual in different states. (b) (Risk-free rate) Suppose that the asset is such that X1+1(sk) = Rp for any state. Derive the value of E[m++1] (c) (Risk-neutral asset pricing) When u(c) = = C, what is the asset-pricing formula? (d) (Subjective probability) 2 Continue to assume u(c) = c. If the asset is such that = ifs=sk Xp+1(s) = - 0 otherwise then what is the price of this asset? (e) (Risk correction for asset pricing) For notational simplicity, drop the subscript for the formula: p = Recall that the covariance Cov(m,x) satisfies E[mx] Cov(m,x) = E[mx] E[m]E[x]. Show that, from the asset-pricing formula, we obtain E[x] p= + Cov(m, x). RE This expression implies that the price of an asset depends on how the asset payoff and SDF covaries across states. 1. (Asset Pricing) Consider a consumer who decides on a consumption plan of pe- riods t (today) and t +1 (the next period). While the contingency on period t is certain, what would happen in period t +1 is uncertain, which is expressed by the states S1,S2,..., Sk. Suppose that the consumer follows the axioms of the (subjective) expected utility and her utility function is given by u(c) = u(ct) + BEK=1u (C++1 (sk))(sk) = u(ct) + BE[u(C++1)], where c denotes the consumption plan, the (subjective) discount factor, a con- sumption in period t, C++1(sk) consumption in period t +1 under state Sk, and t(sk) the (subjective) probability of state Sk. Suppose that there is a financial market which trades an asset which is sold today at price p/ and bares the payoffs xx+1(sk) in period t +1. The consumer's income in period t +1 is also uncertain and is denoted by wr+1(Sk). Her period-t income is certain and is given by wr. Therefore, if she holds a units of the asset, her consumption plans can be expressed by the flow budget constraint C + Pa = w = C++1 (si) = W++1(si) + x++1(si)a C++1(S2) = wf+1(82) + x++1(82)a C++1(SK) = wi+1(SK) + x++1(sk)a Wf+ ( (a) (Asset-pricing formula and stochastic discount factor) Solve the utility maximization problem for optimal asset holding a and ob- tain the "asset-pricing formula" Bu'(c[+1 (Sk)) x2 +1 (5x)(sk) = =1 u' (c) -K or (a) (Asset-pricing formula and stochastic discount factor) Solve the utility maximization problem for optimal asset holding a and ob- tain the "asset-pricing formula" P: = k, Bu'(@+1(84)) k= -x++1 (sk) (sk) u' (c) or P = E [M4 +1X4+1], where my+1 (sk) := Bu'(C:+1(s)) is called "stochastic discount factor (SDF). u'(C) Intuitively, the SDF expresses the "value" of money for the individual in different states. (b) (Risk-free rate) Suppose that the asset is such that X1+1(sk) = Rp for any state. Derive the value of E[m++1] (c) (Risk-neutral asset pricing) When u(c) = = C, what is the asset-pricing formula? (d) (Subjective probability) 2 Continue to assume u(c) = c. If the asset is such that = ifs=sk Xp+1(s) = - 0 otherwise then what is the price of this asset? (e) (Risk correction for asset pricing) For notational simplicity, drop the subscript for the formula: p = Recall that the covariance Cov(m,x) satisfies E[mx] Cov(m,x) = E[mx] E[m]E[x]. Show that, from the asset-pricing formula, we obtain E[x] p= + Cov(m, x). RE This expression implies that the price of an asset depends on how the asset payoff and SDF covaries across states