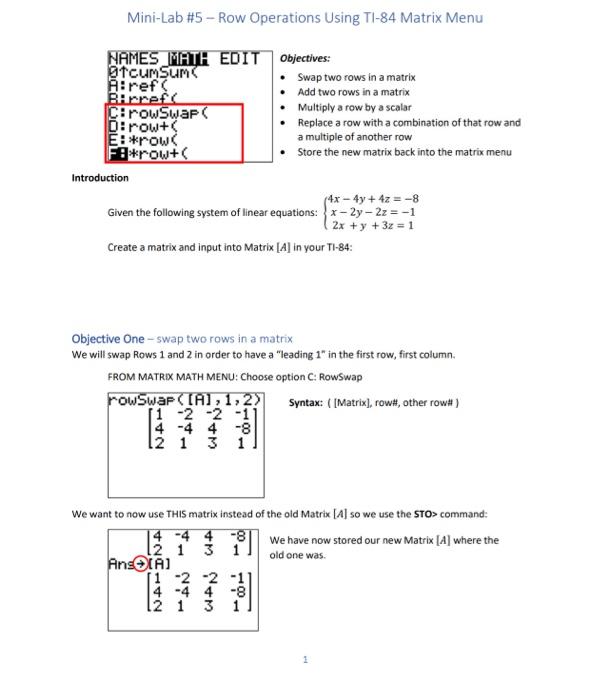
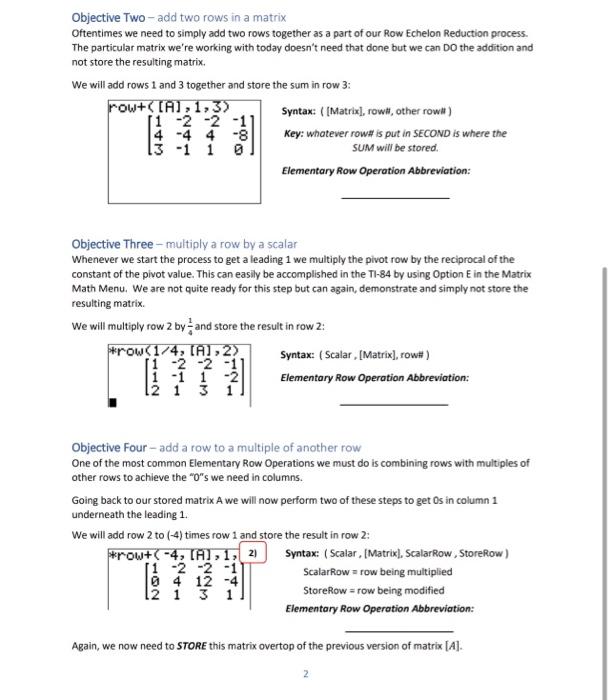
Mini-Lab #5 -Row Operations Using TI-84 Matrix Menu NAMES MATH EDIT Objectives: OtcumSum A:ref Birref( C:rowSwap D:row+ < E: *row F:*row+( Introduction Swap two rows in a matrix Add two rows in a matrix Multiply a row by a scalar Replace a row with a combination of that row and a multiple of another row Store the new matrix back into the matrix menu (4x-4y + 4z=-8 Given the following system of linear equations:x-2y-2z = -1 2x + y +3x = 1 Create a matrix and input into Matrix [A] in your TI-84: Objective One-swap two rows in a matrix We will swap Rows 1 and 2 in order to have a "leading 1" in the first row, first column. FROM MATRIX MATH MENU: Choose option C: RowSwap rowSwap ([A],1,2) [1 -2 -2 -11 4 -4 4-8 Ans[A] Syntax: ([Matrix], row#, other row# ) We want to now use THIS matrix instead of the old Matrix [A] so we use the STO> command: 4-44-8 12 13 1 We have now stored our new Matrix [4] where the old one was. 1 Objective Two-add two rows in a matrix Oftentimes we need to simply add two rows together as a part of our Row Echelon Reduction process. The particular matrix we're working with today doesn't need that done but we can DO the addition and not store the resulting matrix. We will add rows 1 and 3 together and store the sum in row 3: row+[A] 1,3) [1 -2 -2 -11 4 4 4 -8 13 -1 1 Objective Three-multiply a row by a scalar Whenever we start the process to get a leading 1 we multiply the pivot row by the reciprocal of the constant of the pivot value. This can easily be accomplished in the TI-84 by using Option E in the Matrix Math Menu. We are not quite ready for this step but can again, demonstrate and simply not store the resulting matrix. We will multiply row 2 by and store the result in row 2: *row(1/4, [A],2) [1 -2 -2 -1 1 1 1 2 1 3 Syntax: ([Matrix], row#, other rowl) Key: whatever row# is put in SECOND is where the SUM will be stored. Elementary Row Operation Abbreviation: Syntax: (Scalar, [Matrix], row#) Elementary Row Operation Abbreviation: Objective Four - add a row to a multiple of another row One of the most common Elementary Row Operations we must do is combining rows with multiples of other rows to achieve the "0"s we need in columns. Going back to our stored matrix A we will now perform two of these steps to get Os in column 1 underneath the leading 1. *row+(-4, [A], 1, 2) -2 -2 04 12 -4 12 13 1 We will add row 2 to (-4) times row 1 and store the result in row 2: Syntax: (Scalar, [Matrix), ScalarRow, StoreRow) ScalarRow= row being multiplied StoreRow= row being modified Elementary Row Operation Abbreviation: Again, we now need to STORE this matrix overtop of the previous version of matrix [A]. Now we will add row 3 to (-2) times row 1 and store the result in row 3: Next we need to pivot around the 4 in row 2, column 2. This means starting off with a scalar multiplication operation: Continue with the steps needed to transform our matrix to Reduced Row Echelon Form.