Question
Module 1 1. Question 1 True or false? Ifx x is a nonzero real number andn n is a positive integer, thenx^{-n} = -x^n x
Module 1
1.
Question 1
True or false? Ifxxis a nonzero real number andnnis a positive integer, thenx^{-n} = -x^nxn=xn.
1 point
True
False
2.
Question 2
Consider the exponential expression4^{3/2}43/2.
Which number is thebasein the expression?
1 point
44
3/23/2
22
33
3.
Question 3
Use the properties of exponents to find\dfrac{5^{11}}{(5^2)^3\cdot25}(52)325511.
If your answer is not a whole number, enter it as a decimal.
1 point
4.
Question 4
Use the properties of exponents to find\left(\dfrac{4^2\cdot 2^3}{8} ight)^{-1/2}(84223)1/2.
If your answer is not a whole number, enter it as a decimal.
1 point
5.
Question 5
Use the properties of exponents to find\left(\dfrac14 ight)^{-3/2}(41)3/2.
If your answer is not a whole number, enter it as a decimal.
1 point
6.
Question 6
Use the properties of exponents to find\dfrac{2\cdot 6^4}{3^3\cdot24}3324264.
If your answer is not a whole number, enter it as a decimal.
1 point
7.
Question 7
Simplify the expression\dfrac{\sqrt{16x^{-6}y^7}}{x^{-2}y^0}x2y016x6y7.
(Assume thatxxandyyare both nonzero real numbers)
1 point
4xy^{7/2}4xy7/2
\dfrac{4y^{7/2}}{x}x4y7/2
4x^{3/2}y^{7/2}4x3/2y7/2
4x^{3/2}4x3/2
8.
Question 8
Simplify the expression\dfrac{2a^{-4}b^{-2}}{4a^{-6}b^8}4a6b82a4b2.
(Assumeaaandbbare nonzero real numbers.)
1 point
\dfrac{a^2}{2b^{10}}2b10a2
\dfrac{b^6}{2a^{10}}2a10b6
\dfrac12 a^{2/3} b^{-1/4}21a2/3b1/4
\dfrac{1}{2a^2 b^{10}}2a2b101
9.
Question 9
Selectallof the following expressions that are equal to11.
1 point
1^010
0^000
10^{1}101
0^101
1^111
1^{10}110
10^{0}100
10.
Question 10
Letxxbe a real number. Supposemmandnnare nonzero integers.
Which of the following is equal tox^{m}xm/n?
Select all that apply.
1 point
\sqrt[n]{x^m}nxm
\left(\sqrt[n]{x} ight)^m(nx)m
Neither of these
Module 2
1.
Question 1
Solve forttin the exponential equation.
(-5)^t = -\dfrac{1}{125}(5)t=1251
Enter a number only for your answer. If your answer is not a whole number, write it as a decimal.
1 point
2.
Question 2
Findxxsuch that7^{3x} = \sqrt{7^x}73x=7x.
Enter a number only for your answer. If your answer is not a whole number, write it as a decimal.
1 point
3.
Question 3
Solve forssin the exponential equation.
6^{14-2s} = 6^{5s}6142s=65s
Enter a number only for your answer. If your answer is not a whole number, write it as a decimal.
1 point
4.
Question 4
Findvvsuch that
16^{v-1} = 2^{10v + 8}16v1=210v+8
Enter a number only for your answer. If your answer is not a whole number, write it as a decimal.
1 point
5.
Question 5
Solve forxx.
3^{2x + (1/4)} = \dfrac{1}{9^{-2 - (x/4)}}32x+(1/4)=92(x/4)1
Enter a number only for your answer. If your answer is not a whole number, write it as a decimal.
1 point
6.
Question 6
Solve foryy.
25^{2y + (1/2)} = \dfrac{5^{8y}}{25}252y+(1/2)=2558y
Enter a number only for your answer. If your answer is not a whole number, write it as a decimal.
1 point
7.
Question 7
There are approximately8.6 \times 10^{10}8.61010 neurons in the adult human brain.
Convert this number from scientific notation to standard notation.
Enter a number only (no units, no commas).
1 point
8.
Question 8
Theperihelionin a planet's orbit about the Sun is the point where the planet comes closest to the Sun.
At perihelion, the planet Venus is approximately67,700,000\text{ miles}67,700,000miles from the Sun.
Write distance in scientific notation.
1 point
67.7\times 10^{-6}\text{ miles}67.7106miles
6.77\times 10^7\text{ miles}6.77107miles
0.677\times 10^8\text{ miles}0.677108miles
6.77\times 10^{-7}\text{ miles}6.77107miles
67.7\times 10^6\text{ miles}67.7106miles
9.
Question 9
Theaphelionin a planet's orbit about the Sun is the point where the planet is farthest from the Sun.
At perihelion, the planet Earth is approximately9.14\times 10^7\text{ miles}9.14107miles from the Sun.
At aphelion, Earth is approximately9.45\times 10^7\text{ miles}9.45107miles from the Sun.
Find the difference between the Earth's distance from the Sun at perihelion and aphelion, using scientific notation.
1 point
3.1\times 10^6\text{ miles}3.1106miles
3.1\text{ miles}3.1miles
31\times 10^5\text{ miles}31105miles
0.31\times 10^7\text{ miles}0.31107miles
0.31\text{ miles}0.31miles
3.1 \times 10^7\text{ miles}3.1107miles
31\text{ miles}31miles
10.
Question 10
The mass of a single grain of sand is approximately6.75\times 10^{-4} \text{ grams}6.75104grams.
There are1.5\times 10^61.5106 grains of sand in an hour glass.
Find the total mass of sand in the hourglass, in grams.
answer using standard notation (not scientific notation).
Enter a number only (no units, no commas).
1 point
Module 3
1.
Question 1
Which of the expressions below are polynomials?
Select all that apply.
1 point
x^2 - x + \dfrac{2}{x}x2x+x2
2x + 32x+3
4x^3 - 7x + \sqrt{2}4x37x+2
\sqrt{5}x^5 - \sqrt{3}x^3 + x5x53x3+x
3x^2 + 2x^{3/2} + 13x2+2x3/2+1
2.
Question 2
Consider the polynomialp(x) = 9x^2 - 7x^3 + x^5 - 8x + 6p(x)=9x27x3+x58x+6.
What is the degree ofp(x)p(x)?
Enter a number only.
1 point
3.
Question 3
Letp(x)p(x) andq(x)q(x) be two polynomials.
True or false? The degree of the sump(x) + q(x)p(x)+q(x) is the sum of the degrees ofp(x)p(x) andq(x)q(x).
1 point
True
False
4.
Question 4
True or false?
A quadratic equationax^2 + bx + c = 0ax2+bx+c=0 (whereaa,bb, andccare real numbers) has exactly two different solutions.
1 point
True
False
5.
Question 5
Find the sum of the two polynomials:
(x^5 + x^3 - 3) + (x - 2x^3 + 1)(x5+x33)+(x2x3+1)
1 point
x^5 - x^3 + x - 2x5x3+x2
x^5 + 3x^3 - x - 4x5+3x3x4
None of these
2x^5 - x^3 - 22x5x32
x^6 - x^3 - 2x6x32
6.
Question 6
Find the product:
5x^3(x^2 - 3x + 2)5x3(x23x+2)
1 point
5x^6 - 15x^3 + 10x^35x615x3+10x3
5x^5 - 15x^4 + 10x^35x515x4+10x3
5x^6 - 3x + 25x63x+2
5x^5 - 3x + 25x53x+2
None of these
7.
Question 7
Determine the product of the binomials:
(12x - 1)(4 - x^2)(12x1)(4x2)
1 point
None of these
12x^3 - x^2 - 48x + 412x3x248x+4
-11x^2 + 48x - 411x2+48x4
-12x^3 - x^2 + 48x - 412x3x2+48x4
-12x^3 + x^2 + 48x - 412x3+x2+48x4
8.
Question 8
Rewrite the quadratic as a product of linear factors:
14x^2 + x - 314x2+x3
1 point
(7x - 3)(2x + 1)(7x3)(2x+1)
(14x + 1)(x -3)(14x+1)(x3)
(14x - 3)(x + 1)(14x3)(x+1)
None of these
(7x + 1)(2x -3)(7x+1)(2x3)
9.
Question 9
Find the factorization of the cubic polynomial:
3x^3 + 12x^2 - 15x3x3+12x215x
1 point
x(x - 1)(3x + 5)x(x1)(3x+5)
3x(x - 1)(x + 5)3x(x1)(x+5)
x(3x - 5)(x + 1)x(3x5)(x+1)
3x(x - 5)(x + 1)3x(x5)(x+1)
x(3x - 1)(x + 5)x(3x1)(x+5)
10.
Question 10
Solve the polynomial equation by factoring:
2x^3 - 16x^2 + 32x = 02x316x2+32x=0.
Selectallsolutions below.
1 point
x = -4x=4
x =0x=0
x = 16x=16
x = 4x=4
x = -16x=16
x = -2x=2
x = 2x=2
Module 4
1.
Question 1
Complete the sentence.
If a quadratic polynomial has a negative leading coefficient, then its vertex is . . .
1 point
a minimum.
sometimes a minimum and sometimes a maximum.
a maximum.
2.
Question 2
True or false?
The graph of a cubic polynomial always has anxx-intercept.
1 point
True
False
3.
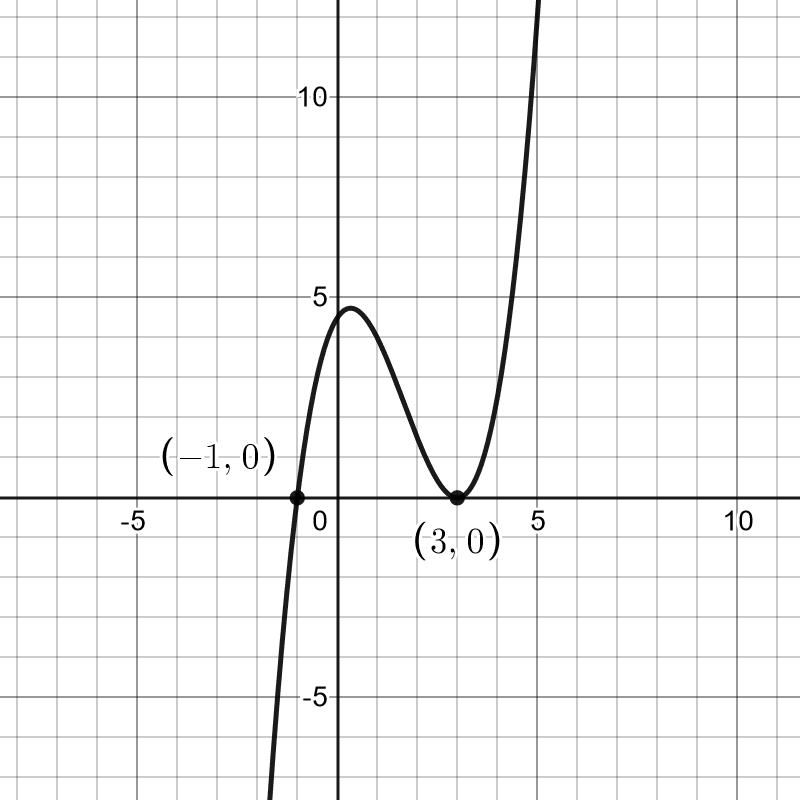
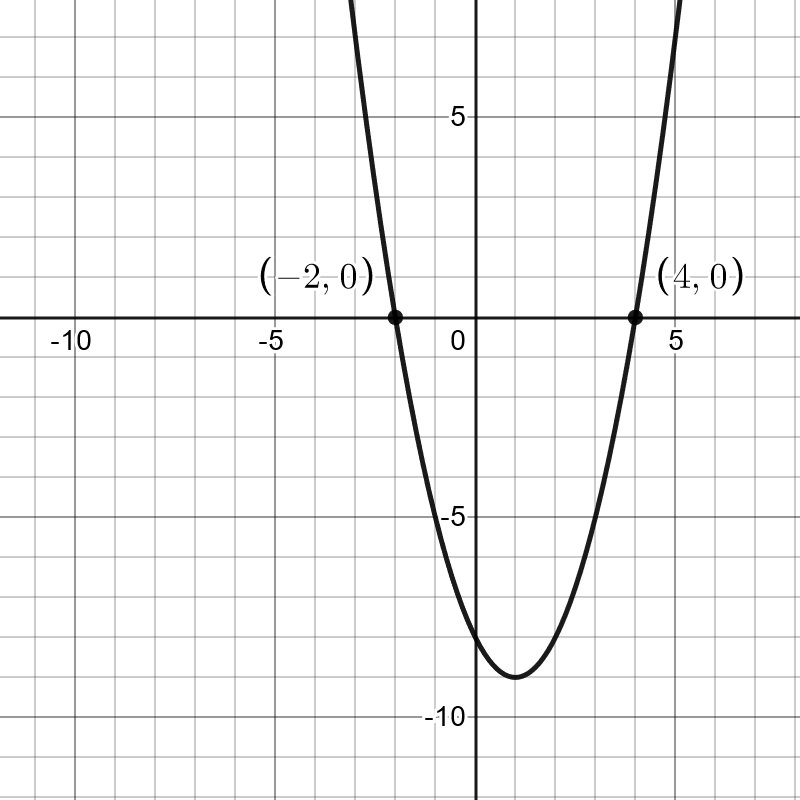
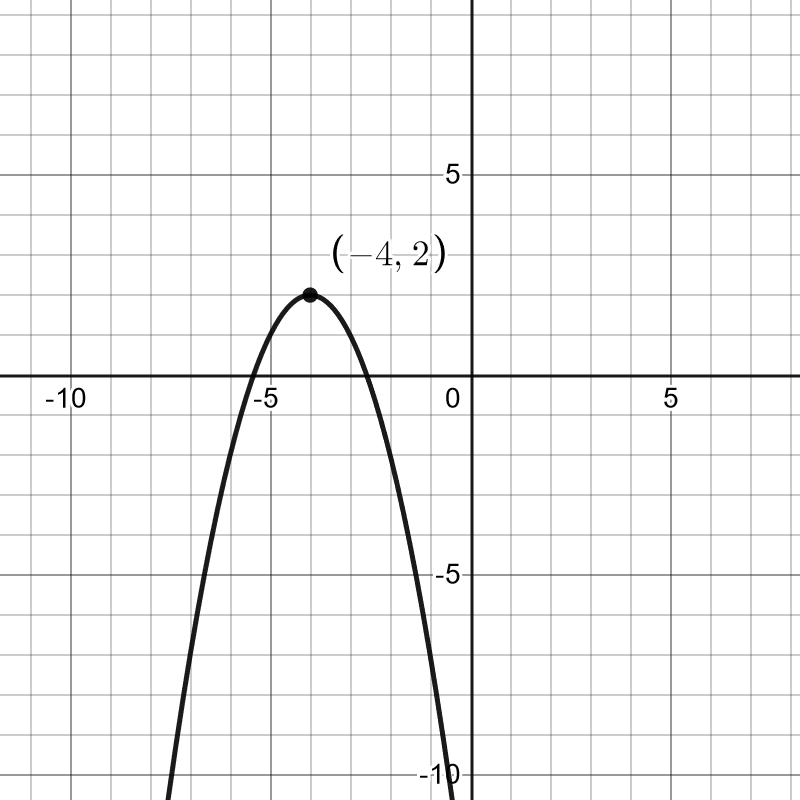
Question 3
A polynomial is graphed below.
Use the end behavior of the graph to determine the parity of the degree and the sign of the leading coefficient.
1 point
even degree; positive leading coefficient
even degree; negative leading coefficient
odd degree; positive leading coefficient
odd degree; negative leading coefficient
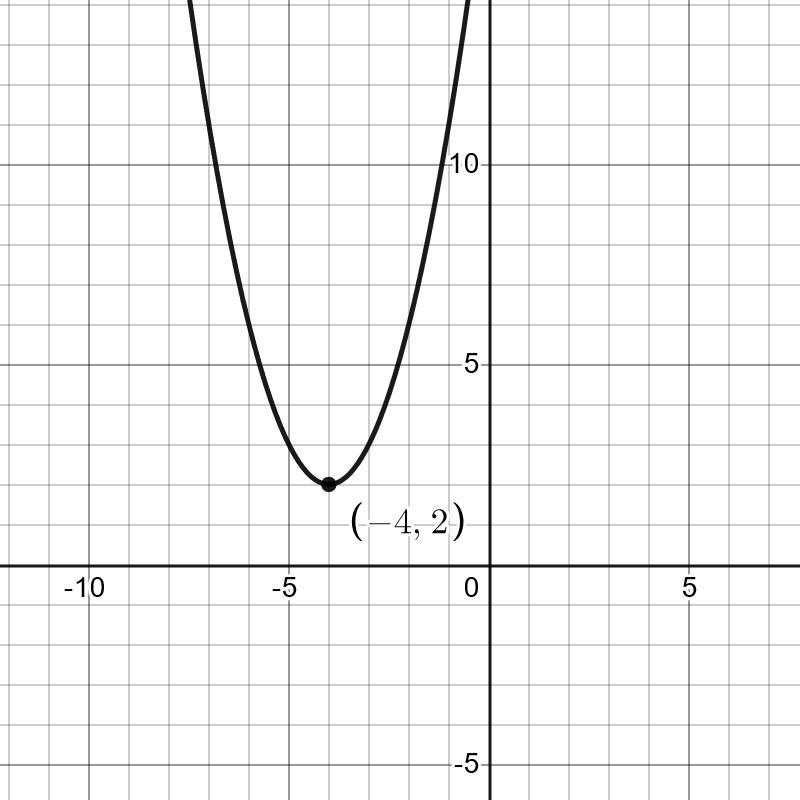
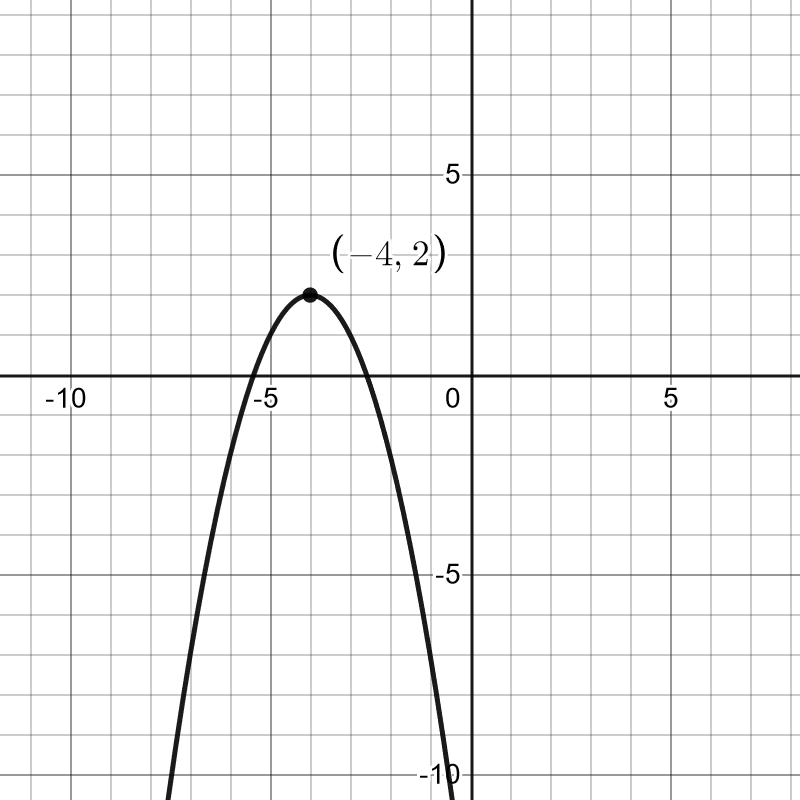
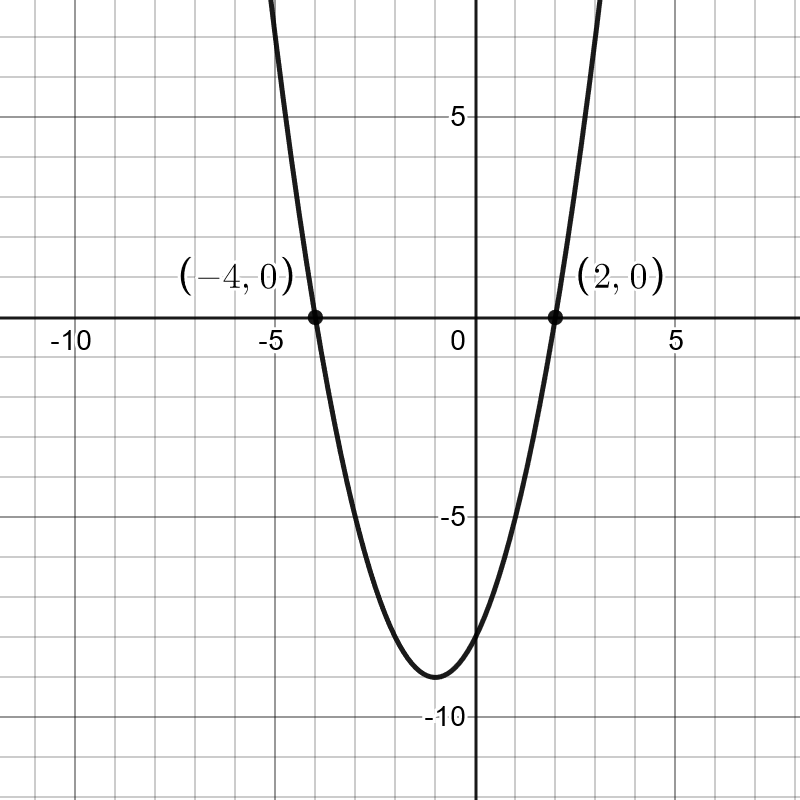
4.
Question 4
A polynomial is graphed below. Which of the polynomials from the list could correspond to the graph?

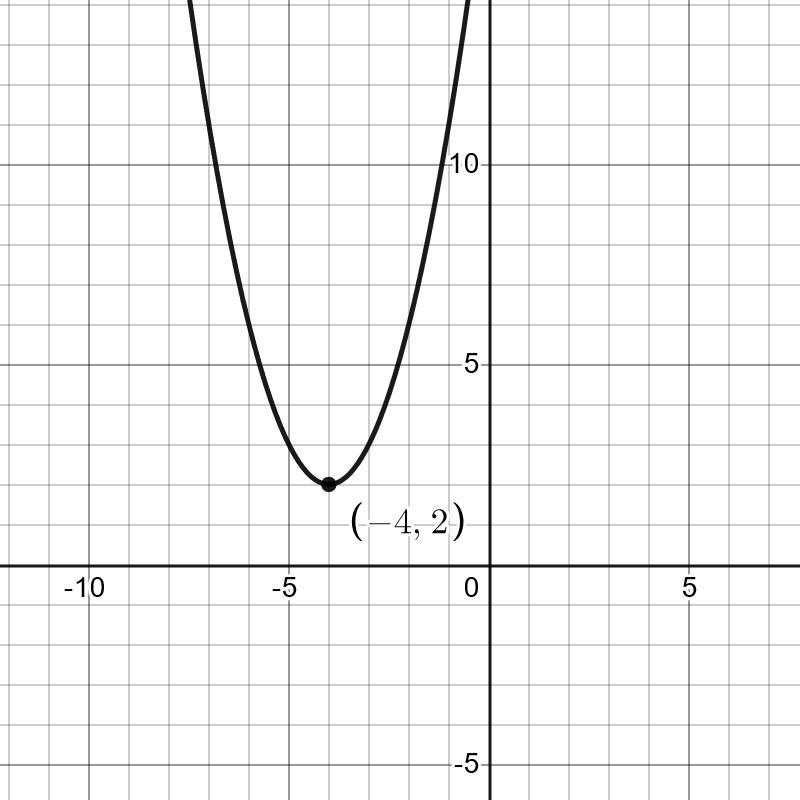


Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


