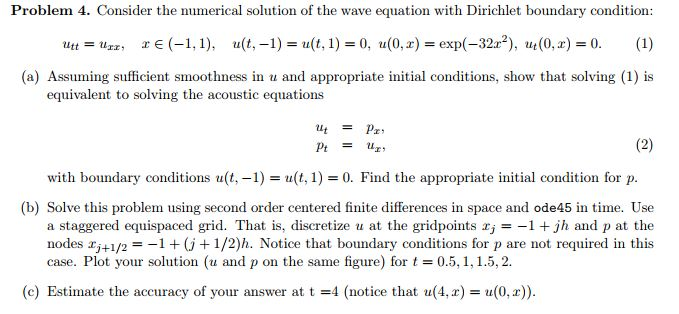
Need help!! For part a, please provide a step-by-step solution along with a detailed explanation!!!! For part b and c, please provide a step-by-step solution through the use of MALAB code along with a detailed explanation!!!! It is alright if you handwrite the step-by-step solution for part b and c but I would prefer the solution to be shown in MATLAB code. This is very URGENT!!! PLEASE reply as soon as possible!! Thank you!!

Problem 4. Consider the numerical solution of the wave equation with Dirichlet boundary condition: utt = uzz , e (-1, 1), u(t,-1)-u(t, 1-0, u(0,x) = expl--32x2), ut(0,2)-0. (1) (a) Assuming sufficient smoothness in u and appropriate initial conditions, show that solving (1) is equivalent to solving the acoustic equations Pt=Uz, with boundary conditions u(t, 1) = u(t, 1-0. Find the appropriate initial condition for p (b) Solve this problem using second order centered finite differences in space and ode45 in time. Use a staggered equispaced grid. That is, discretize u at the gridpoints aj =-1 +jh and p at the nodes +1/2 = -1 + (j + 1 /2)h. Notice that boundary conditions for p are not required in this case. Plot your solution (u and p on the same figure) for t = 0.5, 1, 1.5, 2. (c) Estimate the accuracy of your answer at t =4 (notice that u(4,x-u(0,x)). Problem 4. Consider the numerical solution of the wave equation with Dirichlet boundary condition: utt = uzz , e (-1, 1), u(t,-1)-u(t, 1-0, u(0,x) = expl--32x2), ut(0,2)-0. (1) (a) Assuming sufficient smoothness in u and appropriate initial conditions, show that solving (1) is equivalent to solving the acoustic equations Pt=Uz, with boundary conditions u(t, 1) = u(t, 1-0. Find the appropriate initial condition for p (b) Solve this problem using second order centered finite differences in space and ode45 in time. Use a staggered equispaced grid. That is, discretize u at the gridpoints aj =-1 +jh and p at the nodes +1/2 = -1 + (j + 1 /2)h. Notice that boundary conditions for p are not required in this case. Plot your solution (u and p on the same figure) for t = 0.5, 1, 1.5, 2. (c) Estimate the accuracy of your answer at t =4 (notice that u(4,x-u(0,x))