Need help on Question B. This was all the information that I have been given and figured out so far. Thank you.

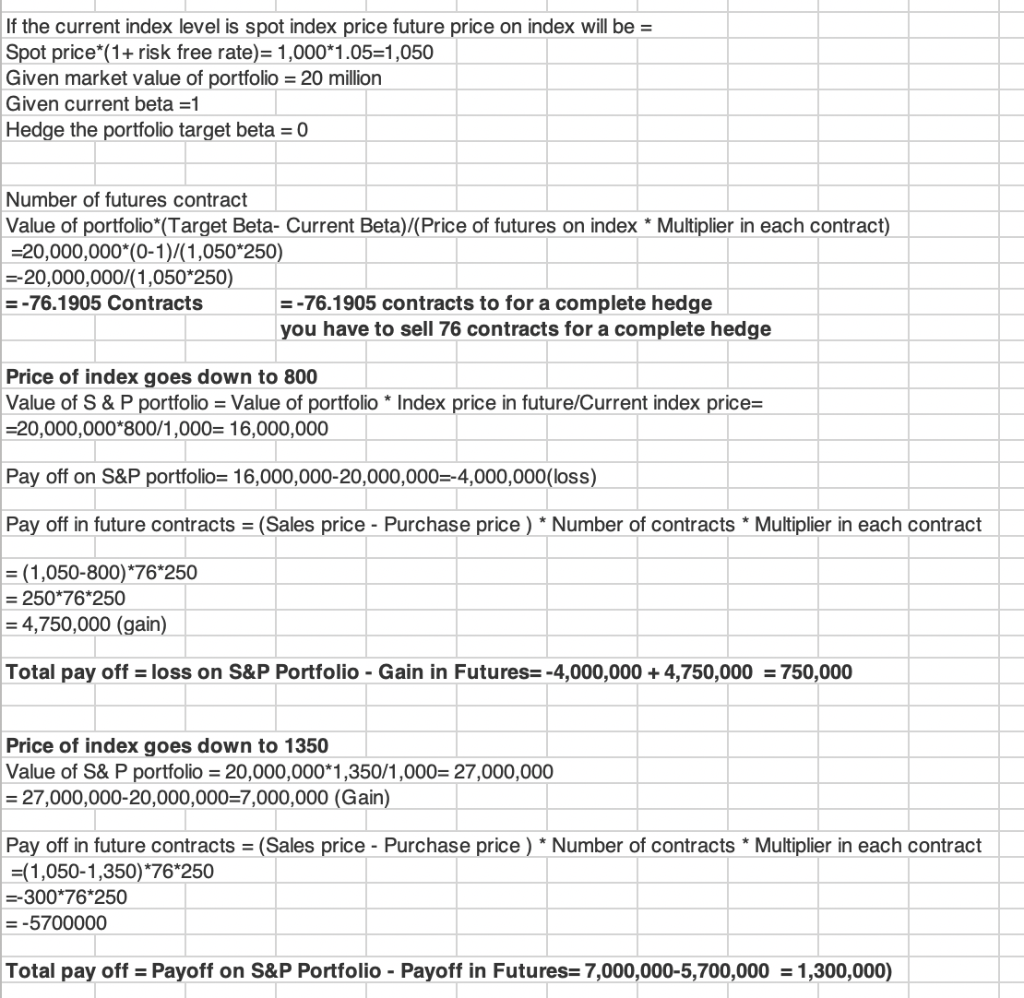
You manage the following S&P 500 portfolio: Market Value: 20 Million, Beta: 1.0, Dividends: 0%, Risk Free Rate: 5%. Futures available on the S&P 500 Index are: Current Index Level: 1,000, Beta: 1.0, Dividends: 0%, Maturity: 1 Year, Multiplier 250 Assume that the performance of the portfolio follows the capital asset pricing model (CAPM) a) You want to fully hedge the portfolio for the coming year. Recommend the hedge and show the performance of the hedge if the Index finishes the year at 800 or 1,350. b) Show the difference in the performance of the hedge between using the Spot Price and the Forward Price in computing the number of contracts used in hedging. For the 800 or 1,350 index scenarios reconcile the performance difference between both hedging outcomes. c) Assume that instead of a 1 year Futures contact the only contract you can sell is a 3 year contract. However you can buy a Futures contract for any maturity. You also want to target a portfolio Beta of 0.25 for the upcoming year. Show the performance of the hedged/adjusted portfolio if the Index finishes the year at 500 or 2,000. d) Assume that instead of a 1 year Futures contact the only contract you can sell or buy are 3 month contracts. You want to target a Beta of -2 for your portfolio for the upcoming year and will therefore need to replace with a new 3 month contract as the existing one expires. Show the performance of the hedged/adjusted portfolio if the Index finishes the first quarter at 800, the second quarter at 900, the third quarter at 950 and finished the year at 850. e) Outlined two unique benefits of Futures over Forwards, and two unique benefits of Forward over Futures. A. Market value of portfolio = 20 million Given current beta =1 Hedge the portfolio so the target beta = 0 Number of futures contract that needs to be purchased/ sold is given as = Value of portfolio * (Target Beta- Current Beta) / (Price of index * Multiplier in each contract) =20,000,000*(0-1)/(1,000*250) = -20,000,000 / 2,500,000 = -80 Contracts = 80 contracts to for a complete hedge you have to sell 80 contracts for a complete hedge Price of index goes down to 800 S&P portfolio = Value of portfolio* Index price in future/Current index price= =20,000,000*800/1,000= 16,000,000 Pay off on S&P portfolio= 16,000,000-20,000,000=-4,000,000(loss) Pay off in future contracts = (Sales price - Purchase price ) * Number of contracts * Multiplier in each contract =(1,000-800)*80*250 = 4,000,000 =200*80*250 = 4,000,000 (gain) Total pay off = loss on S&P Portfolio - Gain in Futures=-4,000,000 + 4,000,000 = 0 The payoff becomes zero which means the portfolio is completely hedged. Price of index goes down to 1350 S&P portfolio = Value of portfolio* Index price in future/Current index price= = 20,000,000 1,350/1,000= 27,000,000 Pay off on S&P portfolio= 27,000,000-20,000,000=7,000,000(Gain) Pay off in future contracts = (Sales price - Purchase price ) * Number of contracts * Multiplier in each contract =(1,000-1,350)*80*250 = 7,000,000 =-350*80*250 = -7,000,000 (loss) Total pay off = Payoff on S&P Portfolio - Payoff in Futures=7,000,000-7,000,000=0 The payoff becomes zero which means the portfolio is completely hedged. If the current index level is spot index price future price on index will be = Spot price*(1+risk free rate)= 1,000*1.05=1,050 Given market value of portfolio = 20 million Given current beta =1 Hedge the portfolio target beta = 0 Number of futures contract Value of portfolio*(Target Beta- Current Beta) (Price of futures on index * Multiplier in each contract) =20,000,000*(0-1)/(1,050*250) --20,000,000/(1,050*250) = -76.1905 Contracts = -76.1905 contracts to for a complete hedge you have to sell 76 contracts for a complete hedge Price of index goes down to 800 Value of S&P portfolio = Value of portfolio * Index price in future/Current index price= =20,000,000*800/1,000= 16,000,000 Pay off on S&P portfolio= 16,000,000-20,000,000=-4,000,000(loss) Pay off in future contracts = (Sales price - Purchase price ) * Number of contracts * Multiplier in each contract = (1,050-800)*76*250 = 250*76*250 = 4,750,000 (gain) Total pay off = loss on S&P Portfolio - Gain in Futures= -4,000,000 + 4,750,000 = 750,000 Price of index goes down to 1350 Value of S& P portfolio = 20,000,000*1,350/1,000= 27,000,000 = 27,000,000-20,000,000=7,000,000 (Gain) Pay off in future contracts = (Sales price - Purchase price ) * Number of contracts * Multiplier in each contract =(1,050-1,350)*76*250 =-300*76*250 =-5700000 Total pay off = Payoff on S&P Portfolio - Payoff in Futures= 7,000,000-5,700,000 = 1,300,000) You manage the following S&P 500 portfolio: Market Value: 20 Million, Beta: 1.0, Dividends: 0%, Risk Free Rate: 5%. Futures available on the S&P 500 Index are: Current Index Level: 1,000, Beta: 1.0, Dividends: 0%, Maturity: 1 Year, Multiplier 250 Assume that the performance of the portfolio follows the capital asset pricing model (CAPM) a) You want to fully hedge the portfolio for the coming year. Recommend the hedge and show the performance of the hedge if the Index finishes the year at 800 or 1,350. b) Show the difference in the performance of the hedge between using the Spot Price and the Forward Price in computing the number of contracts used in hedging. For the 800 or 1,350 index scenarios reconcile the performance difference between both hedging outcomes. c) Assume that instead of a 1 year Futures contact the only contract you can sell is a 3 year contract. However you can buy a Futures contract for any maturity. You also want to target a portfolio Beta of 0.25 for the upcoming year. Show the performance of the hedged/adjusted portfolio if the Index finishes the year at 500 or 2,000. d) Assume that instead of a 1 year Futures contact the only contract you can sell or buy are 3 month contracts. You want to target a Beta of -2 for your portfolio for the upcoming year and will therefore need to replace with a new 3 month contract as the existing one expires. Show the performance of the hedged/adjusted portfolio if the Index finishes the first quarter at 800, the second quarter at 900, the third quarter at 950 and finished the year at 850. e) Outlined two unique benefits of Futures over Forwards, and two unique benefits of Forward over Futures. A. Market value of portfolio = 20 million Given current beta =1 Hedge the portfolio so the target beta = 0 Number of futures contract that needs to be purchased/ sold is given as = Value of portfolio * (Target Beta- Current Beta) / (Price of index * Multiplier in each contract) =20,000,000*(0-1)/(1,000*250) = -20,000,000 / 2,500,000 = -80 Contracts = 80 contracts to for a complete hedge you have to sell 80 contracts for a complete hedge Price of index goes down to 800 S&P portfolio = Value of portfolio* Index price in future/Current index price= =20,000,000*800/1,000= 16,000,000 Pay off on S&P portfolio= 16,000,000-20,000,000=-4,000,000(loss) Pay off in future contracts = (Sales price - Purchase price ) * Number of contracts * Multiplier in each contract =(1,000-800)*80*250 = 4,000,000 =200*80*250 = 4,000,000 (gain) Total pay off = loss on S&P Portfolio - Gain in Futures=-4,000,000 + 4,000,000 = 0 The payoff becomes zero which means the portfolio is completely hedged. Price of index goes down to 1350 S&P portfolio = Value of portfolio* Index price in future/Current index price= = 20,000,000 1,350/1,000= 27,000,000 Pay off on S&P portfolio= 27,000,000-20,000,000=7,000,000(Gain) Pay off in future contracts = (Sales price - Purchase price ) * Number of contracts * Multiplier in each contract =(1,000-1,350)*80*250 = 7,000,000 =-350*80*250 = -7,000,000 (loss) Total pay off = Payoff on S&P Portfolio - Payoff in Futures=7,000,000-7,000,000=0 The payoff becomes zero which means the portfolio is completely hedged. If the current index level is spot index price future price on index will be = Spot price*(1+risk free rate)= 1,000*1.05=1,050 Given market value of portfolio = 20 million Given current beta =1 Hedge the portfolio target beta = 0 Number of futures contract Value of portfolio*(Target Beta- Current Beta) (Price of futures on index * Multiplier in each contract) =20,000,000*(0-1)/(1,050*250) --20,000,000/(1,050*250) = -76.1905 Contracts = -76.1905 contracts to for a complete hedge you have to sell 76 contracts for a complete hedge Price of index goes down to 800 Value of S&P portfolio = Value of portfolio * Index price in future/Current index price= =20,000,000*800/1,000= 16,000,000 Pay off on S&P portfolio= 16,000,000-20,000,000=-4,000,000(loss) Pay off in future contracts = (Sales price - Purchase price ) * Number of contracts * Multiplier in each contract = (1,050-800)*76*250 = 250*76*250 = 4,750,000 (gain) Total pay off = loss on S&P Portfolio - Gain in Futures= -4,000,000 + 4,750,000 = 750,000 Price of index goes down to 1350 Value of S& P portfolio = 20,000,000*1,350/1,000= 27,000,000 = 27,000,000-20,000,000=7,000,000 (Gain) Pay off in future contracts = (Sales price - Purchase price ) * Number of contracts * Multiplier in each contract =(1,050-1,350)*76*250 =-300*76*250 =-5700000 Total pay off = Payoff on S&P Portfolio - Payoff in Futures= 7,000,000-5,700,000 = 1,300,000)