need the answer as soon as possible

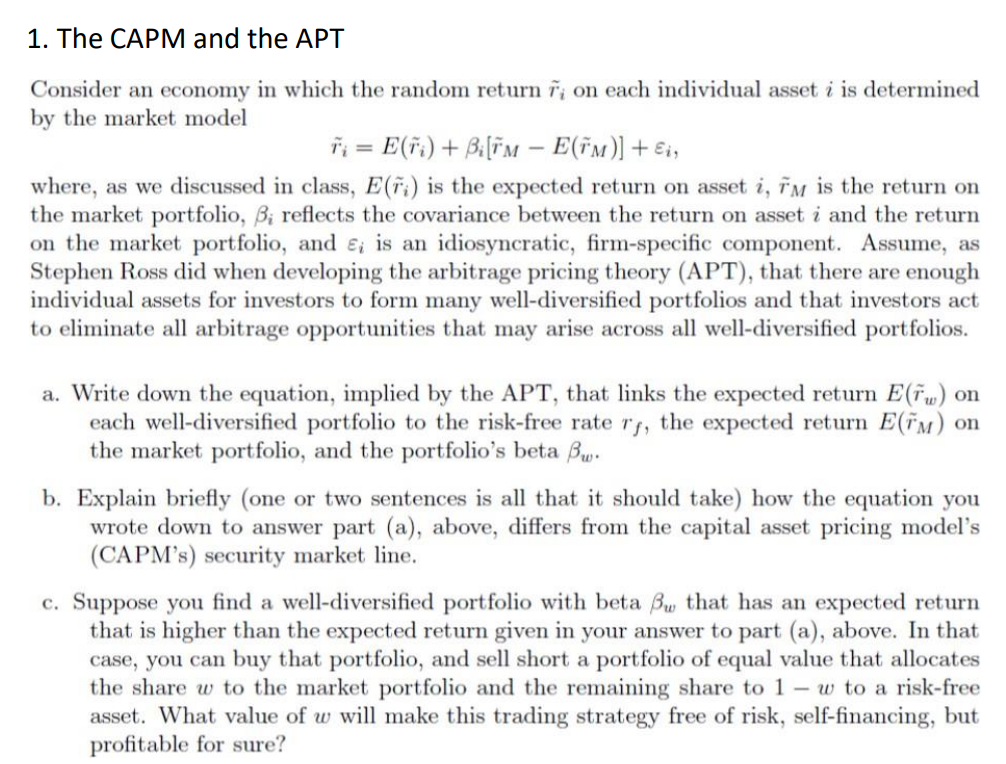
1. The CAPM and the APT Consider an economy in which the random return ti on each individual asset i is determined by the market model Ti = E(Fi) + Bi[TM E(Tm)] + Ei, where, as we discussed in class, E(Fi) is the expected return on asset i, im is the return on the market portfolio, B; reflects the covariance between the return on asset i and the return on the market portfolio, and ; is an idiosyncratic, firm-specific component. Assume, as Stephen Ross did when developing the arbitrage pricing theory (APT), that there are enough individual assets for investors to form many well-diversified portfolios and that investors act to eliminate all arbitrage opportunities that may arise across all well-diversified portfolios. a. Write down the equation, implied by the APT, that links the expected return E(Tw) on each well-diversified portfolio to the risk-free rate rs, the expected return Elim) on the market portfolio, and the portfolio's beta Bw. b. Explain briefly (one or two sentences is all that it should take) how the equation you wrote down to answer part (a), above, differs from the capital asset pricing model's (CAPM's) security market line. c. Suppose you find a well-diversified portfolio with beta Bw that has an expected return that is higher than the expected return given in your answer to part (a), above. In that case, you can buy that portfolio, and sell short a portfolio of equal value that allocates the share w to the market portfolio and the remaining share to 1 - w to a risk-free asset. What value of w will make this trading strategy free of risk, self-financing, but profitable for sure? 1. The CAPM and the APT Consider an economy in which the random return ti on each individual asset i is determined by the market model Ti = E(Fi) + Bi[TM E(Tm)] + Ei, where, as we discussed in class, E(Fi) is the expected return on asset i, im is the return on the market portfolio, B; reflects the covariance between the return on asset i and the return on the market portfolio, and ; is an idiosyncratic, firm-specific component. Assume, as Stephen Ross did when developing the arbitrage pricing theory (APT), that there are enough individual assets for investors to form many well-diversified portfolios and that investors act to eliminate all arbitrage opportunities that may arise across all well-diversified portfolios. a. Write down the equation, implied by the APT, that links the expected return E(Tw) on each well-diversified portfolio to the risk-free rate rs, the expected return Elim) on the market portfolio, and the portfolio's beta Bw. b. Explain briefly (one or two sentences is all that it should take) how the equation you wrote down to answer part (a), above, differs from the capital asset pricing model's (CAPM's) security market line. c. Suppose you find a well-diversified portfolio with beta Bw that has an expected return that is higher than the expected return given in your answer to part (a), above. In that case, you can buy that portfolio, and sell short a portfolio of equal value that allocates the share w to the market portfolio and the remaining share to 1 - w to a risk-free asset. What value of w will make this trading strategy free of risk, self-financing, but profitable for sure