Question
Newton's Law of Cooling states that the rate of cooling of an object is proportional to the temperature difference between the object and its surroundings.
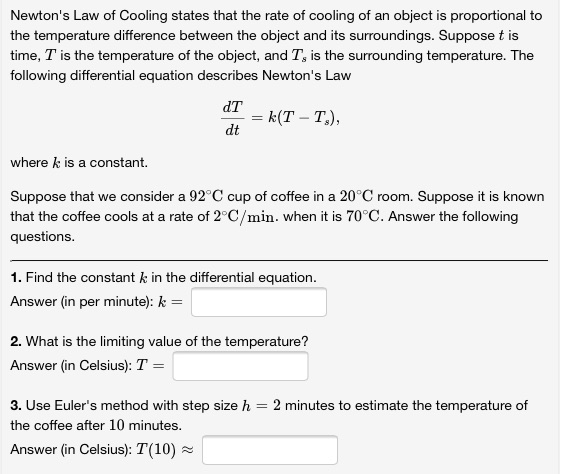
Newton's Law of Cooling states that the rate of cooling of an object is proportional to the temperature difference between the object and its surroundings. Suppose t is time, T is the temperature of the object, and Ts is the surrounding temperature. The following differential equation describes Newton's Law dT/dt =k(T?Ts) ,where k is a constant. Suppose that we consider a 92?C cup of coffee in a 20?C room. Suppose it is known that the coffee cools at a rate of 2?C/min. when it is 70?C Answer the following questions.1. Find the constant k in the differential equation. Answer (in per minute): k= 2. What is the limiting value of the temperature? Answer (in Celsius): T= 3. Use Euler's method with step size h=2 minutes to estimate the temperature of the coffee after 10 minutes. Answer (in Celsius): T(10)?

Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


