Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Newton's Method: Let f be a differentiable function on an interval I with a root in I. To approxi- mate the value of the
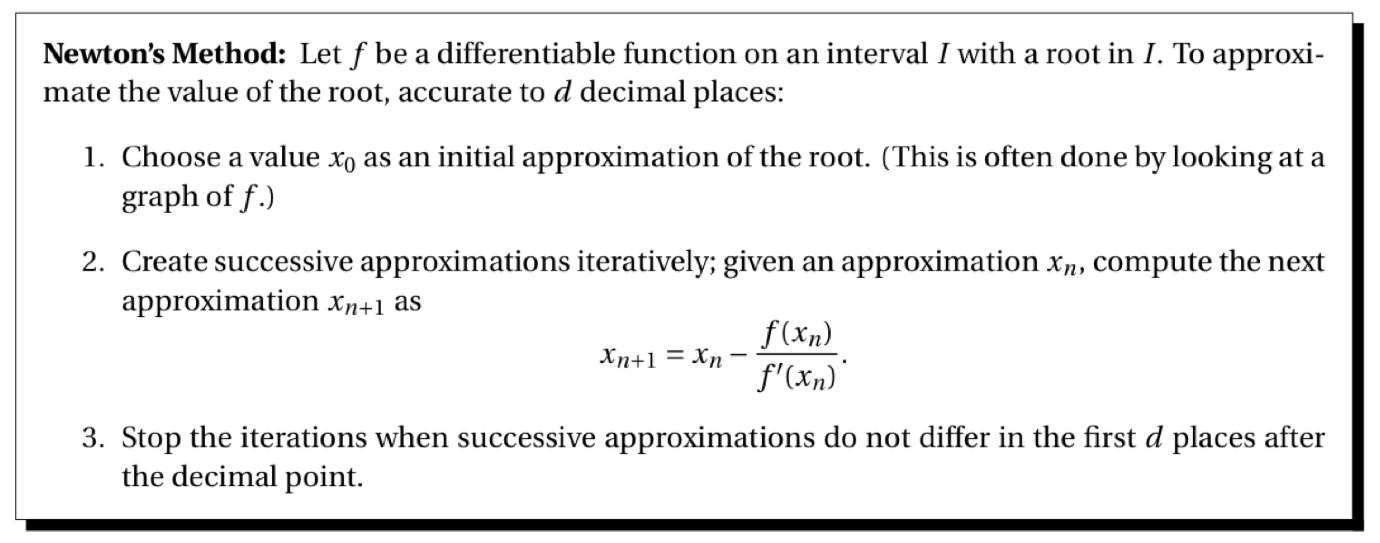
Newton's Method: Let f be a differentiable function on an interval I with a root in I. To approxi- mate the value of the root, accurate to d decimal places: 1. Choose a value xo as an initial approximation of the root. (This is often done by looking at a graph of f.) 2. Create successive approximations iteratively; given an approximation xn, compute the next approximation xn+1 as xn+1 = xn f(xn) f'(xn) 3. Stop the iterations when successive approximations do not differ in the first d places after the decimal point.
Step by Step Solution
There are 3 Steps involved in it
Step: 1
Newtons Method also known as NewtonRaphson method is an iterative method for finding the roots of a ...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


