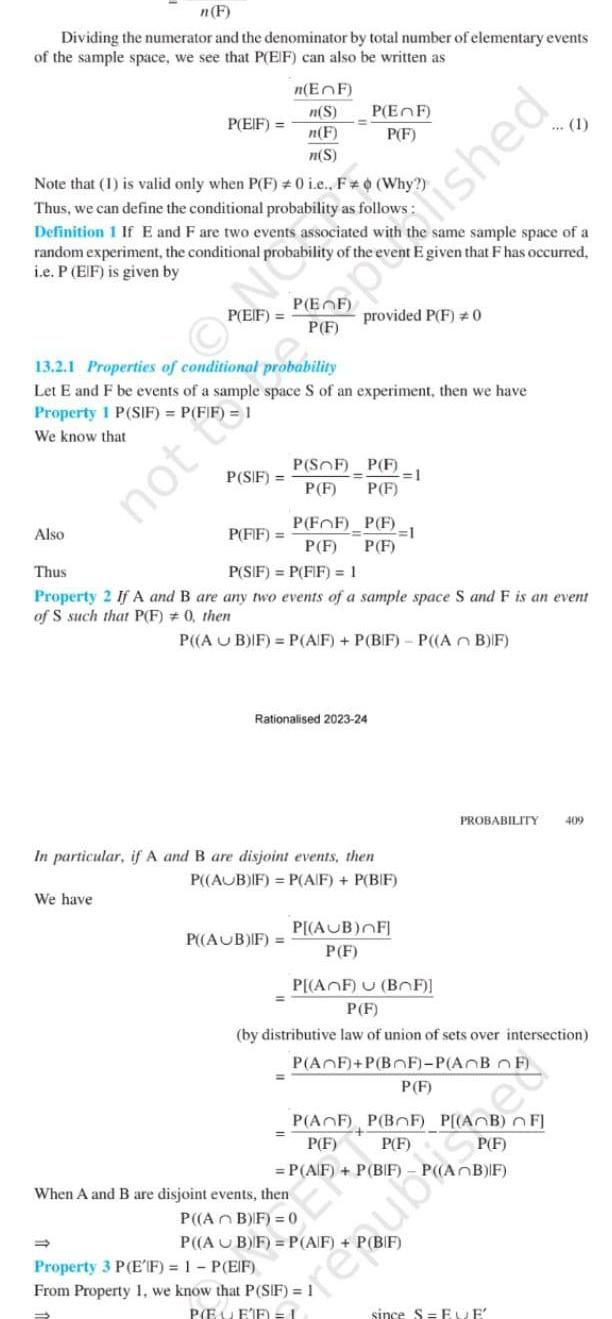
n(F) Dividing the numerator and the denominator by total number of elementary events of the sample space, we see that P(EIF) can also be written as n(EF) n(S) P(EF) P(EIF) = n(F) P(F) n(S) Note that (1) is valid only when P(F) #0 i.e., F# (Why?) Thus, we can define the conditional probability as follows: . (1) Definition 1 If E and F are two events associated with the same sample space of a random experiment, the conditional probability of t i.e. P (EIF) is given by E given that F has occurred, not to polished P(EIF) 13.2.1 Properties of conditional probability provided P(F) #0 Let E and F be events of a sample space S of an experiment, then we have Property 1 P(SIF) = P(F)F) = 1 We know that Also P(SOF) P(F) P(SIF) = =1 P(F) P(F) P(FOF) P(F) P(FIF) = P(F) P(F) Thus P(SIF) = P(FIF) = 1 Property 2 If A and B are any two events of a sample space S and F is an event of S such that P(F) +0, then P((AUB)|F) = P(AF) + P(BIF) - P((AB)|F) Rationalised 2023-24 In particular, if A and B are disjoint events, then We have P((AUB)IF) = P(A|F) + P(BIF) P[(AUB)F] P((AUB)|F) = P(F) PROBABILITY 409 P[(AOF) (BOF)] P(F) (by distributive law of union of sets over intersection) P(AOF)+P(BOF)-P(ABF) P(F) P(AOF) P(BOF) P[(AB)F] P(F) P(F) P(F) = P(AF) + P(BIF) - P((AB)|F) When A and B are disjoint events, then P((AB)|F)=0 P((AB)F) = P(A|F) + BIF) Property 3 P(EIF) = 1 - P(EIF) From Property 1, we know that P(SIF) = 1 P(EEE)=1 since SEUE