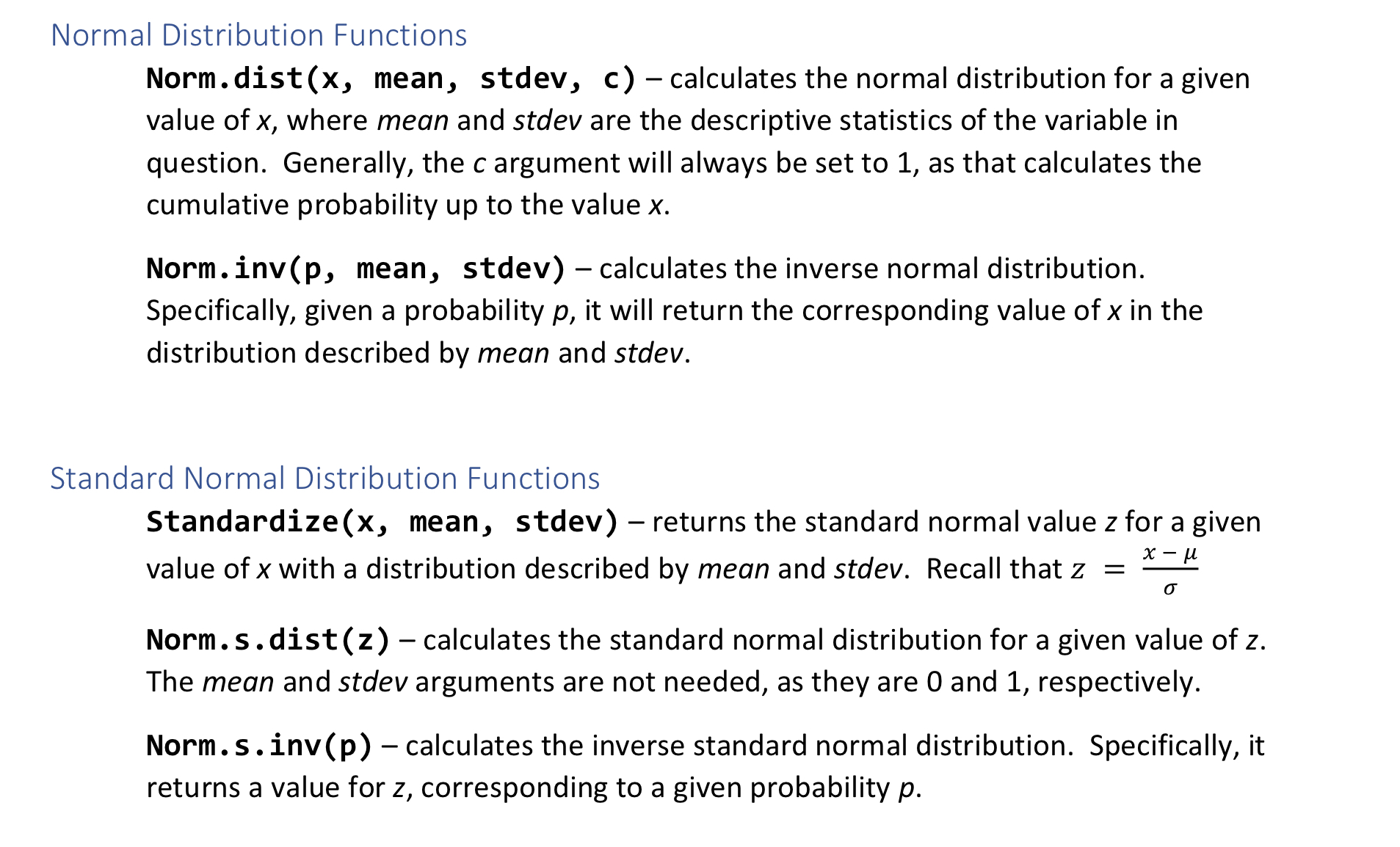
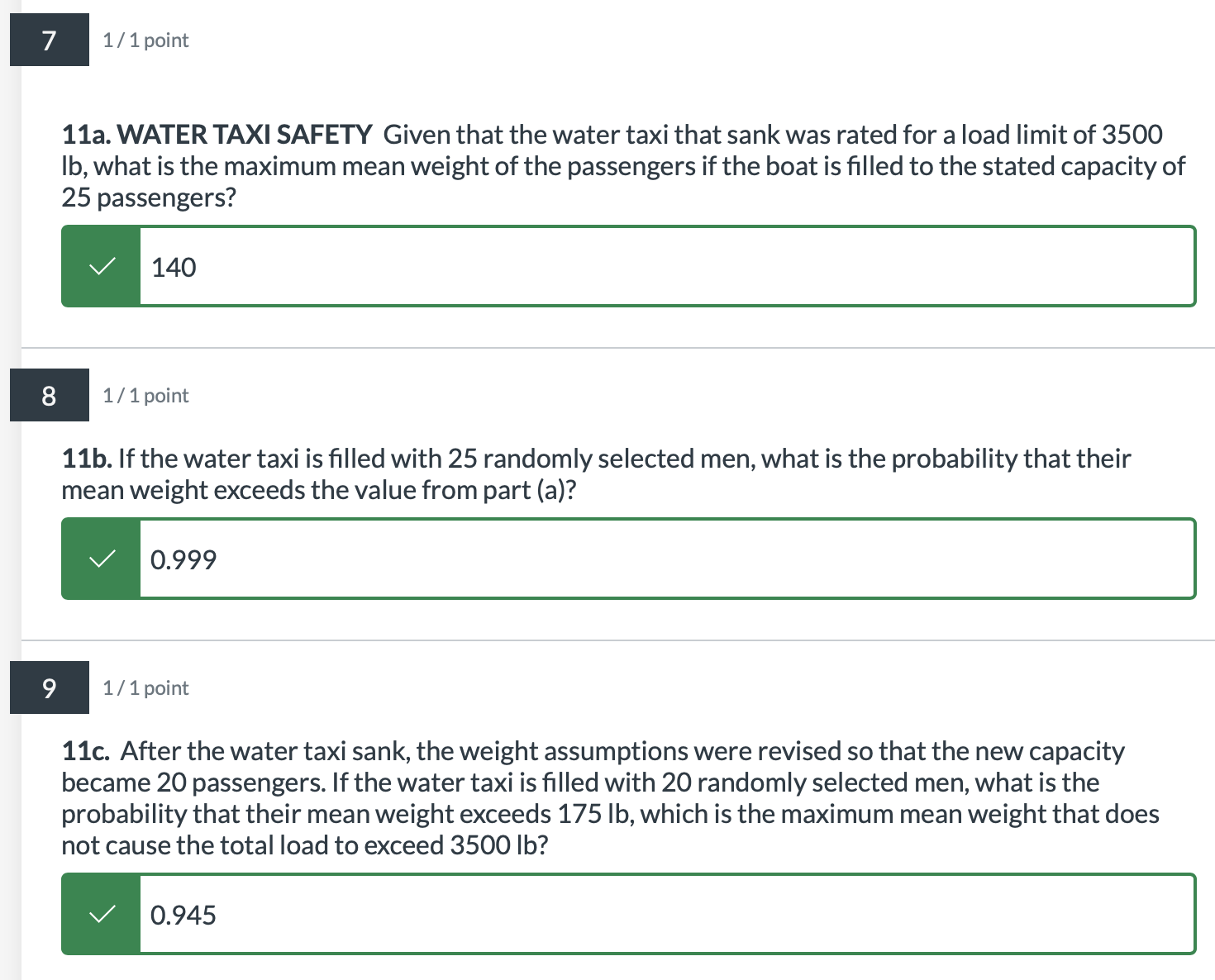
Normal Distribution Functions Norm.dist(x, mean, stdev, c) - calculates the normal distribution for a given value of x, where mean and stdev are the descriptive statistics of the variable in question. Generally, the c argument will always be set to 1, as that calculates the cumulative probability up to the value x. Norm.inv(p, mean, stdev) - calculates the inverse normal distribution. Specifically, given a probability p, it will return the corresponding value of x in the distribution described by mean and stdev. Standard Normal Distribution Functions = x- - Standardize(x, mean, stdev) - returns the standard normal value z for a given value of x with a distribution described by mean and stdev. Recall that z Norm.s.dist(z) - calculates the standard normal distribution for a given value of z. The mean and stdev arguments are not needed, as they are 0 and 1, respectively. Norm.s.inv(p) - calculates the inverse standard normal distribution. Specifically, it returns a value for z, corresponding to a given probability p. 7 1/1 point 8 9 11a. WATER TAXI SAFETY Given that the water taxi that sank was rated for a load limit of 3500 Ib, what is the maximum mean weight of the passengers if the boat is filled to the stated capacity of 25 passengers? 140 1/1 point 11b. If the water taxi is filled with 25 randomly selected men, what is the probability that their mean weight exceeds the value from part (a)? 0.999 1/1 point 11c. After the water taxi sank, the weight assumptions were revised so that the new capacity became 20 passengers. If the water taxi is filled with 20 randomly selected men, what is the probability that their mean weight exceeds 175 lb, which is the maximum mean weight that does not cause the total load to exceed 3500 lb? 0.945