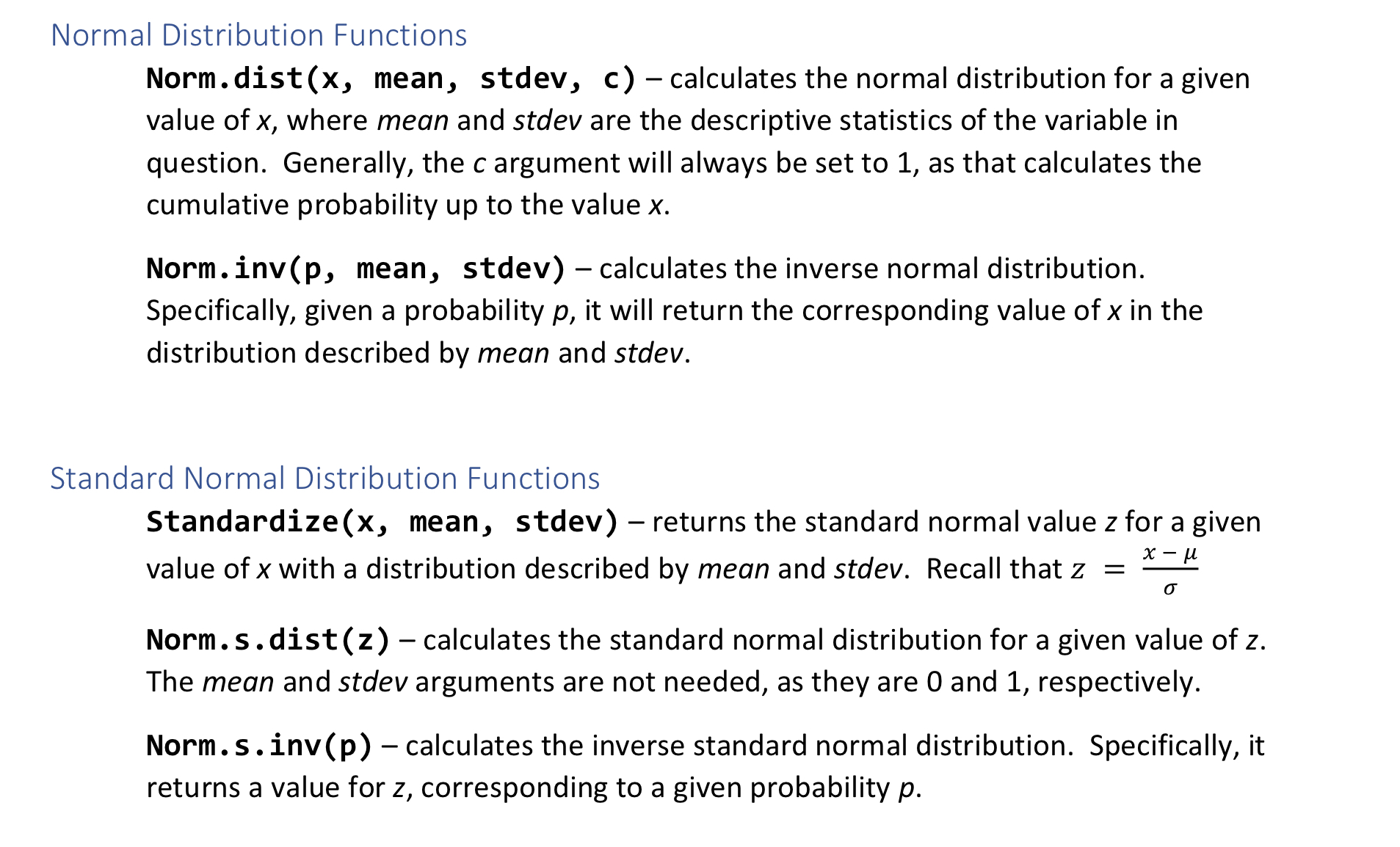
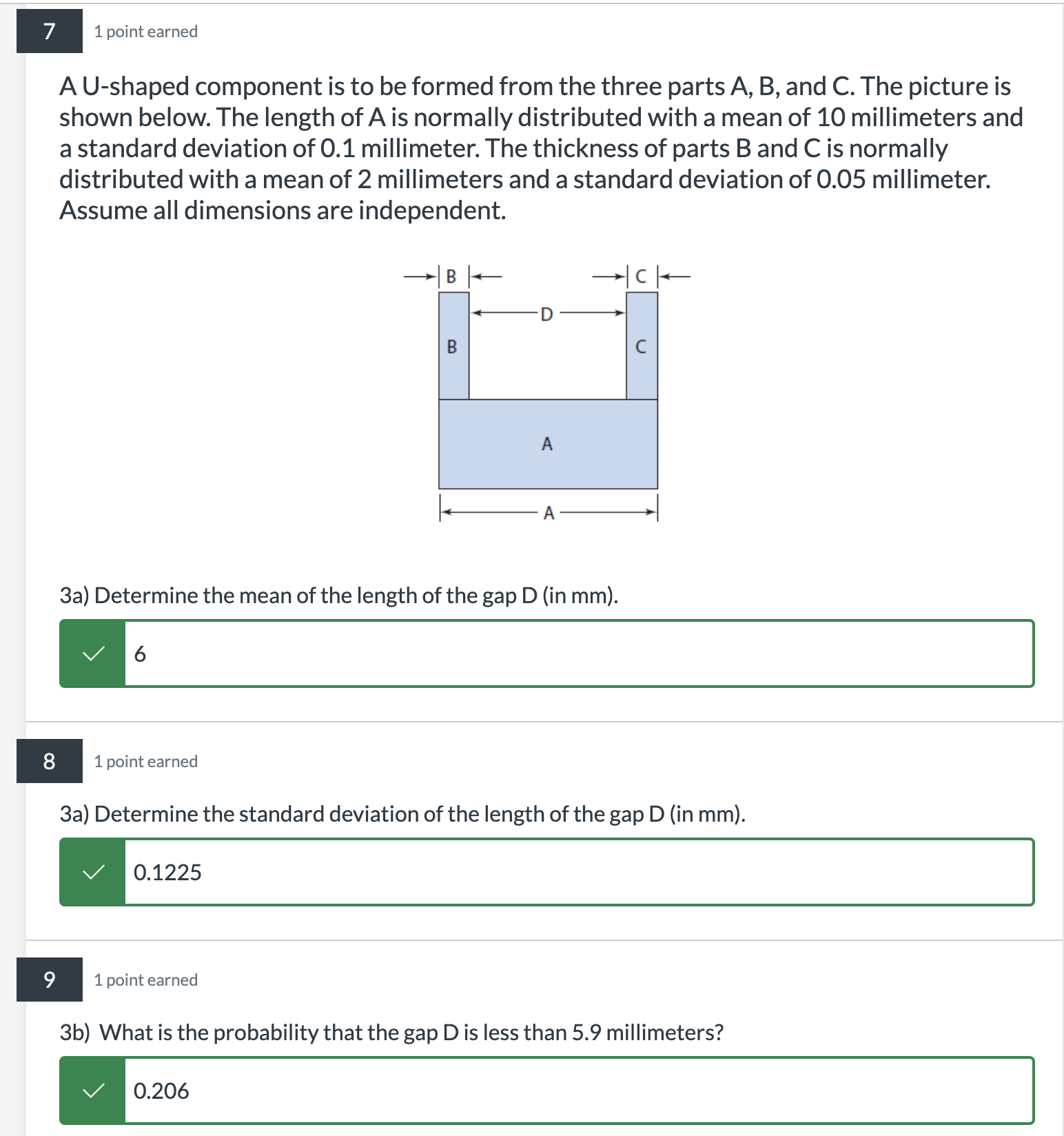
Normal Distribution Functions Norm.dist(x, mean, stdev, c) - calculates the normal distribution for a given value of x, where mean and stdev are the descriptive statistics of the variable in question. Generally, the c argument will always be set to 1, as that calculates the cumulative probability up to the value x. Norm.inv(p, mean, stdev) - calculates the inverse normal distribution. Specifically, given a probability p, it will return the corresponding value of x in the distribution described by mean and stdev. Standard Normal Distribution Functions = x- - Standardize(x, mean, stdev) - returns the standard normal value z for a given value of x with a distribution described by mean and stdev. Recall that z Norm.s.dist(z) - calculates the standard normal distribution for a given value of z. The mean and stdev arguments are not needed, as they are 0 and 1, respectively. Norm.s.inv(p) - calculates the inverse standard normal distribution. Specifically, it returns a value for z, corresponding to a given probability p. 9 8 7 1 point earned A U-shaped component is to be formed from the three parts A, B, and C. The picture is shown below. The length of A is normally distributed with a mean of 10 millimeters and a standard deviation of 0.1 millimeter. The thickness of parts B and C is normally distributed with a mean of 2 millimeters and a standard deviation of 0.05 millimeter. Assume all dimensions are independent. B A 3a) Determine the mean of the length of the gap D (in mm). 6 1 point earned 3a) Determine the standard deviation of the length of the gap D (in mm). 0.1225 1 point earned 3b) What is the probability that the gap D is less than 5.9 millimeters? 0.206