**Number Theory** Please do numbers 30, 31, 32. these are all related. Thank you!

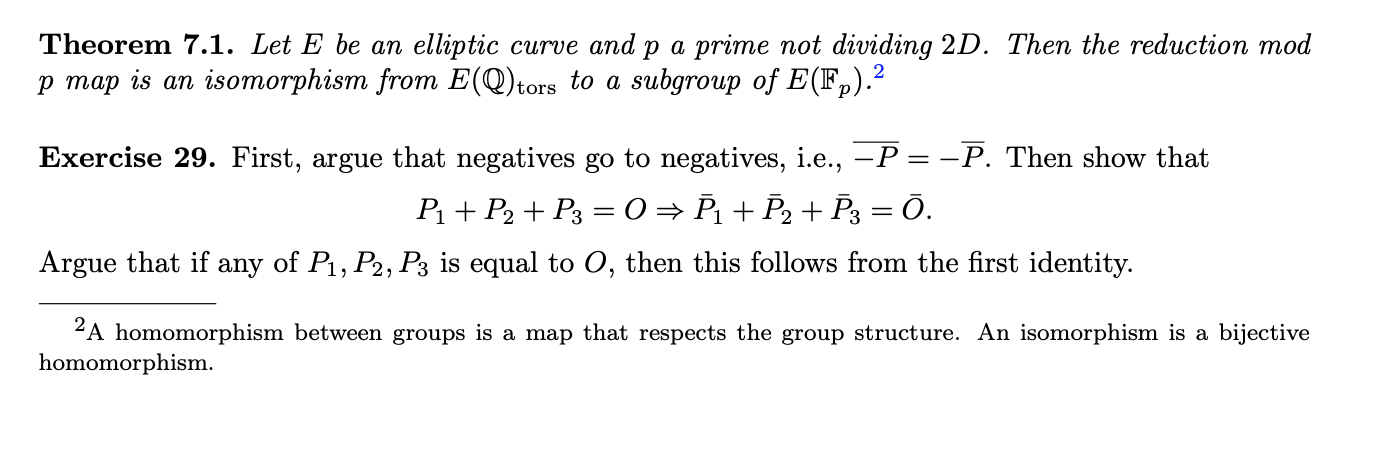

Start with E an elliptic curve y2 = x3 + ax2 +bx+c. Change variables (x, y) (**) to get Y2Z = X3 +aX2Z + 6X Z2 + cz. When Z= 0, we get X3 = 0 and call that the point at infinity, labelled O. It is a triple root, this means that the cubic meets the line at infinity in three points, but the points are all the same. PQ Q P P+Q We are going to learn how to do arithmetic with rational points on E. Using the scheme above, we can see that the 'negative' of a point P = (x, y) on E is the reflection along the x-axis - P = (2, -y), where by negative, we mean that P +(-P) = 0. Now set P= (x1, y), Q = (x2, 42), P*Q= (x3, 43), P+Q= (x3,-43). m = = Exercise 22. Assume that P and Q are given as above, and let's compute P+Q. First show that the line joining P and Q is given by Y2 - Y1 y = mx + d. d=yi mai = y2 - mx2. C2 - 21 Substitute y = mx +c into y2 = r+ ax2 + bx+c and rearrange to get + (a m)x2 + (6 2md)x +(c - d?). This is a cubic equation in x. We know that x1, x2 are roots, so let L3 be the third one. Therefore +(a m)x2 + (6 2md)x + (c- d?) = (x - :- 81)(x x2)(x X3). Expand and compare the coefficient of x2 on both sides to get a m = -11 22 Z3, so that x3 = m- - a - 21 - 22, Y3 = mx3 + d. 0 =23 - Theorem 7.1. Let E be an elliptic curve and p a prime not dividing 2D. Then the reduction mod p map is an isomorphism from E(Q)tors to a subgroup of E(Fp).2 Exercise 29. First, argue that negatives go to negatives, i.e., - P=-P. Then show that P1 + P2 + P3 = + P2 + P3 = 7. Argue that if any of P1, P2, P3 is equal to O, then this follows from the first identity. - 2A homomorphism between groups is a map that respects the group structure. An isomorphism is a bijective homomorphism. 2 Exercise 30. Continuing from the previous exercise, now assume that none of P1, P2, P3 are equal to O. Write P1 = (x1, y), P2 = (x2, y2), P3 = (x3, Y3). Argue that Pi + P2 + P3 O implies that P1, P2, P3 lie on a straight line. Do this by substituting the equation of the line into the cubic as in Exercise 22 and reduce mod p, and also reducing the equations Yi = mxi + d i= 1,2,3 mod p. Finally, argue that the map is bijective by showing that only PH if and only if P=0. In other words, the only element that maps to O is . Now let's see how this is useful. Pick one of the following examples to work out. =- 2 Exercise 31. Let E:y2 = x3 + x. Check that D = -35, so the theorem holds for all p > 5. Check that \E(F5)] = 6 and E(F7)| = 13. This means that E(Q)tors| must divide 6 and 13, so it must be trivial. : == = Exercise 32. Let E : y = x3 43x+166. Check that D= -215. 13. Then verify that P= (3,8) is an integral point on E and has order 7, by computing 2P, 4P, and 8P. Now check that \E(F3)] = 7, and use this to conclude that |E(Q)tors| must divide 7, and in fact it is equal to 7. Start with E an elliptic curve y2 = x3 + ax2 +bx+c. Change variables (x, y) (**) to get Y2Z = X3 +aX2Z + 6X Z2 + cz. When Z= 0, we get X3 = 0 and call that the point at infinity, labelled O. It is a triple root, this means that the cubic meets the line at infinity in three points, but the points are all the same. PQ Q P P+Q We are going to learn how to do arithmetic with rational points on E. Using the scheme above, we can see that the 'negative' of a point P = (x, y) on E is the reflection along the x-axis - P = (2, -y), where by negative, we mean that P +(-P) = 0. Now set P= (x1, y), Q = (x2, 42), P*Q= (x3, 43), P+Q= (x3,-43). m = = Exercise 22. Assume that P and Q are given as above, and let's compute P+Q. First show that the line joining P and Q is given by Y2 - Y1 y = mx + d. d=yi mai = y2 - mx2. C2 - 21 Substitute y = mx +c into y2 = r+ ax2 + bx+c and rearrange to get + (a m)x2 + (6 2md)x +(c - d?). This is a cubic equation in x. We know that x1, x2 are roots, so let L3 be the third one. Therefore +(a m)x2 + (6 2md)x + (c- d?) = (x - :- 81)(x x2)(x X3). Expand and compare the coefficient of x2 on both sides to get a m = -11 22 Z3, so that x3 = m- - a - 21 - 22, Y3 = mx3 + d. 0 =23 - Theorem 7.1. Let E be an elliptic curve and p a prime not dividing 2D. Then the reduction mod p map is an isomorphism from E(Q)tors to a subgroup of E(Fp).2 Exercise 29. First, argue that negatives go to negatives, i.e., - P=-P. Then show that P1 + P2 + P3 = + P2 + P3 = 7. Argue that if any of P1, P2, P3 is equal to O, then this follows from the first identity. - 2A homomorphism between groups is a map that respects the group structure. An isomorphism is a bijective homomorphism. 2 Exercise 30. Continuing from the previous exercise, now assume that none of P1, P2, P3 are equal to O. Write P1 = (x1, y), P2 = (x2, y2), P3 = (x3, Y3). Argue that Pi + P2 + P3 O implies that P1, P2, P3 lie on a straight line. Do this by substituting the equation of the line into the cubic as in Exercise 22 and reduce mod p, and also reducing the equations Yi = mxi + d i= 1,2,3 mod p. Finally, argue that the map is bijective by showing that only PH if and only if P=0. In other words, the only element that maps to O is . Now let's see how this is useful. Pick one of the following examples to work out. =- 2 Exercise 31. Let E:y2 = x3 + x. Check that D = -35, so the theorem holds for all p > 5. Check that \E(F5)] = 6 and E(F7)| = 13. This means that E(Q)tors| must divide 6 and 13, so it must be trivial. : == = Exercise 32. Let E : y = x3 43x+166. Check that D= -215. 13. Then verify that P= (3,8) is an integral point on E and has order 7, by computing 2P, 4P, and 8P. Now check that \E(F3)] = 7, and use this to conclude that |E(Q)tors| must divide 7, and in fact it is equal to 7









