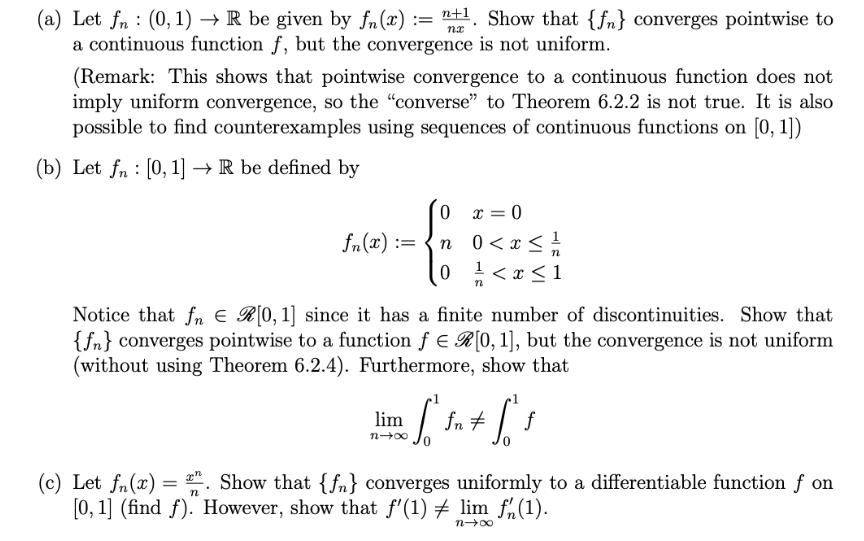
nx (a) Let f (0, 1) R be given by fn(x) = +1. Show that {f} converges pointwise to a continuous function f, but the convergence is not uniform. (Remark: This shows that pointwise convergence to a continuous function does not imply uniform convergence, so the "converse" to Theorem 6.2.2 is not true. It is also possible to find counterexamples using sequences of continuous functions on [0, 1]) (b) Let f [0,1] R be defined by 0x = 0 fn(2):= n 0 < x 1 0 < x