On the last example we worked on a sample of a nine inductor DC resistance, a measured by a associate Mr. Ryan for a proprietary subassembly. Sample mean and standard deviation were determined to be 45.69 and 0.1965 m?, respectively.

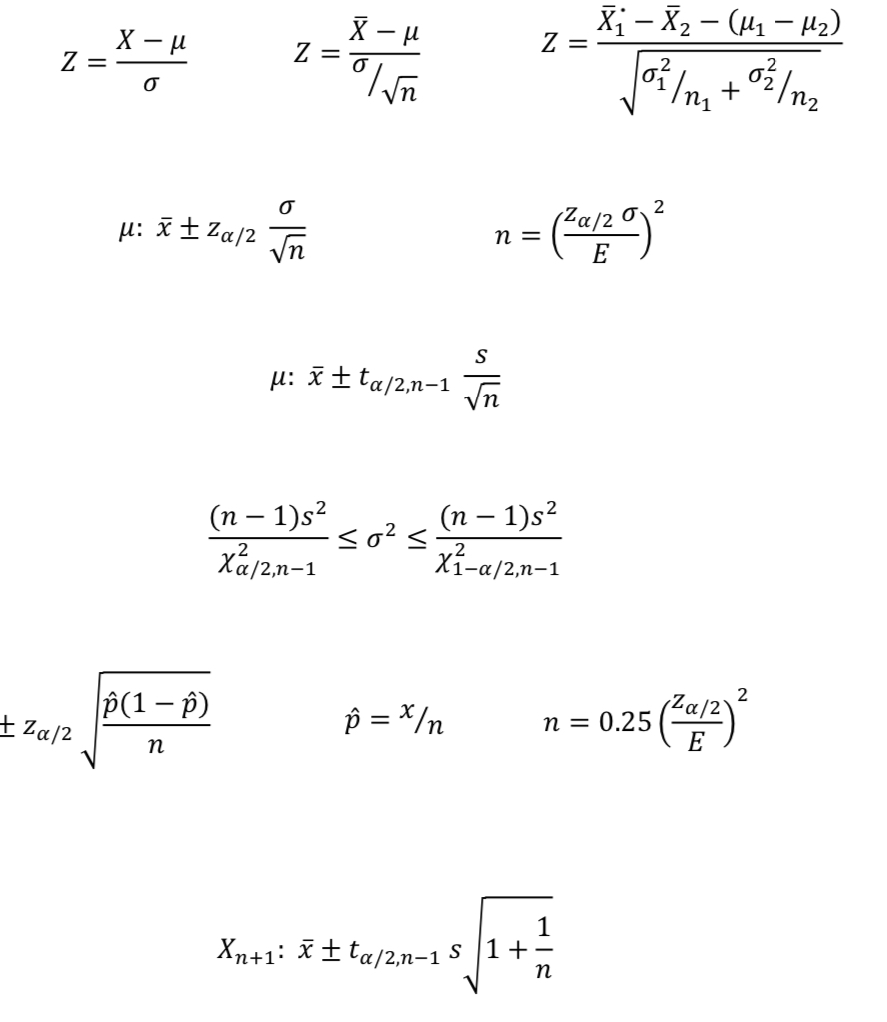
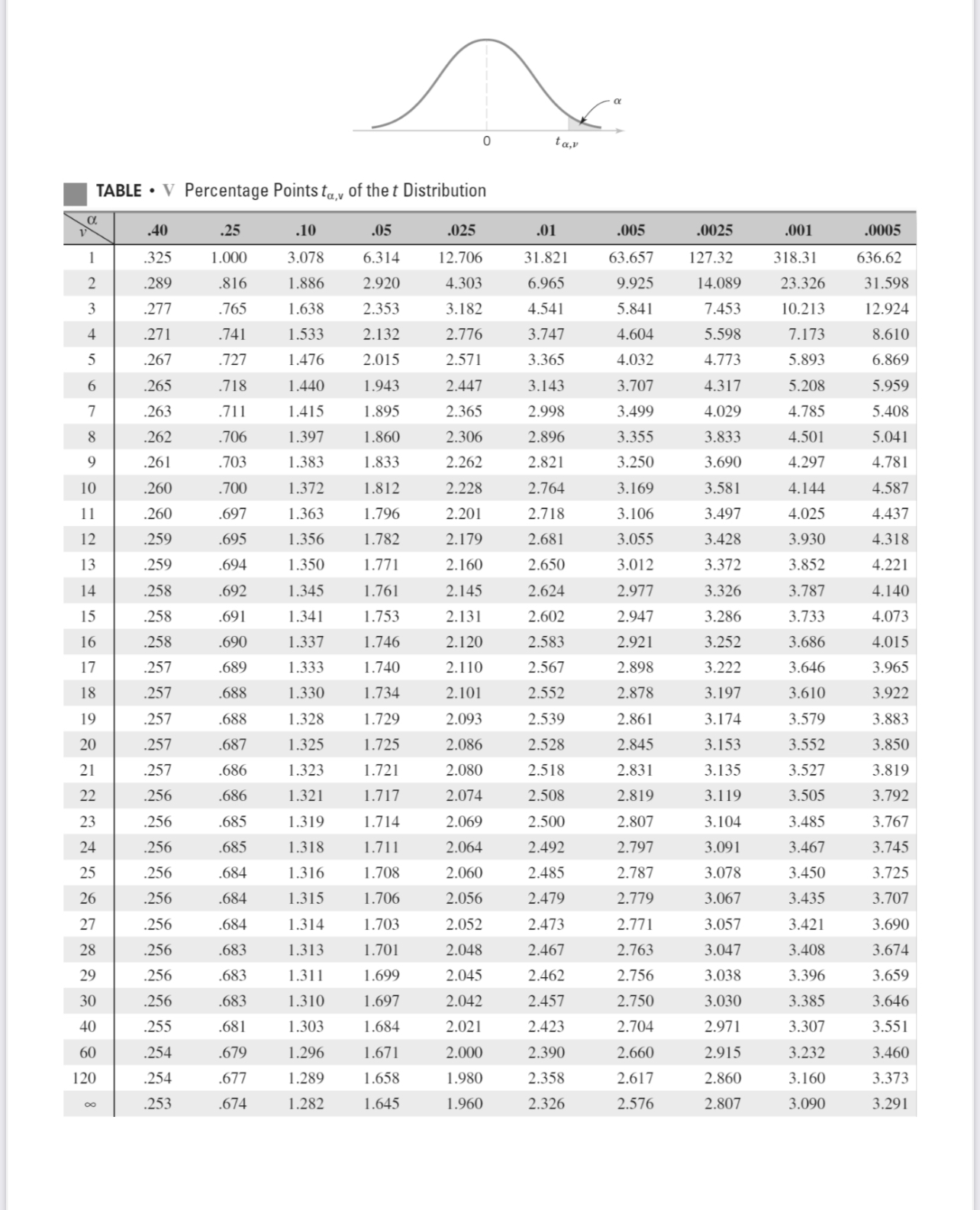
a. Write lower 95% confidence bound on the mean value of the DC resistance. Assume the unknown population standard deviation. Include the unit and draw the bound against the correct distribution.

b. Write the lower 95% prediction bound on the next value of a inductor DC resistance. Make sure that the unit is included.

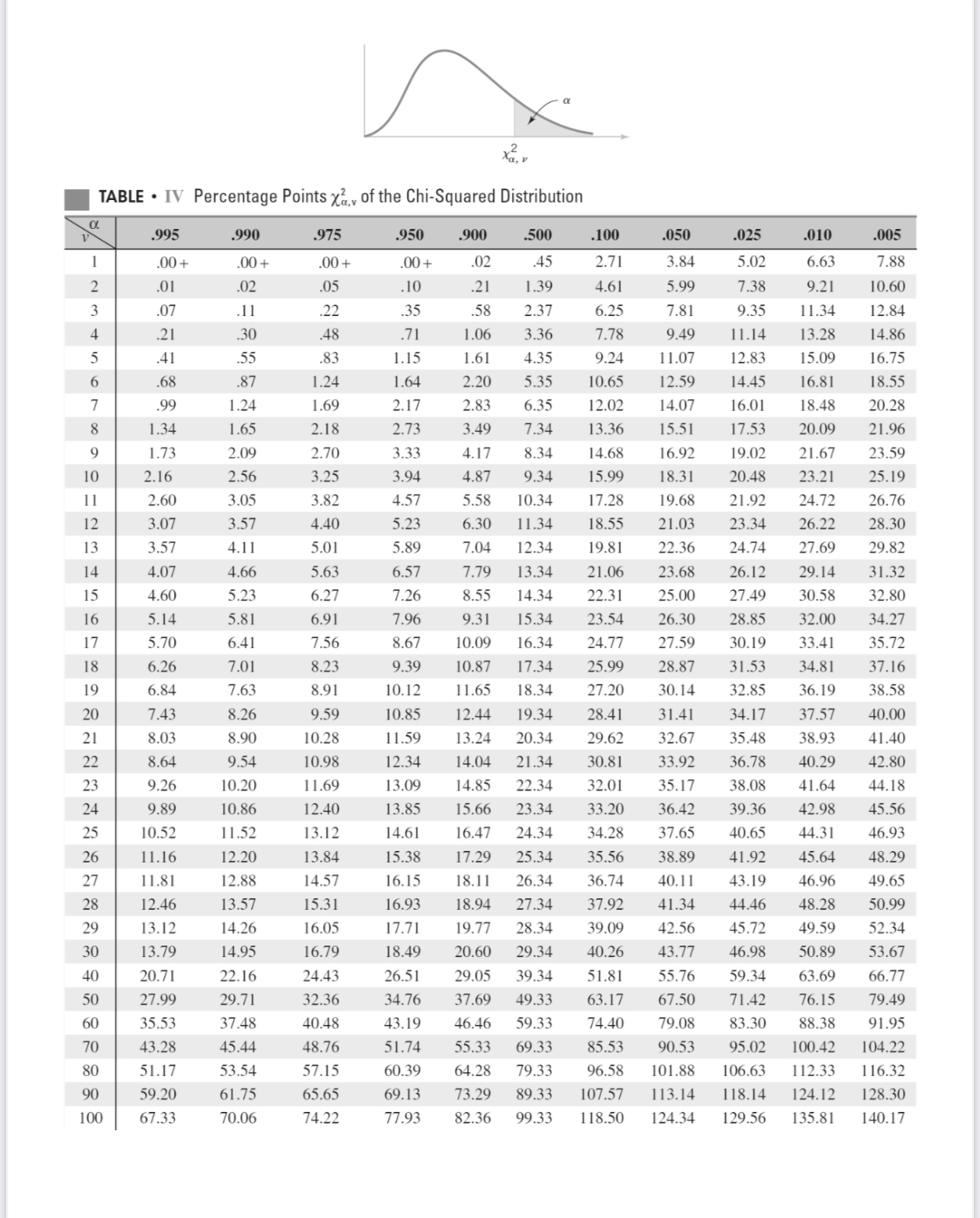
c. Write the upper 99% confidence bound on the standard deviation of inductor DC resistance.Draw the bound against the correct distribution and with an included unit.

This is the original data, all in m?. {45.4 45.4 45.7 45.7 45.7 45.7 45.7 45.9 46.0} e. DC resistances that are over 45.8 m? is considered out-of-spec, write a 95% confidence interval for the proportion of out-of- spec inductors. Just Ignore the large sample requirement and compute it anyway.

f. What sample size ensure to us that this interval is no wider than 2%?

Use table percentage points of the Chi squared distribution in order to solve this problem.

X - ul X - ul Xi - X2 - (M1 - M2) Z = Z = Z= 10 1 + 02 / n2 (Za/2 0 2 M: x + za/2 In n = E S u: x t ta/2, n-1 Vn (n - 1)s2 (n - 1)s2 502