Question: Only need help with the second part which starts with consider the same problem as above, but suppose.... Thanks a lot! Consider a finite-horizon investment

Only need help with the second part which starts with "consider

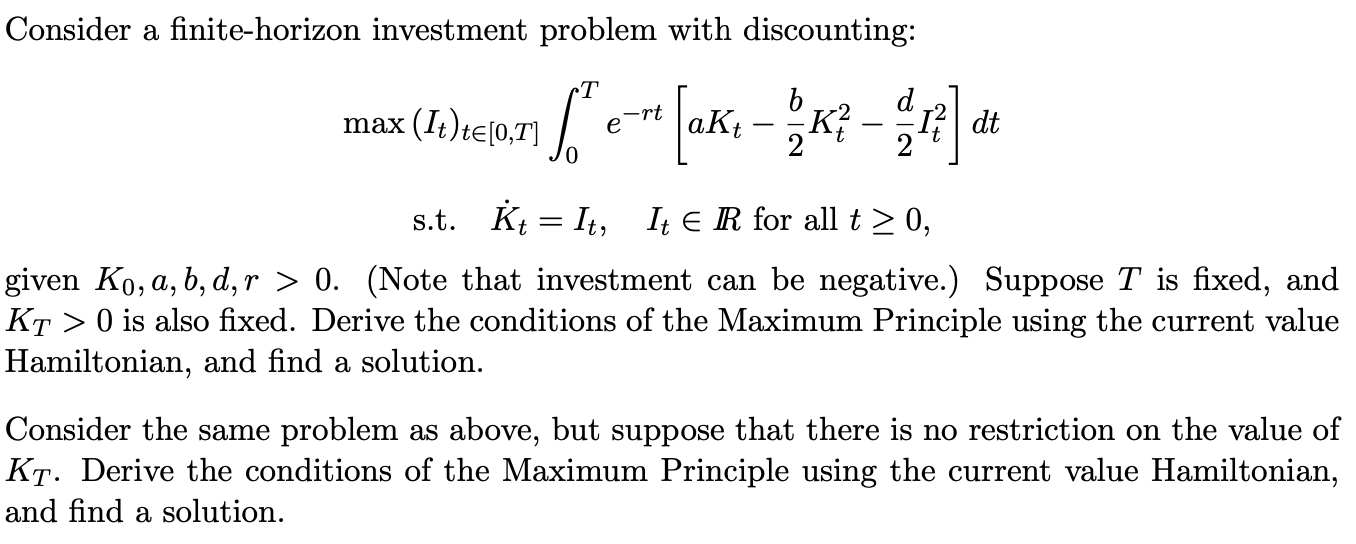
Only need help with the second part which starts with "consider the same problem as above, but suppose...". Thanks a lot!

Consider a finite-horizon investment problem with discounting: max(It)t[0,T]0Tert[aKt2bKt22dIt2]dt s.t. Kt=It,ItR for all t0, given K0,a,b,d,r>0. (Note that investment can be negative.) Suppose T is fixed, and KT>0 is also fixed. Derive the conditions of the Maximum Principle using the current value Hamiltonian, and find a solution. Consider the same problem as above, but suppose that there is no restriction on the value of KT. Derive the conditions of the Maximum Principle using the current value Hamiltonian, and find a solution. Consider a finite-horizon investment problem with discounting: max(It)t[0,T]0Tert[aKt2bKt22dIt2]dt s.t. Kt=It,ItR for all t0, given K0,a,b,d,r>0. (Note that investment can be negative.) Suppose T is fixed, and KT>0 is also fixed. Derive the conditions of the Maximum Principle using the current value Hamiltonian, and find a solution. Consider the same problem as above, but suppose that there is no restriction on the value of KT. Derive the conditions of the Maximum Principle using the current value Hamiltonian, and find a solution

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Accounting Questions!

PowerPoint Assignment for BUS 401: Principles of Finance You have been asked by a manager in your organization to put together a training program explaining Net Present Value (NPV) and Future Value...

uantitative Analysis BA 452 Homework 3 Questions Homework 3 covers the theory and applications in Lessons I-6 and I-7. This document has four parts: Objectives of doing your homework. Assignment of...

Summarise this article for presentation How to Stop Customers from Fixating on Price At a consumer products company we're familiar with, no one on the senior team would ever refer to the company's...

Help me please. In the month of September, all of the walmart grocery stores in Virginia sold 429,382 eggs. In that same month, all of the walmart grocery stores in Texas sold 283,054 eggs. How many...

By MARK@ (Ruska, Markevichus, Naniev, Grachev) Emerging russian ice-cream market is good area for different producers as it has great potential 1. Currently Russian ice-cream market is the 4th...

Please help me to write a summary for attached article (about 900 words) Accounting Horizons Vol. 22, No. 4 2008 pp. 453-470 American Accounting Association DOI: 10.2308/acch.2008.22.4.453 On the...

assay of what you may think is important and what can be interesting from this reading. Does technology make your life easier? 1.1 Introduction: the "Easier-life Thesis" It is commonly repeated that...

TACKLE ALL PARTSP5 Problem 1 The Airfare Problem1. You are trying to get the cheapest airfare that you can. You just called up and found that the ticket home will cost $400, and it cannot be refunded...

Prepare the financial analysis to support your decision between bankruptcy,voluntary reorganization,and Chapter11reorganization.It must be complete and prepared in a professional level,with the...

Q1-Explain how the EBIT Chart works (inputs determining the outputs-the two lines on the chart Case Leahy Bread Company (LBC) in Cohen Finance Workbook, page 111( a 'caselette') SEE THE IS/BS MODEL &...

The rings below are joined by a double bond that undergoes cis-trans isomerization much more readily than the bond of a typical alkene. Provide an explanation.

Prove Theorem 1.4. Assume that V and W are vector spaces of dimensions n and m with bases B and D, and that h: V W is a linear map. If h is represented by and V is represented by then the...

The one - year discount factor, at a discount rate of 2 5 percent per year, is: Multiple Choice 0 . 8 . 1 . 0 . 0 . 7 5 . 1 . 2 5 .

5. Develop a scenario comparing two PH programs and involving the use of a CBA.

identify potential ethical and environmental issues about pay and reward.

explain the importance of developing a pay and reward strategy for an organisation

2. Interview managers or employees from three organisations of your choice from different industrial sectors to establish what effect, if any, the introduction of the living wage has or would have on...