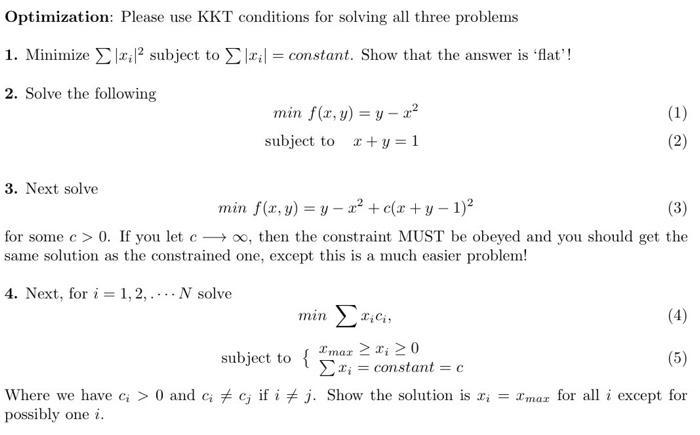
Optimization: Please use KKT conditions for solving all three problems 1. Minimize x2 subject to |x| = constant. Show that the answer is "flat"! 2. Solve the following min f(x, y) = y x2 subject to x+y=1 3. Next solve min f(x,y) = y=x+c(x + y 1) (1) (2) (3) for some c>0. If you let coo, then the constraint MUST be obeyed and you should get the same solution as the constrained one, except this is a much easier problem! 4. Next, for i = 1, 2,... N solve minaci subject to { Imax x; 0 constant: = C (4) (5) Where we have c; > 0 and cc, if i j. Show the solution is x = max for all i except for possibly one i.