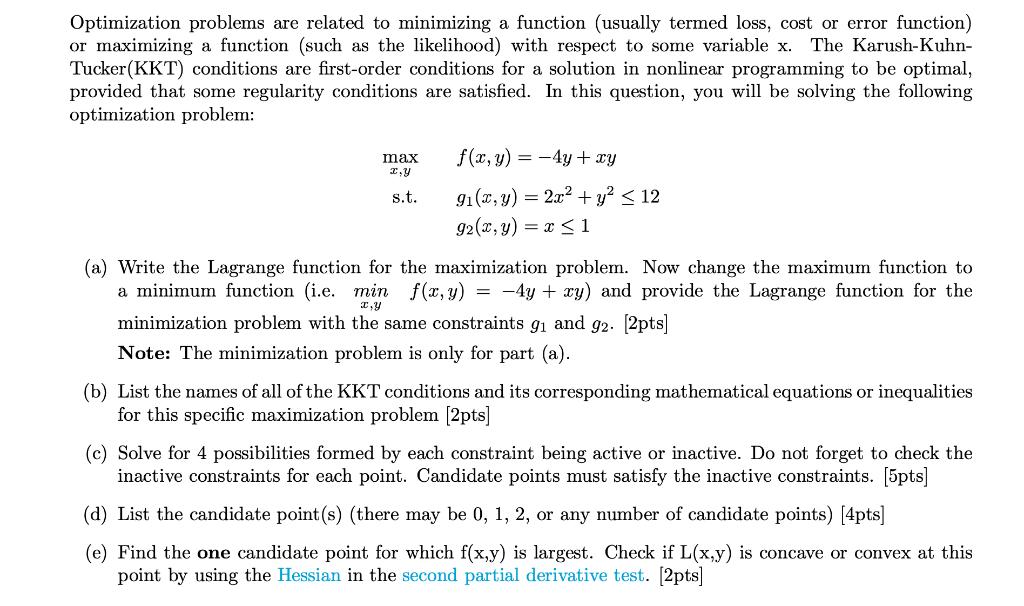
Optimization problems are related to minimizing a function (usually termed loss, cost or error function) or maximizing a function (such as the likelihood) with respect to some variable x. The Karush-Kuhn- Tucker(KKT) conditions are first-order conditions for a solution in nonlinear programming to be optimal, provided that some regularity conditions are satisfied. In this question, you will be solving the following optimization problem: max x,y s.t. f(x, y)=-4y + xy 91(x, y) = 2x + y 12 92(x, y) = x 1 (a) Write the Lagrange function for the maximization problem. Now change the maximum function to a minimum function (i.e. min f(x,y) = 4y + xy) and provide the Lagrange function for the x,y minimization problem with the same constraints 9 and 92. [2pts] Note: The minimization problem is only for part (a). (b) List the names of all of the KKT conditions and its corresponding mathematical equations or inequalities for this specific maximization problem [2pts] (c) Solve for 4 possibilities formed by each constraint being active or inactive. Do not forget to check the inactive constraints for each point. Candidate points must satisfy the inactive constraints. [5pts] (d) List the candidate point (s) (there may be 0, 1, 2, or any number of candidate points) [4pts] (e) Find the one candidate point for which f(x,y) is largest. Check if L(x,y) is concave or convex at this point by using the Hessian in the second partial derivative test. [2pts]