Part 1  Differences between two means (T-test)

‘Differences in sleep and income by sex’ Group Statistics
	Sex	N	Mean	Std. Deviation	Std. Error Mean
Weight in pounds	Male	170	168.12	24.210	1.857
	Female	136	129.51	18.429	1.580
Average hours of sleep per night	Male	170	7.653	1.5981	.1226
	Female	136	8.281	1.8569	.1592
Household income	Male	170	39403.24	19235.298	1475.280
	Female	136	41150.75	19850.689	1702.183

Independent Samples Effect Sizes
		Standardizera	Point Estimate	95% Confidence Interval
				Lower	Upper
Weight in pounds	Cohen's d	21.832	1.769	1.502	2.033
	Hedges' correction	21.886	1.764	1.498	2.028
	Glass's delta	18.429	2.095	1.757	2.430
Average hours of sleep per night	Cohen's d	1.7178	-.366	-.593	-.138
	Hedges' correction	1.7221	-.365	-.591	-.138
	Glass's delta	1.8569	-.338	-.567	-.109
Household income	Cohen's d	19510.976	-.090	-.315	.136
	Hedges' correction	19559.277	-.089	-.314	.136
	Glass's delta	19850.689	-.088	-.314	.138
a. The denominator used in estimating the effect sizes.  Cohen's d uses the pooled standard deviation.  Hedges' correction uses the pooled standard deviation, plus a correction factor.  Glass's delta uses the sample standard deviation of the control group. Part 1 Differences between two means Review your data output from your T-tests.  1. If we use t-tests to assess a significant difference between two means, why do we have consider the variance of the two group scores as part of this process? (1 mark) 2. Did you violate assumptions of variance with any of the t-tests? Explain. (2 marks) 3. What would be the statistical conclusion from the analyses for both sleep, income and weight? Ensure that in your conclusion you express your t-scores from your data (see Powerpoint and T-test Practice assignment for how to express t-values for such write-ups) (2 marks) 4. You ran three t-tests in this experiment. Why is this not advised? (1 mark) 5. Which type of t-test (dependent or independent) will always have less pooled variance associated with it? Why? (2 marks) 6. Why does the t-distribution use standard error instead of the standard deviation/z-scores? (2 marks)

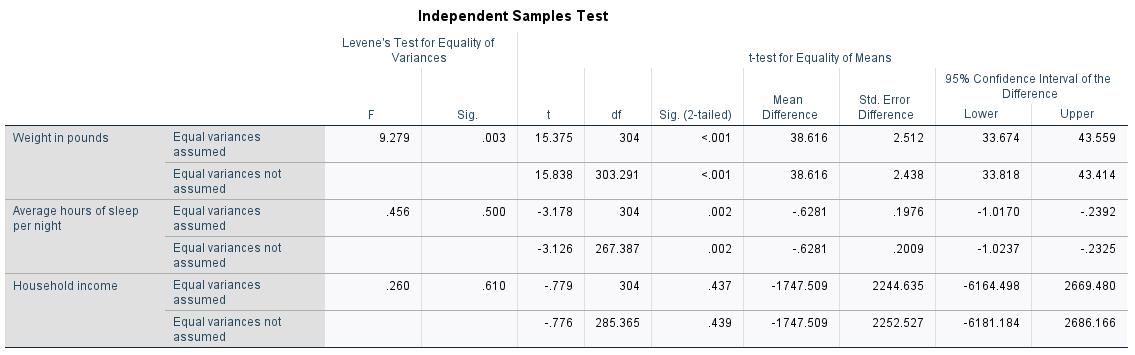
Weight in pounds Average hours of sleep per night Household income. Equal variances assumed Equal variances not assumed Equal variances assumed Equal variances not assumed. Equal variances assumed Equal variances not. assumed. Levene's Test for Equality of Variances Sig. F Independent Samples Test t df 304 15.375 15.838 303.291 -3.178 304 -3.126 267.387 -.779 304 -.776 285.365 9.279 .456 .260 .003 .500 .610 Sig. (2-tailed)