Question
Part [1] The first shape is a torus, a donut shape. Let a be the distance from the center of the overall shape to center
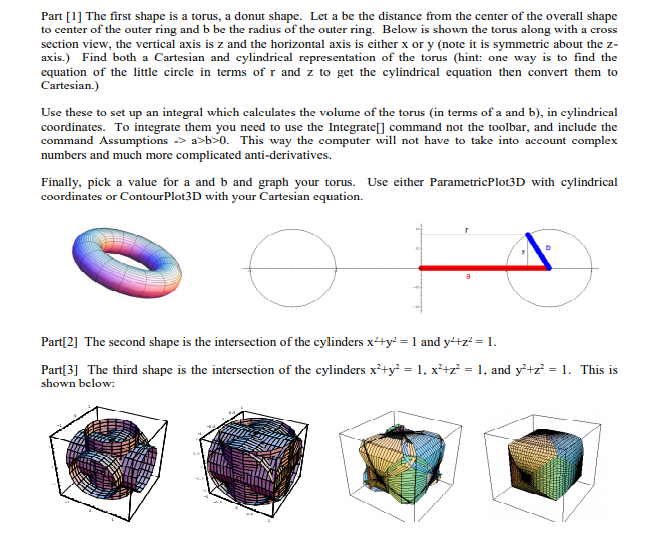
Part [1] The first shape is a torus, a donut shape. Let a be the distance from the center of the overall shape to center of the outer ring and b be the radius of the outer ring. Below is shown the torus along with a cross section view, the vertical axis is z and the horizontal axis is either x or y (note it is symmetric about the zaxis.) Find both a Cartesian and cylindrical representation of the torus (hint: one way is to find the equation of the little circle in terms of r and z to get the cylindrical equation then convert them to Cartesian.)
Use these to set up an integral which calculates the volume of the torus (in terms of a and b), in cylindrical coordinates. To integrate them you need to use the Integrate[] command not the toolbar, and include the command Assumptions -> a>b>0. This way the computer will not have to take into account complex numbers and much more complicated anti-derivatives.
Finally, pick a value for a and b and graph your torus. Use either ParametricPlot3D with cylindrical coordinates or ContourPlot3D with your Cartesian equation.
Part[2] The second shape is the intersection of the cylinders x2+y2 = 1 and y2+z2 = 1.
Part[3] The third shape is the intersection of the cylinders x2+y2 = 1, x2+z2 = 1, and y2+z2 = 1. This is shown below:
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


