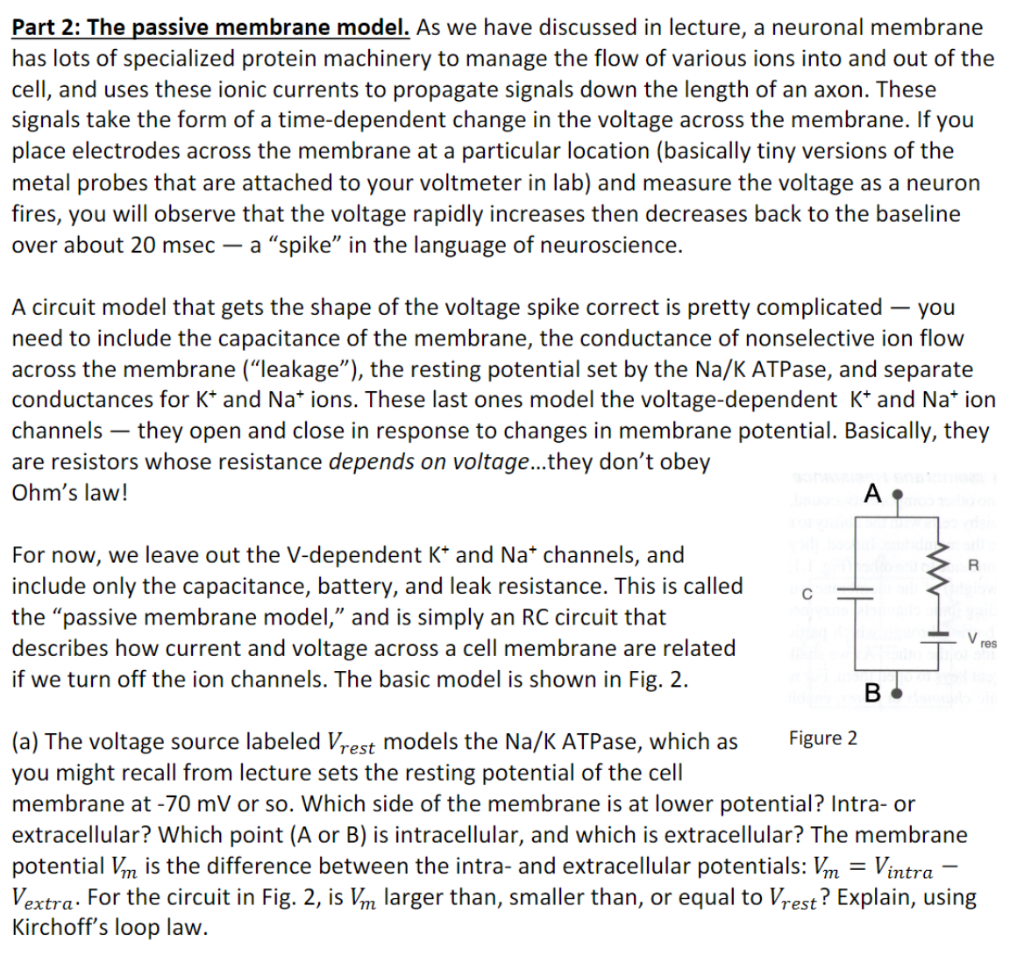
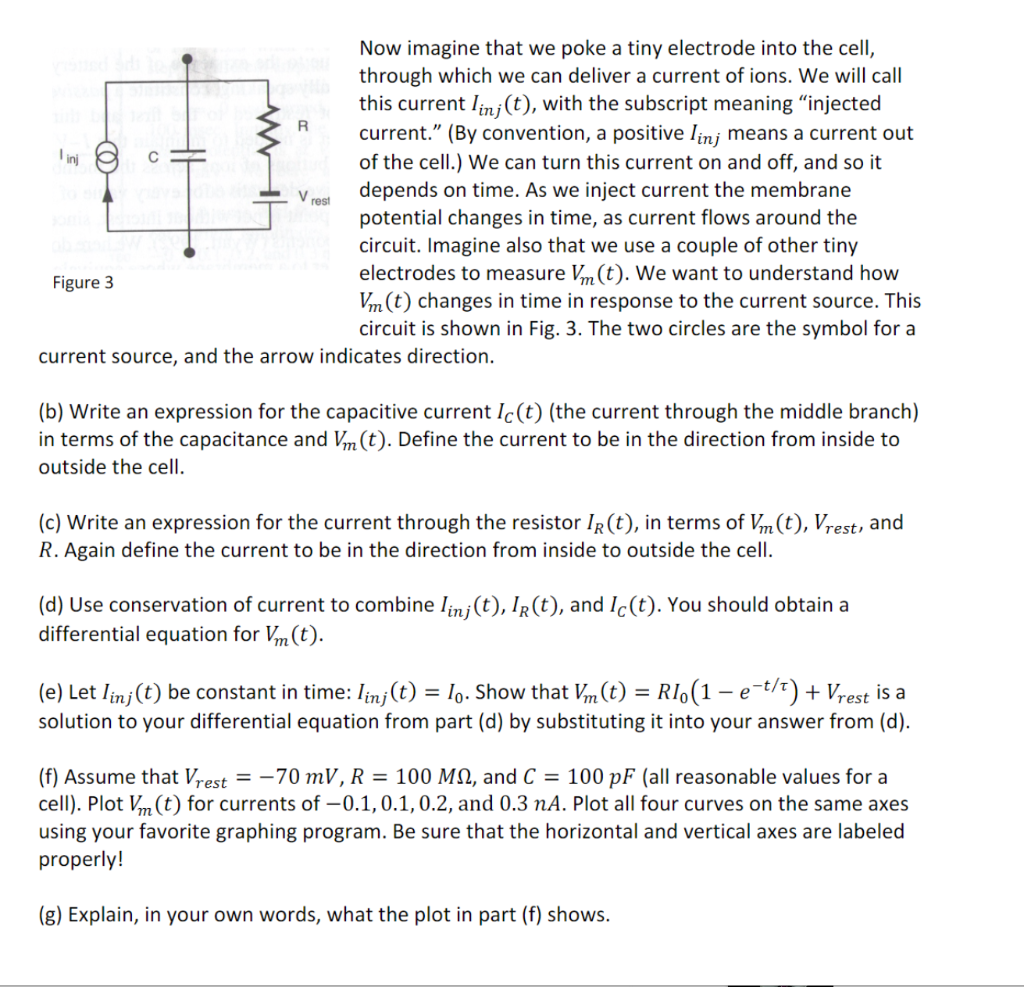
Part 2: The passive membrane model. As we have discussed in lecture, a neuronal membrane has lots of specialized protein machinery to manage the flow of various ions into and out of the cell, and uses these ionic currents to propagate signals down the length of an axon. These signals take the form of a time-dependent change in the voltage across the membrane. If you place electrodes across the membrane at a particular location (basically tiny versions of the metal probes that are attached to your voltmeter in lab) and measure the voltage as a neuron fires, you will observe that the voltage rapidly increases then decreases back to the baseline over about 20 msec - a "spike" in the language of neuroscience. A circuit model that gets the shape of the voltage spike correct is pretty complicated - you need to include the capacitance of the membrane, the conductance of nonselective ion flow across the membrane ("leakage"), the resting potential set by the Na/K ATPase, and separate conductances for K+and Na+ions. These last ones model the voltage-dependent K+and Na+ion channels - they open and close in response to changes in membrane potential. Basically, they are resistors whose resistance depends on voltage...they don't obey Ohm's law! For now, we leave out the V-dependent K+and Na+channels, and include only the capacitance, battery, and leak resistance. This is called the "passive membrane model," and is simply an RC circuit that describes how current and voltage across a cell membrane are related if we turn off the ion channels. The basic model is shown in Fig. 2. (a) The voltage source labeled Vrest models the Na/K ATPase, which as Figure 2 you might recall from lecture sets the resting potential of the cell membrane at 70mV or so. Which side of the membrane is at lower potential? Intra- or extracellular? Which point (A or B) is intracellular, and which is extracellular? The membrane potential Vm is the difference between the intra- and extracellular potentials: Vm=Vintra Vextra. For the circuit in Fig. 2, is Vm larger than, smaller than, or equal to Vrest ? Explain, using Kirchoff's loop law. Now imagine that we poke a tiny electrode into the cell, through which we can deliver a current of ions. We will call this current Iinj(t), with the subscript meaning "injected current." (By convention, a positive Iinj means a current out of the cell.) We can turn this current on and off, and so it depends on time. As we inject current the membrane potential changes in time, as current flows around the circuit. Imagine also that we use a couple of other tiny electrodes to measure Vm(t). We want to understand how Vm(t) changes in time in response to the current source. This circuit is shown in Fig. 3. The two circles are the symbol for a current source, and the arrow indicates direction. (b) Write an expression for the capacitive current IC(t) (the current through the middle branch) in terms of the capacitance and Vm(t). Define the current to be in the direction from inside to outside the cell. (c) Write an expression for the current through the resistor IR(t), in terms of Vm(t),Vrest, and R. Again define the current to be in the direction from inside to outside the cell. (d) Use conservation of current to combine Iinj(t),IR(t), and IC(t). You should obtain a differential equation for Vm(t) (e) Let Iinj(t) be constant in time: Iinj(t)=I0. Show that Vm(t)=RI0(1et/)+Vrest is a solution to your differential equation from part (d) by substituting it into your answer from (d). (f) Assume that Vrest=70mV,R=100M, and C=100pF (all reasonable values for a cell). Plot Vm(t) for currents of 0.1,0.1,0.2, and 0.3nA. Plot all four curves on the same axes using your favorite graphing program. Be sure that the horizontal and vertical axes are labeled properly! (g) Explain, in your own words, what the plot in part (f) shows. Part 2: The passive membrane model. As we have discussed in lecture, a neuronal membrane has lots of specialized protein machinery to manage the flow of various ions into and out of the cell, and uses these ionic currents to propagate signals down the length of an axon. These signals take the form of a time-dependent change in the voltage across the membrane. If you place electrodes across the membrane at a particular location (basically tiny versions of the metal probes that are attached to your voltmeter in lab) and measure the voltage as a neuron fires, you will observe that the voltage rapidly increases then decreases back to the baseline over about 20 msec - a "spike" in the language of neuroscience. A circuit model that gets the shape of the voltage spike correct is pretty complicated - you need to include the capacitance of the membrane, the conductance of nonselective ion flow across the membrane ("leakage"), the resting potential set by the Na/K ATPase, and separate conductances for K+and Na+ions. These last ones model the voltage-dependent K+and Na+ion channels - they open and close in response to changes in membrane potential. Basically, they are resistors whose resistance depends on voltage...they don't obey Ohm's law! For now, we leave out the V-dependent K+and Na+channels, and include only the capacitance, battery, and leak resistance. This is called the "passive membrane model," and is simply an RC circuit that describes how current and voltage across a cell membrane are related if we turn off the ion channels. The basic model is shown in Fig. 2. (a) The voltage source labeled Vrest models the Na/K ATPase, which as Figure 2 you might recall from lecture sets the resting potential of the cell membrane at 70mV or so. Which side of the membrane is at lower potential? Intra- or extracellular? Which point (A or B) is intracellular, and which is extracellular? The membrane potential Vm is the difference between the intra- and extracellular potentials: Vm=Vintra Vextra. For the circuit in Fig. 2, is Vm larger than, smaller than, or equal to Vrest ? Explain, using Kirchoff's loop law. Now imagine that we poke a tiny electrode into the cell, through which we can deliver a current of ions. We will call this current Iinj(t), with the subscript meaning "injected current." (By convention, a positive Iinj means a current out of the cell.) We can turn this current on and off, and so it depends on time. As we inject current the membrane potential changes in time, as current flows around the circuit. Imagine also that we use a couple of other tiny electrodes to measure Vm(t). We want to understand how Vm(t) changes in time in response to the current source. This circuit is shown in Fig. 3. The two circles are the symbol for a current source, and the arrow indicates direction. (b) Write an expression for the capacitive current IC(t) (the current through the middle branch) in terms of the capacitance and Vm(t). Define the current to be in the direction from inside to outside the cell. (c) Write an expression for the current through the resistor IR(t), in terms of Vm(t),Vrest, and R. Again define the current to be in the direction from inside to outside the cell. (d) Use conservation of current to combine Iinj(t),IR(t), and IC(t). You should obtain a differential equation for Vm(t) (e) Let Iinj(t) be constant in time: Iinj(t)=I0. Show that Vm(t)=RI0(1et/)+Vrest is a solution to your differential equation from part (d) by substituting it into your answer from (d). (f) Assume that Vrest=70mV,R=100M, and C=100pF (all reasonable values for a cell). Plot Vm(t) for currents of 0.1,0.1,0.2, and 0.3nA. Plot all four curves on the same axes using your favorite graphing program. Be sure that the horizontal and vertical axes are labeled properly! (g) Explain, in your own words, what the plot in part (f) shows