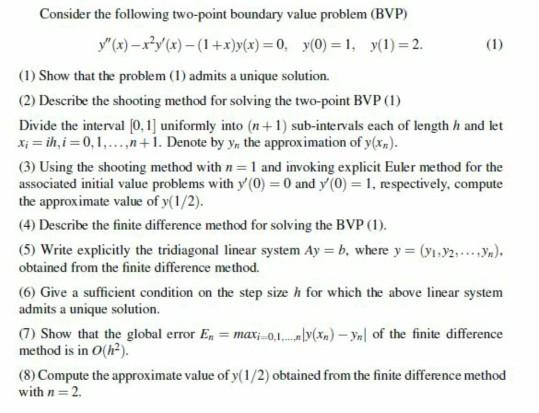
part 3,5,6,7 and 8

(1) Consider the following two-point boundary value problem (BVP) y"(x) x?y'(x) - (1+x)y(x) = 0; y(0)=1, y(1)=2. (1) Show that the problem (1) admits a unique solution. (2) Describe the shooting method for solving the two-point BVP (1) Divide the interval (0.1) uniformly into (n+1) sub-intervals each of length h and let xi =ih,i=0,1,...,n+1. Denote by Yn the approximation of y(x). (3) Using the shooting method with n=1 and invoking explicit Euler method for the associated initial value problems with y0) = 0 and y(0) = 1, respectively, compute the approximate value of y(1/2). (4) Describe the finite difference method for solving the BVP (1). (5) Write explicitly the tridiagonal linear system Ay = b. where y = (91,92, ....). obtained from the finite difference method. (6) Give a sufficient condition on the step size h for which the above linear system admits a unique solution. (7) Show that the global error En = max; -0,1..- (n) Yol of the finite difference method is in 0(h (8) Compute the approximate value of y(1/2) obtained from the finite difference method with n=2. (1) Consider the following two-point boundary value problem (BVP) y"(x) x?y'(x) - (1+x)y(x) = 0; y(0)=1, y(1)=2. (1) Show that the problem (1) admits a unique solution. (2) Describe the shooting method for solving the two-point BVP (1) Divide the interval (0.1) uniformly into (n+1) sub-intervals each of length h and let xi =ih,i=0,1,...,n+1. Denote by Yn the approximation of y(x). (3) Using the shooting method with n=1 and invoking explicit Euler method for the associated initial value problems with y0) = 0 and y(0) = 1, respectively, compute the approximate value of y(1/2). (4) Describe the finite difference method for solving the BVP (1). (5) Write explicitly the tridiagonal linear system Ay = b. where y = (91,92, ....). obtained from the finite difference method. (6) Give a sufficient condition on the step size h for which the above linear system admits a unique solution. (7) Show that the global error En = max; -0,1..- (n) Yol of the finite difference method is in 0(h (8) Compute the approximate value of y(1/2) obtained from the finite difference method with n=2