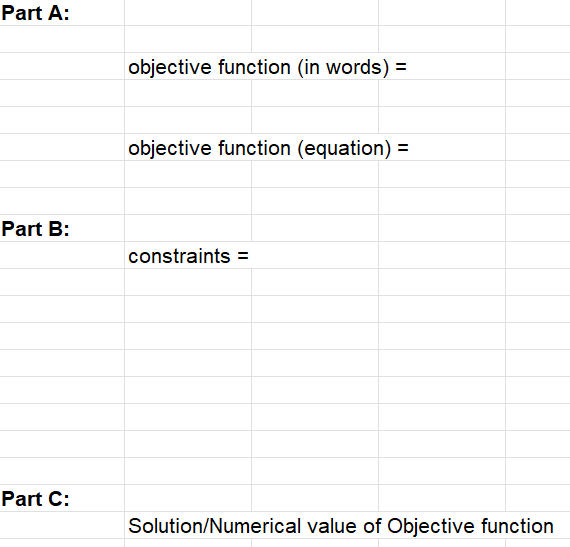
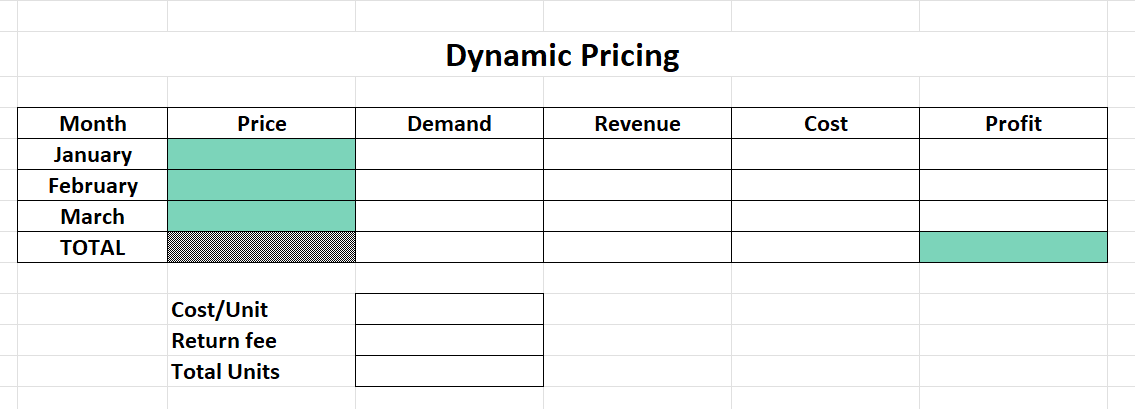Part A: objective function (in words) = objective function (equation) = Part B: constraints = Part C: Solution/Numerical value of Objective function Dynamic Pricing \begin{tabular}{|c|l|l|l|l|l|} \hline Month & Price & Demand & Revenue & Cost & Profit \\ \hline January & & & & & \\ \hline February & & & & & \\ \hline March & & & & & \\ \hline TOTAL & & & & & \\ \hline \end{tabular} Cost/Unit Return fee Total Units \begin{tabular}{|l|} \hline \\ \hline \\ \hline \end{tabular} Stevens Airlines is considering air service from Hoboken to Paris and Hoboken to LA. However, Stevens Airlines has one gate in Hoboken from which it can only operate 12 hours a day to complete any services. Each flight requires one hour of gate time. Each flight to Paris consumes 15 hours of pilot crew time and is expected to produce a profit of $2500. Each flight to LA uses 10 hours of pilot crew time per flight and will result in a profit of $2000 per flight. Pilot and crew labor is limited to 150 hours per day. The market for service to Paris is limited to a max of 9 flights a day. A. Identify the objective function and create a formula to represent it. Use x1 to represent number of flights per day to Paris, and x2 to represent number of flights per day to LA. B. List the constraint equations. C. Use the elimination method to solve the constraint equations and then determine the solution to part A. (Hint: to solve the problem assume that all constraint equations use equal signs instead of signs) Part II: You just performed very simple linear programing to determine the optimal solution. Now given the below demand equations, solve the following problem. .... A retailer has purchased 725 ski parkas before the start of the winter season. The season lasts three months, and the retailer has forecast demand in each of the three months to be Januarydemand=6250.97JanuaryPriceFebruarydemand=6251.05FebruaryPriceMarchdemand=6251.15MarchPrice The retailer has paid $108 each for each parka but will return the unsold ones for $73 each (there is a $35 return fee for each unsold parka). Hint: Consider the unsold parkas x35 in the Total Cost Hint 2: Total Profit = Total Revenue - Total Cost Run a dynamic pricing models (price for each month may vary) for this problem. Determine which price to charge for each of the three months, in order to maximize profit