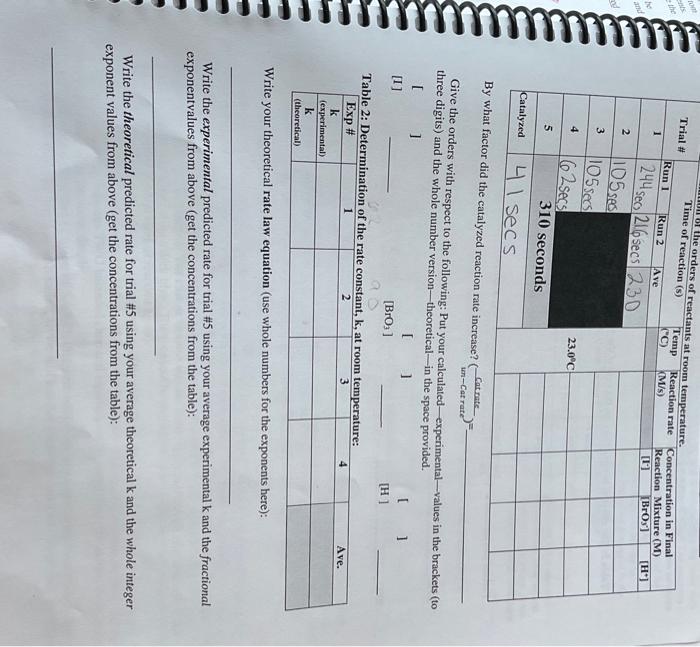
Part A: Reaction Orders and Rate Law - The reaction will start the instant that the two solutions ( A and B - each prepared in a separate test tube) are mixed together. - Be careful when measuring out the reagents for this lab; even though we are using 10.0mL graduated cylinders to measure out reagents - not the most precise instrument - the volumes being measured equal the molarity of the reagents, and therefore, they DO have an effect on the rate. Remember, the rate we are measuring is dependent on the concentration of reagents, and therefore to volume of reagents added crucial to reprolume of the solutions will affect the rate of reaction. Careful dispensation of reagents is - The following table shows the quantities of reagents used in preparing the two solutions which when mixed (A\&B) make the reaction mixture. The mixing of KBrO3 (in solution B) with the KI (in solution A) will start the reaction; therefore, measure the reagents in separate 10.0mL graduated cylinders and then put the separate solutions into separate test tubes until you are ready to start the reaction. The reaction, and the stopwatch, will start when the two test tubes are mixed together. 1. You will need approximately 20.0ml MAX for each of the four reagents in this lab. Get and clean 5 small (50-100 mL) beakers (make sure you label which beaker is which), dry them and then put approximately 20mL or each reagent into the properly labeled beaker (the 5h beaker is for D.I water used to maintain constant final volume), you can get that from the tap with the white handle. Also use cleaned transfer pipettes (these are the plastic pipettes) for each beaker - do not mix these up, keep the same transfer pipette in the same beaker throughout the entire lab!! THIS IS CRITICAL. 2. Because there are two solutions that when mixed will start the reaction, get and clean TWO 10mL. graduated cylinders - one will be used exclusively for preparation of solution A and the other will be used exclusively for the preparation of solution B. DO NOT GET THESE GRADUATED CYLINDERS MIXED UP. If you prepare solution A in the graduated cylinder that was used for solution B the previous time, it will result in very poor data. Also, DO NOT MIX A AND B IN THE GRADUATED CYLINDERS that would contaminate both and ruin the experiment. 3. Get and clean 4 test tubes. Since there are two runs for the first trial, you can prepare both sets of solutions at the same time-DO NOT TRY TO TIME BOTH RUNS AT THE SAME TIME for these runs at room temperature, they are not so long as to be a problem. Each nun is short in time (under 5 minutes), and if you try to do both at the same time you will not be able to get as precise a measurement of the time. Measure out two sets of the solutions (A&B) required for Trial 1. DO this by adding each reagent to the appropriate graduated cylinder using a transfer pipette-DO NOT USE THE MARKING ON THE TRANSFER PIPET - THEY ARE NOT ACCURATE AT ALL!!! In graduated cylinder A prepare solution A by putting in 2.0mL of KI using a transfer pipette exclusively for KI. Be careful not to go over the 2.0mL mark, and make sure you get the bottom of the meniscus "right" on top of the 2.0 mL line with the graduated cylinder at eye level. Then (without going over) put 2.0mL of Na2S2O3 into the cylinder on top of the KI solution, again being careful not to go over, and getting the bottom of the meniscus "right" on the 4.0mL line - that is the 2.0mL of KI+2.0mL of Na2S2O3 make a total of 4.0 mL. DO NOT, and this is very, very important, DO NOT TRY TO SUCK OUT ANY EXCESS Using your experimentally predicred rate for trial is, calculate the percent emor between your experinental predictco. rate and the experimentally determined rate from the instructor provided info: Table 3: The effect of where VdibpenseandMreneztarethevolumeyouaddedandmolarityofthereagentsaslistedonthecontainens (and in the table): Viotal afer miving and Mufter miving are the final total volume after the two test tubes were mixed the FINAL volumes will be the same for all solution of the two test tubes-this is for what we are solving. Since used was equal to 1.00mL - each drop is about 0.05mL ) calculate that for you (the drops of stareh indicator Total volume of reaction solutions after mixing: (1.0mL starch )+(2.0mLI+)+(2.0mLS2O32)+(2.0mLH2O)+(2.0mLH+)+(2.0mL.BrOs)= 11.00mL total volume of mixtures! (NOTE THAT THIS IS mL, NOT LITERS) Now to calculate the concentration of each reagent after mixing, we use the formula: [X]=(11.000mL[volumeofreagent(mL)][concentraionofreagent(M)]) Use this to find the concentration of each reactant. Fill out the data sheet with your calculated values. NOTE: even though we used the M1V1=# moles relationship, we did not have to convert our volumes into liters here (although it is not wrong to do so), this was because we were dividing the number of moles by the same volume This can be done so fong as the units canceled out (we had 1/1000th less moles divided by 1/000th less volume). Converting "Reaction Time" to "Reaction Rate" he rate we are interested in has the form of r=(t[Bro). Why bromate? Easy, it has no coefficient in the The rate we are interested chemical equation; therefore, its rate is the rate of the reaction. However, our reaction was "timed" based on the number of moles of sodium thiosulfate (Na2S2O3) consumed during the reaction. The number of moles of sodium thiosulfate was the same for each reaction-therefore, the faster the thiosulfate moles got consumed, the faster the reaction. The change in color was the result of the appearance of iodine which reacts with the starch added and turns a dark blue. There cannot be any appreciable iodine, until all of the thiosulfate ions are consumed (look at the fast reaction again). To be able to convert the change in concentration of bromate from the moles of the timer reagent ( Na2S2O3). the balanced chemical equation needs to be used (the stoichiometric relationship between the two), Or in this case, the relationship between TWO balanced chemical equations at the same time (remember, there were two reactions going on at the same time!). First, calculate the number of moles of Na2S2O3 that were added-it was actually the thiosulfate ions ( S2O32) which is involved in the reaction with jodine, so we will use that form from now on. To do this, use the same Ley wuat ractor did the catalyzed reaction rate increase? (un-CatrateCatrate)= Give the orders with respect to the following: Put your calculated-experimental-values in the brackets (to three digits) and the whole number version-theoretical - in the space provided. [ ] [I] [BrO3] [H] Table 2: Dot Write your theoretical rate law equation (use whole numbers for the exponents here): Write the experimental predicted rate for trial \#5 using your average experimental k and the fractional exponentvalues from above (get the concentrations from the table): Write the theoretical predicted rate for trial \#5 using your average theoretical k and the whole integer exponent values from above (get the concentrations from the table): Atis late imilanicy of herriate bopis derise the count of L VRRY rraction: for thit itcond pow, The experimertal valueg vhould pioluce a very cominest vadie, based upon many trial of peaction (5) ) s. petmain the sume, the rale of the reactoon wit chanse with terperalure. Thi the restion for that irint The cxtet satre nuabor of breaure ions whe aon umed in every reaction, thy cxist same change in lorumate you got in Table 1. Part A: Reaction Orders and Rate Law - The reaction will start the instant that the two solutions ( A and B - each prepared in a separate test tube) are mixed together. - Be careful when measuring out the reagents for this lab; even though we are using 10.0mL graduated cylinders to measure out reagents - not the most precise instrument - the volumes being measured equal the molarity of the reagents, and therefore, they DO have an effect on the rate. Remember, the rate we are measuring is dependent on the concentration of reagents, and therefore to volume of reagents added crucial to reprolume of the solutions will affect the rate of reaction. Careful dispensation of reagents is - The following table shows the quantities of reagents used in preparing the two solutions which when mixed (A\&B) make the reaction mixture. The mixing of KBrO3 (in solution B) with the KI (in solution A) will start the reaction; therefore, measure the reagents in separate 10.0mL graduated cylinders and then put the separate solutions into separate test tubes until you are ready to start the reaction. The reaction, and the stopwatch, will start when the two test tubes are mixed together. 1. You will need approximately 20.0ml MAX for each of the four reagents in this lab. Get and clean 5 small (50-100 mL) beakers (make sure you label which beaker is which), dry them and then put approximately 20mL or each reagent into the properly labeled beaker (the 5h beaker is for D.I water used to maintain constant final volume), you can get that from the tap with the white handle. Also use cleaned transfer pipettes (these are the plastic pipettes) for each beaker - do not mix these up, keep the same transfer pipette in the same beaker throughout the entire lab!! THIS IS CRITICAL. 2. Because there are two solutions that when mixed will start the reaction, get and clean TWO 10mL. graduated cylinders - one will be used exclusively for preparation of solution A and the other will be used exclusively for the preparation of solution B. DO NOT GET THESE GRADUATED CYLINDERS MIXED UP. If you prepare solution A in the graduated cylinder that was used for solution B the previous time, it will result in very poor data. Also, DO NOT MIX A AND B IN THE GRADUATED CYLINDERS that would contaminate both and ruin the experiment. 3. Get and clean 4 test tubes. Since there are two runs for the first trial, you can prepare both sets of solutions at the same time-DO NOT TRY TO TIME BOTH RUNS AT THE SAME TIME for these runs at room temperature, they are not so long as to be a problem. Each nun is short in time (under 5 minutes), and if you try to do both at the same time you will not be able to get as precise a measurement of the time. Measure out two sets of the solutions (A&B) required for Trial 1. DO this by adding each reagent to the appropriate graduated cylinder using a transfer pipette-DO NOT USE THE MARKING ON THE TRANSFER PIPET - THEY ARE NOT ACCURATE AT ALL!!! In graduated cylinder A prepare solution A by putting in 2.0mL of KI using a transfer pipette exclusively for KI. Be careful not to go over the 2.0mL mark, and make sure you get the bottom of the meniscus "right" on top of the 2.0 mL line with the graduated cylinder at eye level. Then (without going over) put 2.0mL of Na2S2O3 into the cylinder on top of the KI solution, again being careful not to go over, and getting the bottom of the meniscus "right" on the 4.0mL line - that is the 2.0mL of KI+2.0mL of Na2S2O3 make a total of 4.0 mL. DO NOT, and this is very, very important, DO NOT TRY TO SUCK OUT ANY EXCESS Using your experimentally predicred rate for trial is, calculate the percent emor between your experinental predictco. rate and the experimentally determined rate from the instructor provided info: Table 3: The effect of where VdibpenseandMreneztarethevolumeyouaddedandmolarityofthereagentsaslistedonthecontainens (and in the table): Viotal afer miving and Mufter miving are the final total volume after the two test tubes were mixed the FINAL volumes will be the same for all solution of the two test tubes-this is for what we are solving. Since used was equal to 1.00mL - each drop is about 0.05mL ) calculate that for you (the drops of stareh indicator Total volume of reaction solutions after mixing: (1.0mL starch )+(2.0mLI+)+(2.0mLS2O32)+(2.0mLH2O)+(2.0mLH+)+(2.0mL.BrOs)= 11.00mL total volume of mixtures! (NOTE THAT THIS IS mL, NOT LITERS) Now to calculate the concentration of each reagent after mixing, we use the formula: [X]=(11.000mL[volumeofreagent(mL)][concentraionofreagent(M)]) Use this to find the concentration of each reactant. Fill out the data sheet with your calculated values. NOTE: even though we used the M1V1=# moles relationship, we did not have to convert our volumes into liters here (although it is not wrong to do so), this was because we were dividing the number of moles by the same volume This can be done so fong as the units canceled out (we had 1/1000th less moles divided by 1/000th less volume). Converting "Reaction Time" to "Reaction Rate" he rate we are interested in has the form of r=(t[Bro). Why bromate? Easy, it has no coefficient in the The rate we are interested chemical equation; therefore, its rate is the rate of the reaction. However, our reaction was "timed" based on the number of moles of sodium thiosulfate (Na2S2O3) consumed during the reaction. The number of moles of sodium thiosulfate was the same for each reaction-therefore, the faster the thiosulfate moles got consumed, the faster the reaction. The change in color was the result of the appearance of iodine which reacts with the starch added and turns a dark blue. There cannot be any appreciable iodine, until all of the thiosulfate ions are consumed (look at the fast reaction again). To be able to convert the change in concentration of bromate from the moles of the timer reagent ( Na2S2O3). the balanced chemical equation needs to be used (the stoichiometric relationship between the two), Or in this case, the relationship between TWO balanced chemical equations at the same time (remember, there were two reactions going on at the same time!). First, calculate the number of moles of Na2S2O3 that were added-it was actually the thiosulfate ions ( S2O32) which is involved in the reaction with jodine, so we will use that form from now on. To do this, use the same Ley wuat ractor did the catalyzed reaction rate increase? (un-CatrateCatrate)= Give the orders with respect to the following: Put your calculated-experimental-values in the brackets (to three digits) and the whole number version-theoretical - in the space provided. [ ] [I] [BrO3] [H] Table 2: Dot Write your theoretical rate law equation (use whole numbers for the exponents here): Write the experimental predicted rate for trial \#5 using your average experimental k and the fractional exponentvalues from above (get the concentrations from the table): Write the theoretical predicted rate for trial \#5 using your average theoretical k and the whole integer exponent values from above (get the concentrations from the table): Atis late imilanicy of herriate bopis derise the count of L VRRY rraction: for thit itcond pow, The experimertal valueg vhould pioluce a very cominest vadie, based upon many trial of peaction (5) ) s. petmain the sume, the rale of the reactoon wit chanse with terperalure. Thi the restion for that irint The cxtet satre nuabor of breaure ions whe aon umed in every reaction, thy cxist same change in lorumate you got in Table 1