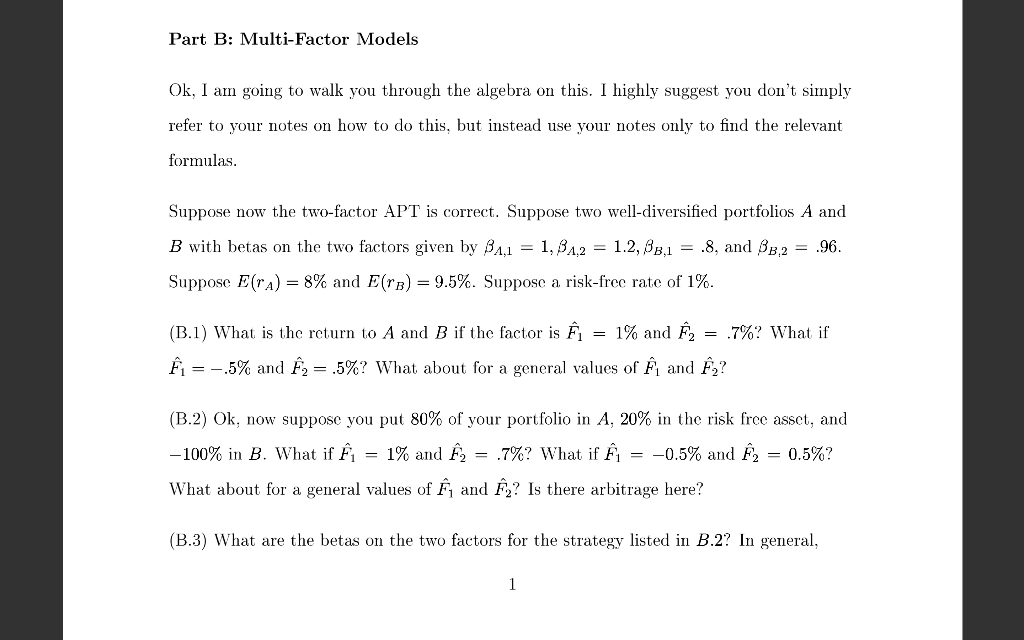
Part B: Multi-Factor Models Ok, I am going to walk you through the algebra on this. I highly suggest you don't simply refer to your notes on how to do this, but instead use your notes only to find the relevant formulas. Suppose now the two-factor APT is correct. Suppose two well-diversified portfolios A and B with betas on the two factors given by B1,1 = 1, B1,2 = 1.2, 33,1 = .8, and 31,2 = .96. Suppose E(TA) = 8% and E(TB) = 9.5%. Suppose a risk-free rate of 1%. = (B.1) What is the return to A and B if the factor is = 1% and .7%? What if = -.5% and 2 =.5%? What about for a general values of and ? (B.2) Ok, now suppose you put 80% of your portfolio in A, 20% in the risk free asset, and -100% in B. What if 1% and = .7%? What if = -0.5% and 2 = 0.5%? What about for a general values of and ? Is there arbitrage here? (B.3) What are the betas on the two factors for the strategy listed in B.2? In general, 1 if you have two well-diversified portfolios A and B, with beta values BA,1, 84,2, 33,1, and 38.2, and you invest wa in portfolio A, wo in portfolio B and the rest in the risk-free asset, what are the betas of that portfolio?. Write out ALL of your algebra steps. (B.4) Given you analysis in B.3, suppose there are two factor portfolios P, and P2, with B2,1 = 1, 32,2 = 0,38,1 = 0 and B2 = 1. Suppose you invest wi in Pi, wy in P2, and the rest in the risk free asset. What are the betas of this new portfolio? (B.5) Suppose now that the expected return to portfolio B is 10%. Suppose (from B.4) that Erp) = 6% and E(Tp) = 7%. Is there arbitrage in this situation? Part B: Multi-Factor Models Ok, I am going to walk you through the algebra on this. I highly suggest you don't simply refer to your notes on how to do this, but instead use your notes only to find the relevant formulas. Suppose now the two-factor APT is correct. Suppose two well-diversified portfolios A and B with betas on the two factors given by B1,1 = 1, B1,2 = 1.2, 33,1 = .8, and 31,2 = .96. Suppose E(TA) = 8% and E(TB) = 9.5%. Suppose a risk-free rate of 1%. = (B.1) What is the return to A and B if the factor is = 1% and .7%? What if = -.5% and 2 =.5%? What about for a general values of and ? (B.2) Ok, now suppose you put 80% of your portfolio in A, 20% in the risk free asset, and -100% in B. What if 1% and = .7%? What if = -0.5% and 2 = 0.5%? What about for a general values of and ? Is there arbitrage here? (B.3) What are the betas on the two factors for the strategy listed in B.2? In general, 1 if you have two well-diversified portfolios A and B, with beta values BA,1, 84,2, 33,1, and 38.2, and you invest wa in portfolio A, wo in portfolio B and the rest in the risk-free asset, what are the betas of that portfolio?. Write out ALL of your algebra steps. (B.4) Given you analysis in B.3, suppose there are two factor portfolios P, and P2, with B2,1 = 1, 32,2 = 0,38,1 = 0 and B2 = 1. Suppose you invest wi in Pi, wy in P2, and the rest in the risk free asset. What are the betas of this new portfolio? (B.5) Suppose now that the expected return to portfolio B is 10%. Suppose (from B.4) that Erp) = 6% and E(Tp) = 7%. Is there arbitrage in this situation