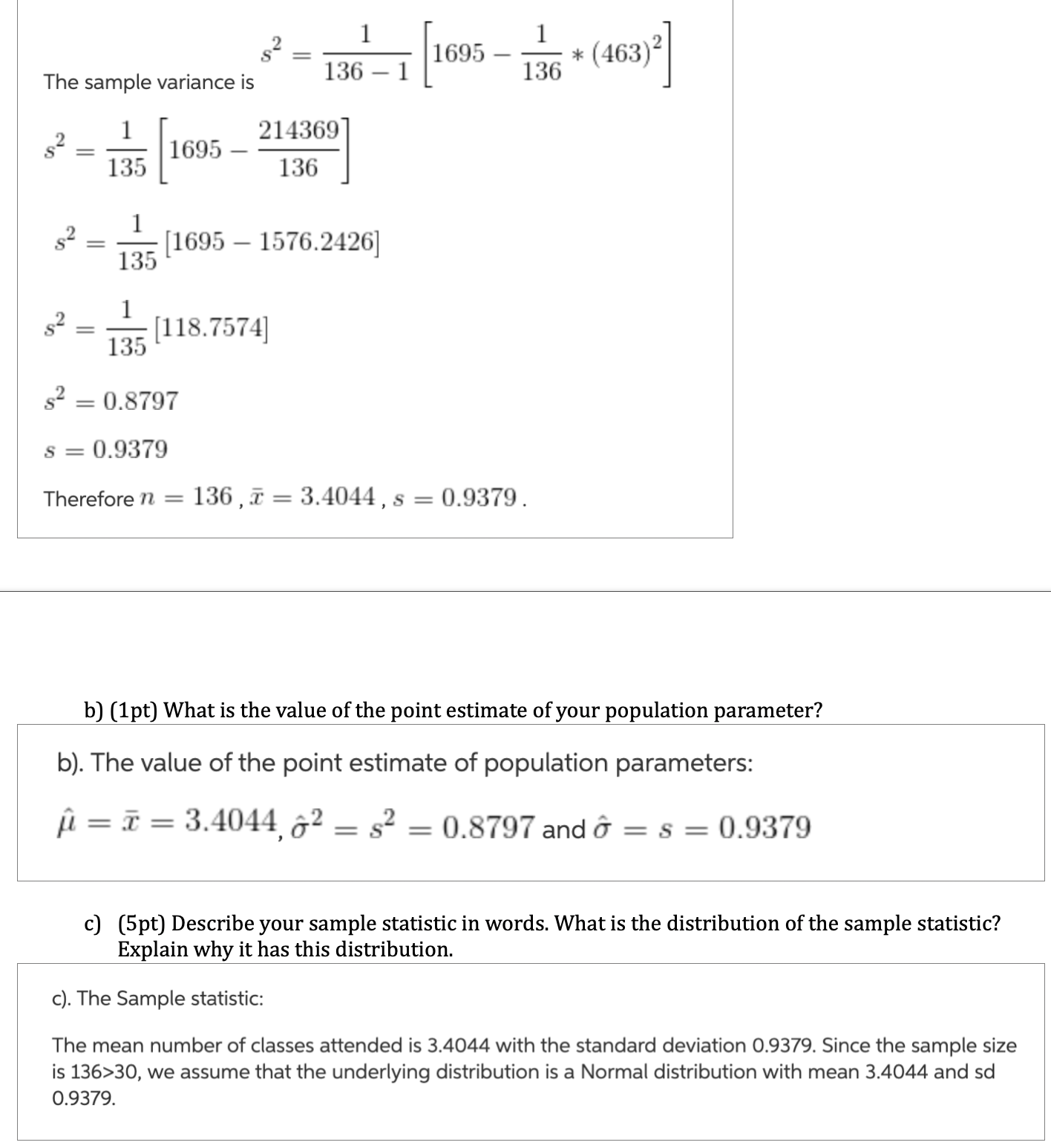
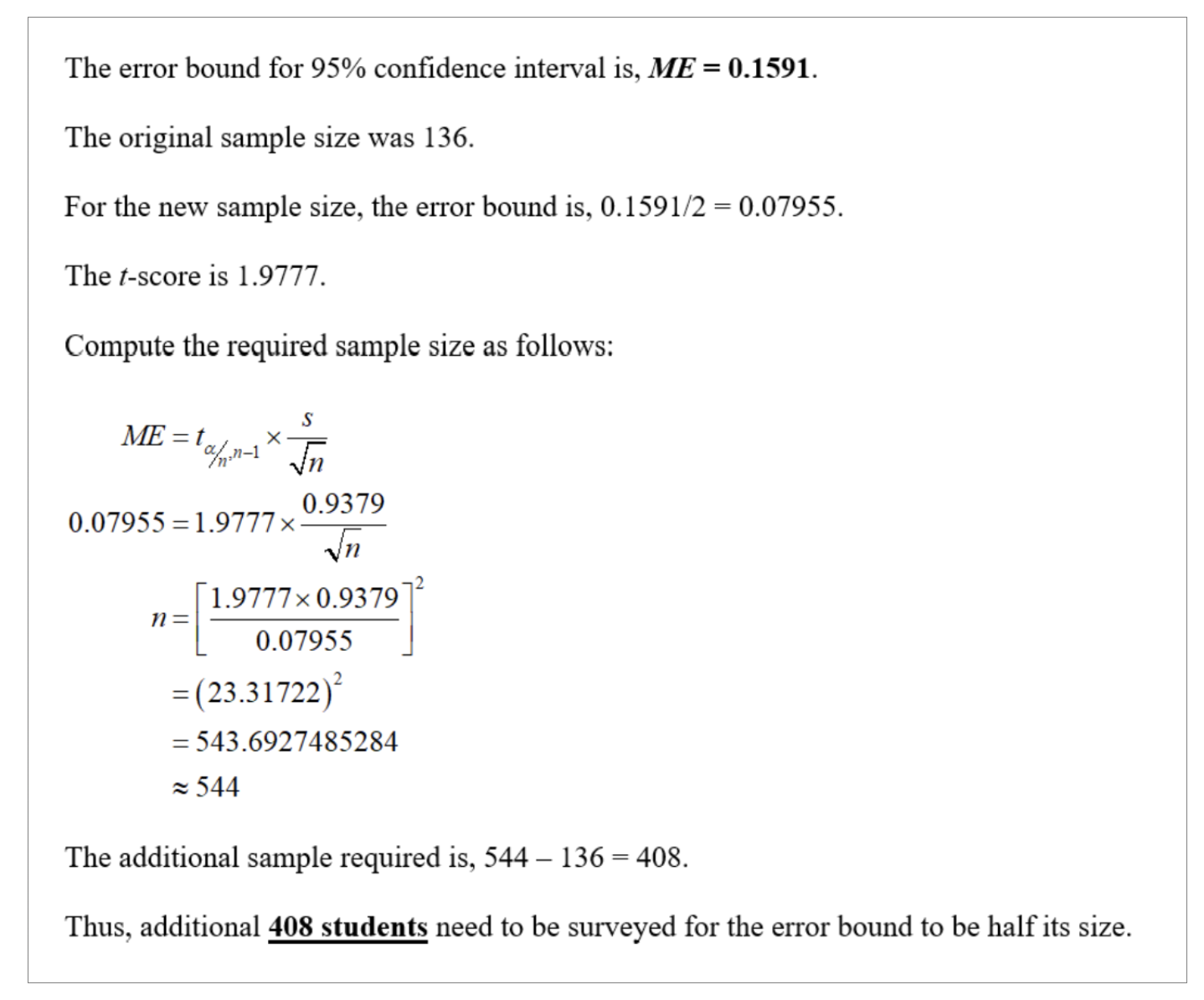
Part II: One-Population, Average Question (75 total pts) 1) Question: a) (1pt) What is the question you asked the student? How many classes are you taking this quarter? 2) Confidence Interval: a) (4pt) What are the values of the following statistics / parameters (if you know them)? How did you calculate each value (explain with formulas or specific calculator commands used)? O p, X, p,u, n, s, and/or . (Note: some of these will not be applicable to your question) a). We have the number of classes as X and the frequencies in each class as f.To calculate the sample mean, sd and the number, we use the following formulae: n = fi the mean number of classes n fi *xi = n and the sample variance is given by 1 ( fi * xi) n and the standard deviation is the square root of variance. We construct the table below for computing the statistics: * xifi fi Class Number (xi) (fi) 0 1 0 0 1 2 3 62 4 46 4924 4 4 18 36 186 558 184 736 33 5 13 6 1 36 36 Total 136 463 1695 n = fi 65 56 325 We have n = 136 The mean number of classes: 463 = = 3.4044 136 x = fi * xi n The sample variance is 136-1 [1695 - 1316 (463)] * 1 214369 = 1695 135 136 $2 = 1 == [1695 1576.2426] 135 1 135 [118.7574] = 0.8797 s = 0.9379 Therefore n = 136, 3.4044, s = 0.9379. b) (1pt) What is the value of the point estimate of your population parameter? b). The value of the point estimate of population parameters: = = 3.4044, = s = 0.8797 and = s = 0.9379 c) (5pt) Describe your sample statistic in words. What is the distribution of the sample statistic? Explain why it has this distribution. c). The Sample statistic: The mean number of classes attended is 3.4044 with the standard deviation 0.9379. Since the sample size is 136>30, we assume that the underlying distribution is a Normal distribution with mean 3.4044 and sd 0.9379. d) (4pt) For c = 0.9, 0.95, & 0.99, calculate the error bound. Show all work, using the error bound formula. S ME = t1-a/2,n1 * d). We know that the error bound is given by value of t corresponding to c=1-a. For c=0.9, 0.95,135 where t1-a/2,n-1 is the critical 1.6562 and the error bound is therefore 0.9379 ME == 1.6562* 136 ME= 1.6562 * 0.0804 = 0.1332 The confidence interval is ME = 3.4044 0.1332 = (3.2712, 3.5376). For c=0.95, we have to.975,135 = 1.9777 ME = 1.9777 * 0.9379 136 ME = 0.1591 The confidence interval is TME = 3.4044 For c=0.99, we have to.995,135 = 2.6127 == 2.6127 * 0.9379 136 0.1591 = = (3.2453, 3.5635). ME = 0.2101 The confidence interval is ME = 3.4044 0.2101 = (3.1943, 3.6145). The error bound for c=0.9 is 0.1332 and the 90% confidence interval for mean is (3.2712,3.5376) The error bound for c=0.95 is 0.1591 and the 95% confidence interval for mean is (3.2453,3.5635) The error bound for c=0.99 is 0.2101 and the 99% confidence interval for mean is (3.1943,3.6145) e) (4pt) Use the error bounds to construct a 90%, 95%, and 99% confidence interval for the population parameter. The error bound for 90% confidence interval is, ME = 0.1332. The 90% confidence interval for mean is: CI=x+ME = 3.40440.1332 = (3.2712,3.5376) The error bound for 95% confidence interval is, ME = 0.1591. The 95% confidence interval for mean is: CI = x + ME = 3.40440.1591 = (3.2453,3.5635) The error bound for 90% confidence interval is, ME = 0.2101. The 99% confidence interval for mean is: CI = x + ME = 3.40440.2101 = (3.1943,3.6145) f) (4pt) What calculator command did you use to check your work for the confidence interval? Explain why. TI-84 calculator command: STATS TESTS TInteravl Press STATS Enter the values of x, s, n and confidence level Press Calculate g) (2pt) Interpret the meaning of each confidence interval in the context of the problem. The 90% confidence interval implies that there is a 0.90 probability that the true mean number of classes attended lies in the interval (3.2712, 3.5376). The 95% confidence interval implies that there is a 0.95 probability that the true mean number of classes attended lies in the interval (3.2453, 3.5635). The 99% confidence interval implies that there is a 0.99 probability that the true mean number of classes attended lies in the interval (3.1943, 3.6145). h) (2pt) If you are interested in decreasing the error bound while keeping the confidence level constant, what will happen to the sample size? Explain. The margin of error of a confidence interval, |1%-1 1. Sample size (n) 2. Standard deviation () 3. Confidence level (1 - ) % S depends on three things, The sample size is inversely related to the error bound, i.e., as n increases ME decreases and vice-versa. So, if we want to decrease the error bound, we need to increase the sample size. i) (2pt) If you are interested in increasing the confidence level while keeping the sample size constant, what will happen to the error bound? Explain. The critical value of t increase as the confidence level increase. And the critical value is directly related to the error bound, i.e., as n increases ME also increases. So, on increasing the confidence level, keeping n constant, the error bound will increase. j) (2pt) If you are interested in increasing the sample size while keeping the error bound constant, what will happen to the confidence level? Explain. As discussed in part (h) and (i), the error bound increases when sample size is decreased or confidence level is increased. And the error bound decreases when sample size is increased or confidence level is decreased. So, if the sample size is increased, for the ME value to be constant, the confidence level has to be decreased. k) (6pt) How many additional students must you sample for the error bound to be half its size? Do this for c = 0.95. Keep t-score or z-score the same as part d. Show all steps. The error bound for 95% confidence interval is, ME = 0.1591. The original sample size was 136. For the new sample size, the error bound is, 0.1591/2 = 0.07955. The t-score is 1.9777. Compute the required sample size as follows: S ME=t an-1 n 0.9379 0.07955 1.9777x- n n = 1.9777 0.9379 0.07955 =(23.31722) = 543.6927485284 544 The additional sample required is, 544 136 = 408. - Thus, additional 408 students need to be surveyed for the error bound to be half its size.