PART4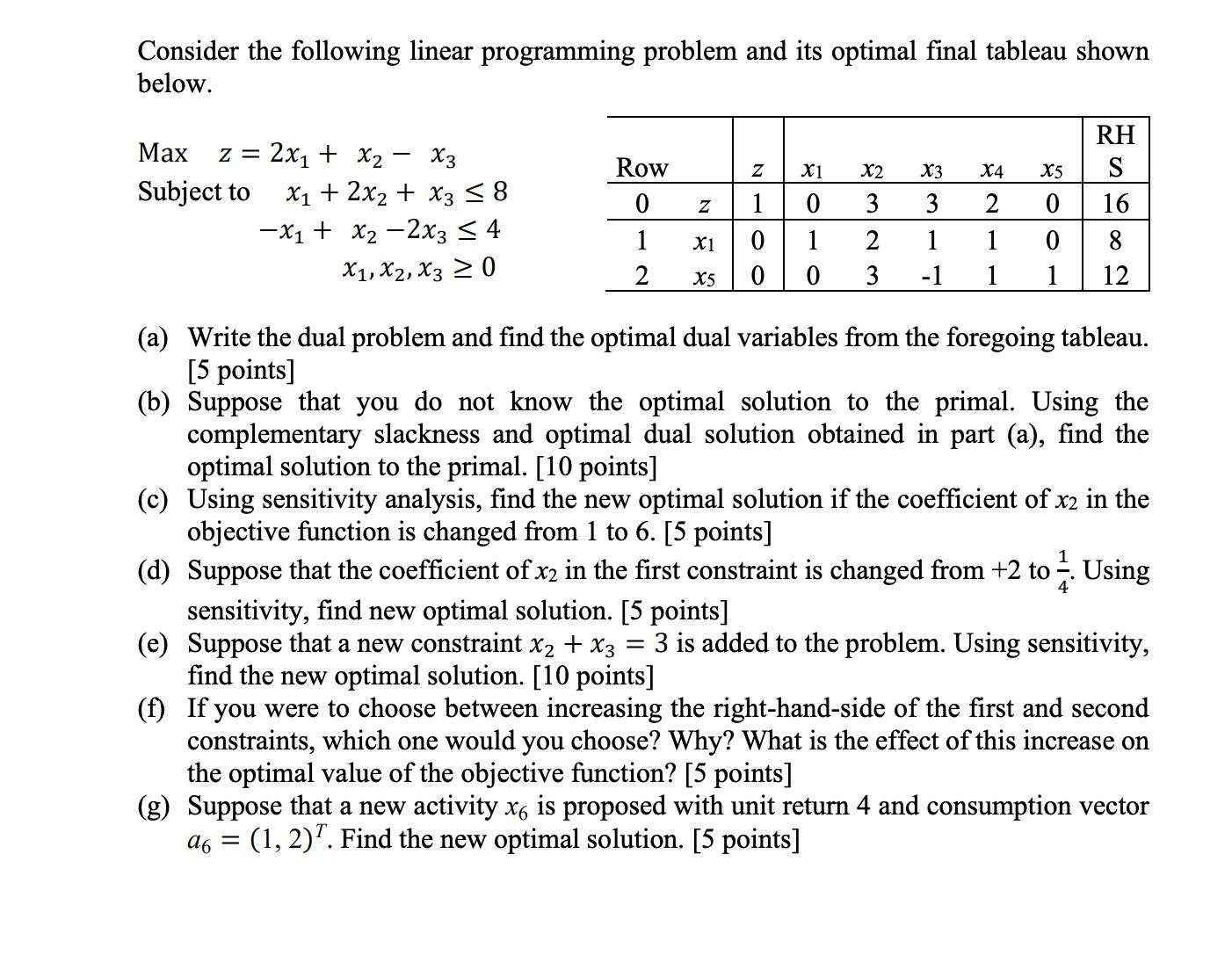

Consider the following linear programming problem and its optimal final tableau shown below. RH Max Z = 2x1 + x2 X3 Row X1 X2 X3 X4 X5 S Subject to X1 + 2x2 + x3 < 8 1 3 3 2 16 -X1 + x2 -2x3 < 4 1 1 2 1 1 8 X1 X1, X2, X3 2 0 2 3 -1 1 12 X5 (a) Write the dual problem and find the optimal dual variables from the foregoing tableau. [5 points] (b) Suppose that you do not know the optimal solution to the primal. Using the complementary slackness and optimal dual solution obtained in part (a), find the optimal solution to the primal. [10 points] (c) Using sensitivity analysis, find the new optimal solution if the coefficient of x2 in the objective function is changed from 1 to 6. [5 points] (d) Suppose that the coefficient of x2 in the first constraint is changed from +2 to -. Using sensitivity, find new optimal solution. [5 points] (e) Suppose that a new constraint x2 + x3 = 3 is added to the problem. Using sensitivity, find the new optimal solution. [10 points] (f) If you were to choose between increasing the right-hand-side of the first and second constraints, which one would you choose? Why? What is the effect of this increase on the optimal value of the objective function? [5 points] (g) Suppose that a new activity x6 is proposed with unit return 4 and consumption vector a6 = (1, 2)'. Find the new optimal solution. [5 points]