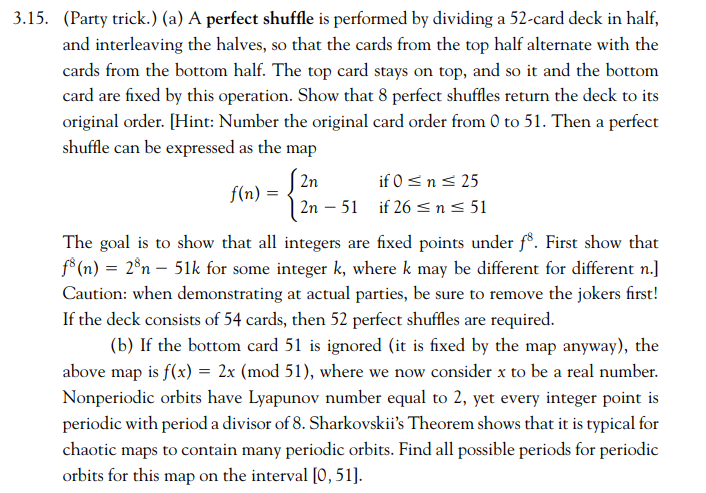
(Party trick.) (a) A perfect shuffle is performed by dividing a 52-card deck in half, and interleaving the halves, so that the cards from the top half alternate with the cards from the bottom half. The top card stays on top, and so it and the bottom card are fixed by this operation. Show that 8 perfect shuffles return the deck to its original order. [Hint: Number the original card order from 0 to 51 . Then a perfect shuffle can be expressed as the map f(n)={2n2n51if0n25if26n51 The goal is to show that all integers are fixed points under f8. First show that f8(n)=28n51k for some integer k, where k may be different for different n.] Caution: when demonstrating at actual parties, be sure to remove the jokers first! If the deck consists of 54 cards, then 52 perfect shuffles are required. (b) If the bottom card 51 is ignored (it is fixed by the map anyway), the above map is f(x)=2x(mod51), where we now consider x to be a real number. Nonperiodic orbits have Lyapunov number equal to 2, yet every integer point is periodic with period a divisor of 8 . Sharkovskii's Theorem shows that it is typical for chaotic maps to contain many periodic orbits. Find all possible periods for periodic orbits for this map on the interval [0,51]. (Party trick.) (a) A perfect shuffle is performed by dividing a 52-card deck in half, and interleaving the halves, so that the cards from the top half alternate with the cards from the bottom half. The top card stays on top, and so it and the bottom card are fixed by this operation. Show that 8 perfect shuffles return the deck to its original order. [Hint: Number the original card order from 0 to 51 . Then a perfect shuffle can be expressed as the map f(n)={2n2n51if0n25if26n51 The goal is to show that all integers are fixed points under f8. First show that f8(n)=28n51k for some integer k, where k may be different for different n.] Caution: when demonstrating at actual parties, be sure to remove the jokers first! If the deck consists of 54 cards, then 52 perfect shuffles are required. (b) If the bottom card 51 is ignored (it is fixed by the map anyway), the above map is f(x)=2x(mod51), where we now consider x to be a real number. Nonperiodic orbits have Lyapunov number equal to 2, yet every integer point is periodic with period a divisor of 8 . Sharkovskii's Theorem shows that it is typical for chaotic maps to contain many periodic orbits. Find all possible periods for periodic orbits for this map on the interval [0,51]