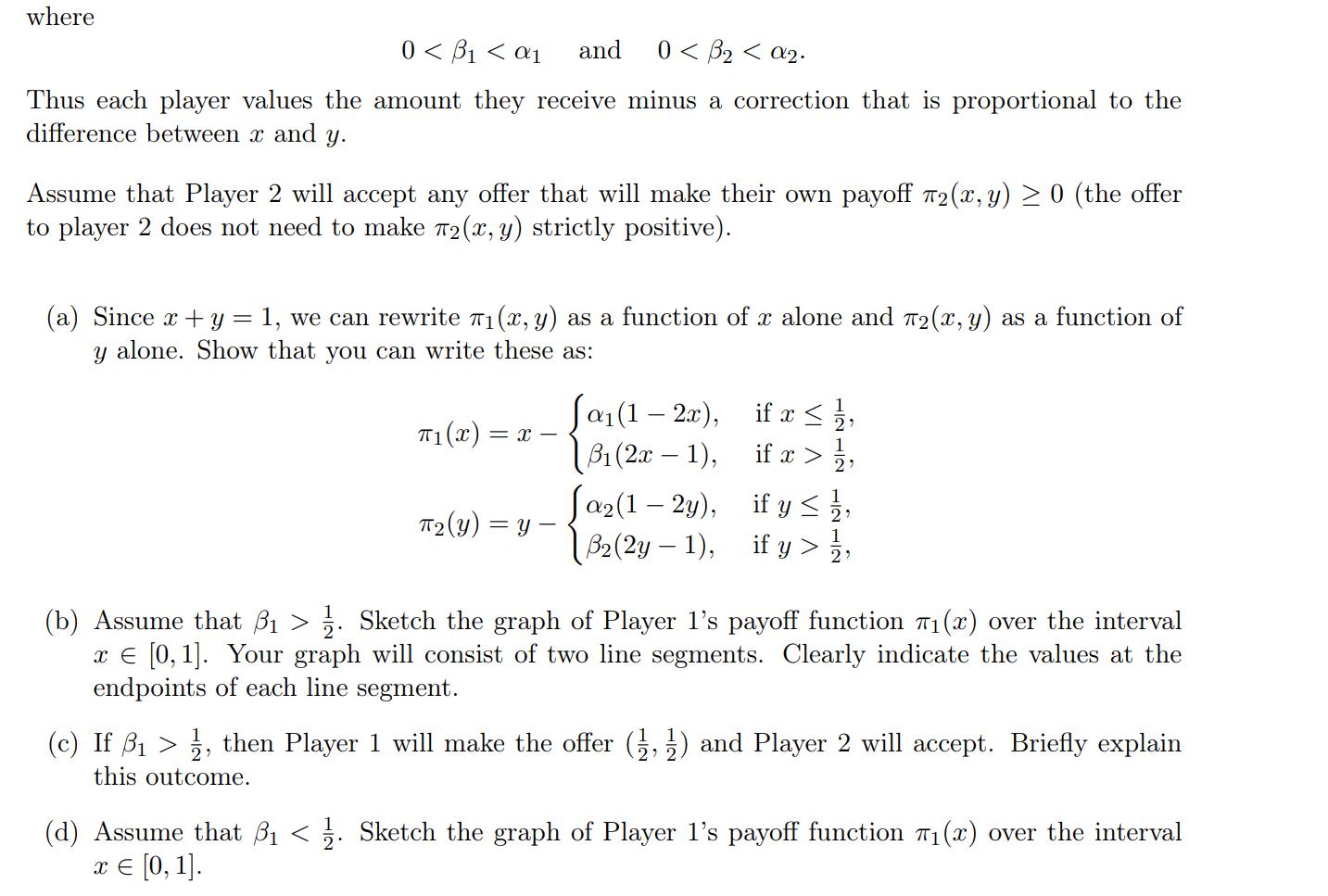
Players 1 and 2 are in an ultimatum game and will divide a particular good. Player 1 offers a division (r, y) with r and y nonnegative and + y = 1. If Player 2 accepts this offer then Player 1 will receive the fraction of the good and Player 2 will receive the fraction y. If Player 2 rejects the offer, then both players receive zero. The value r can be any real number in the interval [0, 1]. Both players are inequality averse. The players' payoffs depend on both the amount they receive and the equality of the division, but each player prefer an unequal division that favors themselves over an unequal division that favors the other player. The payoffs are as follows Jai (y-2), if x y. 1(x, y)=x- 81(x-y), if y where 0 < 1 < and 0 , 1 (b) Assume that > . Sketch the graph of Player 1's payoff function 7(x) over the interval x = [0,1]. Your graph will consist of two line segments. Clearly indicate the values at the endpoints of each line segment. (c) If B, then Player 1 will make the offer (, ) and Player 2 will accept. Briefly explain 21 this outcome. (d) Assume that < 1. Sketch the graph of Player 1's payoff function 71 (x) over the interval x = [0, 1].