please ans. all the parts. thanks!!

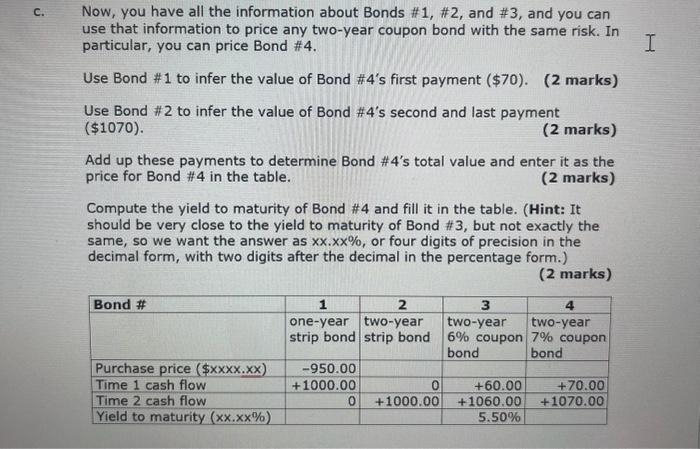
(20 marks total) Zero-coupon bonds (also called strip bonds or zeros for short) have a unique role to play in bond markets, because they only have one future cash flow. This means that you can check the price of any coupon bond (in the same risk class) by comparing the coupon bond's individual expected cash flows to zero coupons that mature on the same date. If your coupon bonds don't have the same value as the sum of all the zero-coupon bonds, there is a mispricing in the market, which presents an arbitrage opportunity for savvy bond traders. This is one application of the Law of One Price in real markets. So, here is an example (yes, a thought experiment) that shows how this works. The Law of One Price implies that financial instruments with the same risk and the same cash flows at the same time should have the same price. You are given the following incomplete information on four different bonds. Assume that all these bonds have the same risk, and that any coupon payments are paid annually. - Bond \#1: Strip bond maturing at the end of one year, with a face value of $1000, currently priced at $950, yield missing - Bond \#2: Strip bond maturing at the end of two years, with a face value of $1000, current price missing - Bond \#3: 6% coupon bond maturing at the end of two years, with a face value of $1000, with a yield to maturity of 5.5%, current price missing - Bond \#4: 7\% coupon bond maturing at the end of two years, with a face value of $1000, current price and yield missing Required: a. You should be able to use what you have learned about bond pricing in this lesson and the practice problems to calculate the price of Bond #3 and the yield to maturity of Bond #1. Fill these in in the table below, displaying the price as $xxxx,xx and the yield as xx,xx%. (4 marks) b. Next, use the information for Bond #1 and Bond #3 to determine the equilibrium price of Bond $2. Note that the first coupon payment ($60) of Bond #3 occurs at the same time as the maturity of Bond \#1, so the value of that coupon payment has to be the same, proportionately, as Bond 1. Calculate the implied value of that coupon payment. (2 marks) Next, use the information for Bond #1 and Bond #3 to determine the equilibrium price of Bond \#2. Note that the first coupon payment ($60) of Bond #3 occurs at the same time as the maturity of Bond \#1, so the value of that coupon payment has to be the same, proportionately, as Bond \#1. Calculate the implied value of that coupon payment. (2 marks) Now, by implication, the rest of Bond \#3's value, which you already know, must be entirely accounted for by the second payment ($1060) at maturity. Calculate the value of the second payment. (2 marks) Finally, note that the second payment for Bond \#3 coincides with Bond #2 's only payment, so you can infer that Bond \#2's price, proportionately, must be the same as the implied value of Bond \#3's second payment, allowing you to determine the appropriate price for Bond $2. Fill in the price of Bond #2 in the table below. (2 marks) Calculate the annual yield to maturity for Bond #2 and enter it in the table below. (2 marks) c. Now, you have all the information about Bonds #1,#2, and #3, and you can use that information to price any two-year coupon bond with the same risk. In particular, you can price Bond \#4. Use Bond \#1 to infer the value of Bond \#4's first payment ( $70 ). (2 marks) Use Bond \#2 to infer the value of Bond \#4's second and last payment ($1070). (2 marks) Add up these payments to determine Bond \#4's total value and enter it as the price for Bond \#4 in the table. (2 marks) Compute the yield to maturity of Bond #4 and fill it in the table. (Hint: It should be very close to the yield to maturity of Bond $3, but not exactly the same, so we want the answer as x.x%, or four digits of precision in the decimal form, with two digits after the decimal in the percentage form.) (2 marks) Now, you have all the information about Bonds #1,#2, and #3, and you can use that information to price any two-year coupon bond with the same risk. In particular, you can price Bond \#4. Use Bond \#1 to infer the value of Bond \#4's first payment (\$70). (2 marks) Use Bond \#2 to infer the value of Bond \#4's second and last payment ($1070). (2 marks) Add up these payments to determine Bond \#4's total value and enter it as the price for Bond \#4 in the table. ( 2 marks) Compute the yield to maturity of Bond #4 and fill it in the table. (Hint: It should be very close to the yield to maturity of Bond \#3, but not exactly the same, so we want the answer as x.x%, or four digits of precision in the decimal form, with two digits after the decimal in the percentage form.) (2 marks)