PLEASE ANSWER ALL OF THEM PROPERLY WITH ALL THE CALCULATIONS AND EXPLAINATION. 1) Vincent is deciding whether to buy a painting. The current sale price of the painting is 100 dollars. The resale price of the painting after T years is 100 + T dollars, where T is a positive integer. Ownership of the painting generates a psychological benefit of 10 dollars per year, where the benefit is received at the end of each year. Assume that the discount rate is r = 0.10. (a) Suppose that Vincent buys the painting. What is the earliest year after which he should sell the painting? [Hint: maximize the net present value with respect to the year after which he sells the painting.] (b) Suppose that Vincent sells the painting as in part (a). What is the net present value for his investment decision? (c) Should Vincent buy the painting? Explain briefly. (d) Suppose instead that Vincent cannot sell the painting after purchase. What is the internal rate of return on the investment in the painting? 2) Mason has the utility function U(C, R) = C R, where C is consumption, and R is leisure. The price of a unit of consumption is one. There are 24 hours available that can be divided between working and leisure. Let w = 1 be the wage received per hour of work. Mason has an unearned income of Y = 4.

(a) What is Mason's budget constraint? [Hint: consumption should equal labor income plus unearned income.] (b) What is the quantity of labor supplied by Mason? (c) Suppose that the government imposes a 50% tax only on labor income. What is the quantity of labor supplied by Mason?

(d) Suppose that the government imposes a 50% tax only on unearned income. What is the quantity of labor supplied by Mason? 3) Norma has the utility function u(c1, c2) = log(c1) +? log(c2), where c1 is consumption in period 1, and c2 is consumption in period 2. Norma receives an income of I = 36 in each of the two periods. She can borrow and save at an interest rate of r. The price of a unit of consumption is one in each period. (a) What is Norma's intertemporal budget constraint? [Hint: the present discounted value of consumption should equal the present discounted value of earnings.] (b) Suppose that r = 0 and ? = 1. What is Norma's consumption in each period? (c) Suppose that r = 0.5 and ? = 1. What is Norma's consumption in each period? (d) Suppose that r = 0.5 and ? = 0.25. What is Norma's consumption in each period? 4) Consider a competitive labor market with 800 identical firms. Each firm has the production function f(l) = ? l, where l denotes the amount of labor employed by the firm. The price of a unit of output is fixed at P = 1. Let w be the wage rate. The market supply of labor is given by L = 1600w. (a) What is the market demand for labor as a function of the wage rate? [Hint: the labor demand of an individual firm is derived from profit maximization.] (b) What is the equilibrium wage rate? (c) What is the elasticity of the market demand for labor with respect to the wage rate? (d) What is the economic rent earned by labor?

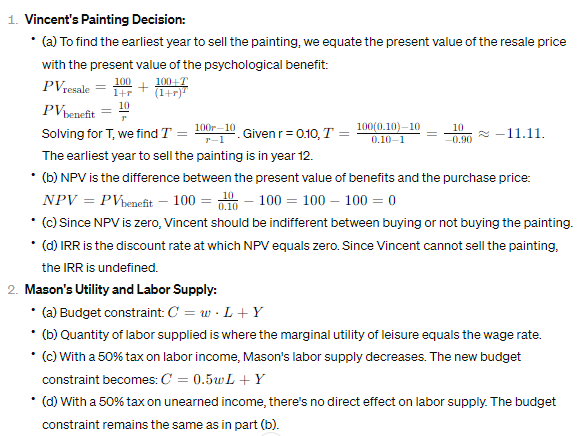
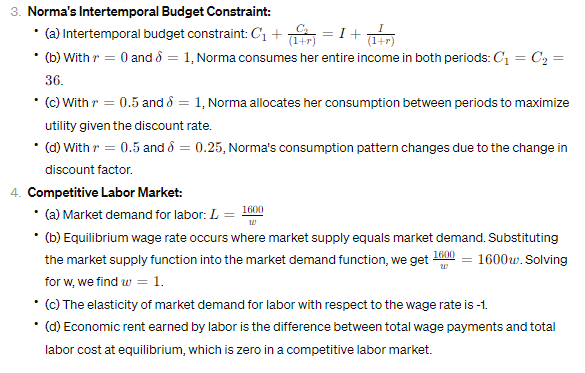1. Vincent's Painting Decision: " (a) To find the earliest year to sell the painting, we equate the present value of the resale price with the present value of the psychological benefit: PVresale = 100 + 100 T PV benefit = Solving for T, we find T' = 100r-1 . Given r = 0.10, T = 100(0.10)-10 10 0.10-1 10.90 -11.11. The earliest year to sell the painting is in year 12. " (b) NPV is the difference between the present value of benefits and the purchase price: NPV = PVbenefit - 100 = 0.10 - 100 = 100 - 100 = 0 " (c) Since NPV is zero, Vincent should be indifferent between buying or not buying the painting. (d) IRR is the discount rate at which NPV equals zero. Since Vincent cannot sell the painting, the IRR is undefined. 2. Mason's Utility and Labor Supply: " (a) Budget constraint: O' = w . L+ Y " (b) Quantity of labor supplied is where the marginal utility of leisure equals the wage rate. " (c) With a 50% tax on labor income, Mason's labor supply decreases. The new budget constraint becomes: C' = 0.5wl + Y * (d) With a 50% tax on unearned income, there's no direct effect on labor supply. The budget constraint remains the same as in part (b).3. Norma's Intertemporal Budget Constraint: " (a) Intertemporal budget constraint: C'1 + ( 1 + 7 ) = 1+ * (b) With r = 0 and 6 = 1, Norma consumes her entire income in both periods: C1 = C2 = 36. * (c) With = 0.5 and 6 = 1, Norma allocates her consumption between periods to maximize utility given the discount rate. " () With r = 0.5 and 6 = 0.25, Norma's consumption pattern changes due to the change in discount factor. 4. Competitive Labor Market: * (a) Market demand for labor: L = 1600 * (b) Equilibrium wage rate occurs where market supply equals market demand. Substituting the market supply function into the market demand function, we get To = 1600w. Solving for w, we find w = 1. "(c) The elasticity of market demand for labor with respect to the wage rate is -1. "(d) Economic rent earned by labor is the difference between total wage payments and total labor cost at equilibrium, which is zero in a competitive labor market