PLEASE ANSWER ALL PARTS and EXPLAIN! This is Game Theory. Thank You. 
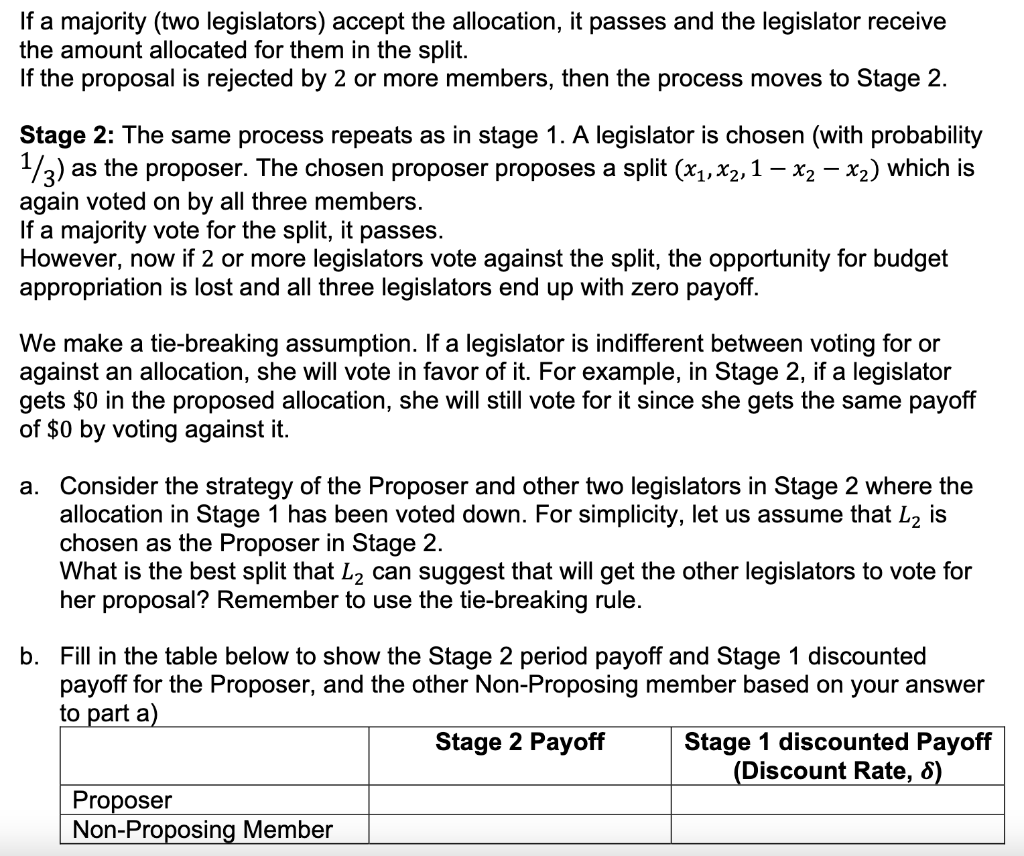

(only MA students) Legislative Bargaining: This question extends the ultimatum bargaining game to three players. The context is one where members of a legislature are deciding how to allocate the budget across districts. There are three legislators, L1, L2 and L3 who have $1 to distribute across the three districts represented by the three legislators. Each legislator wants to maximize the allocation to their own district. The discount rate is the same for all three and is given by 8 [0,1]. The sequence of decisions is as follows: Stage 1: First a legislator is chosen at random to propose a split between the three districts. Each legislator has equal probability (equal to 1/3) of being chosen as the "Proposer". Let (X1, X2,1 x2 x2) be the split proposed by the proposer, where x [0,1] is the amount given to L1, X2 [0,1 xi] is the amount given to L2 and the remainder 1 x1 X2 is given to L3. Next, the allocation (X1, X2,1 X2 x2), is voted on by all three members of the legislature simultaneously. If a majority (two legislators) accept the allocation, it passes and the legislator receive the amount allocated for them in the split. If the proposal is rejected by 2 or more members, then the process moves to Stage 2. Stage 2: The same process repeats as in stage 1. A legislator is chosen (with probability 1/3) as the proposer. The chosen proposer proposes a split (x1,x2,1 x2 xz) which is again voted on by all three members. If a majority vote for the split, it passes. However, now if 2 or more legislators vote against the split, the opportunity for budget appropriation is lost and all three legislators end up with zero payoff. We make a tie-breaking assumption. If a legislator is indifferent between voting for or against an allocation, she will vote in favor of it. For example, in Stage 2, if a legislator gets $0 in the proposed allocation, she will still vote for it since she gets the same payoff of $0 by voting against it. a. Consider the strategy of the Proposer and other two legislators in Stage 2 where the allocation in Stage 1 has been voted down. For simplicity, let us assume that L2 is chosen as the Proposer in Stage 2. What is the best split that L2 can suggest that will get the other legislators to vote for her proposal? Remember to use the tie-breaking rule. b. Fill in the table below to show the Stage 2 period payoff and Stage 1 discounted payoff for the Proposer, and the other Non-Proposing member based on your answer to part a) Stage 2 Payoff Stage 1 discounted Payoff (Discount Rate, 8) Proposer Non-Proposing Member C. Before a legislator is chosen in Stage 2 as the proposer, what is the expected discounted payoff to a legislator from rejecting the Stage 1 proposal. Remember that each legislator has a 1/3 probability of being chosen as a proposer. d. Suppose the proposer in Stage 1 is chosen to be L, given the expected discounted payoff to each legislator if the process moves to Stage 2, how should L4 split the dollar so that she maximizes her allocation while still getting a majority to vote for the proposal. e. How does the discount rate affect the allocation? (only MA students) Legislative Bargaining: This question extends the ultimatum bargaining game to three players. The context is one where members of a legislature are deciding how to allocate the budget across districts. There are three legislators, L1, L2 and L3 who have $1 to distribute across the three districts represented by the three legislators. Each legislator wants to maximize the allocation to their own district. The discount rate is the same for all three and is given by 8 [0,1]. The sequence of decisions is as follows: Stage 1: First a legislator is chosen at random to propose a split between the three districts. Each legislator has equal probability (equal to 1/3) of being chosen as the "Proposer". Let (X1, X2,1 x2 x2) be the split proposed by the proposer, where x [0,1] is the amount given to L1, X2 [0,1 xi] is the amount given to L2 and the remainder 1 x1 X2 is given to L3. Next, the allocation (X1, X2,1 X2 x2), is voted on by all three members of the legislature simultaneously. If a majority (two legislators) accept the allocation, it passes and the legislator receive the amount allocated for them in the split. If the proposal is rejected by 2 or more members, then the process moves to Stage 2. Stage 2: The same process repeats as in stage 1. A legislator is chosen (with probability 1/3) as the proposer. The chosen proposer proposes a split (x1,x2,1 x2 xz) which is again voted on by all three members. If a majority vote for the split, it passes. However, now if 2 or more legislators vote against the split, the opportunity for budget appropriation is lost and all three legislators end up with zero payoff. We make a tie-breaking assumption. If a legislator is indifferent between voting for or against an allocation, she will vote in favor of it. For example, in Stage 2, if a legislator gets $0 in the proposed allocation, she will still vote for it since she gets the same payoff of $0 by voting against it. a. Consider the strategy of the Proposer and other two legislators in Stage 2 where the allocation in Stage 1 has been voted down. For simplicity, let us assume that L2 is chosen as the Proposer in Stage 2. What is the best split that L2 can suggest that will get the other legislators to vote for her proposal? Remember to use the tie-breaking rule. b. Fill in the table below to show the Stage 2 period payoff and Stage 1 discounted payoff for the Proposer, and the other Non-Proposing member based on your answer to part a) Stage 2 Payoff Stage 1 discounted Payoff (Discount Rate, 8) Proposer Non-Proposing Member C. Before a legislator is chosen in Stage 2 as the proposer, what is the expected discounted payoff to a legislator from rejecting the Stage 1 proposal. Remember that each legislator has a 1/3 probability of being chosen as a proposer. d. Suppose the proposer in Stage 1 is chosen to be L, given the expected discounted payoff to each legislator if the process moves to Stage 2, how should L4 split the dollar so that she maximizes her allocation while still getting a majority to vote for the proposal. e. How does the discount rate affect the allocation









