Please answer all question, I completed all but I am not sure are correct.(Please give my more detail explain about Q11 Thanks!)

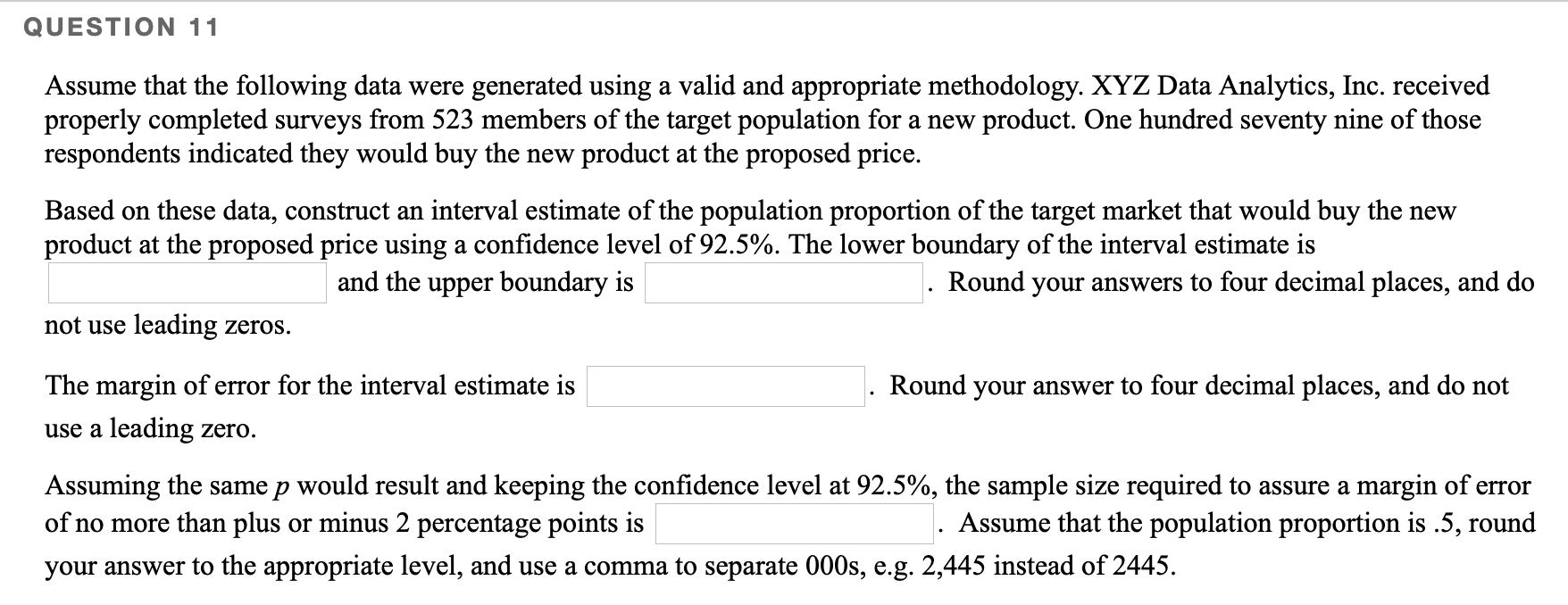
QUESTION 1 We use probability to A '\\ - " control uncertainty '\\ - " manage the uncertainty caused by random sampling methods A " - 1' create uncertainty, especially among students (Hint: this is a wrong answer) A '~ 7 1' create statistical bias A All of the choices above 'A None of the choices above QUESTION 2 Statistical bias is Il I I mev1table A " - 1' systematic ll of no consequence A the difference between the sample statistic and the population parameter A All of the choices above 'A None of the choices above QUESTION 3 A sampling distribution A 'x - 1' may be assumed to be normal if n is at least 30 A may be assumed to be normal if the underlying population is normal A is a distribution of sample statistics A is the probability distribution of all the possible values an estimator may assume when a random sample of 7: items is taken 0 All of the choices above None of the choices above QUESTION 4 Sample statistics A ' , 3 are efcient, unbiased estimators for their respective population parameters A 4 are random vanables A . . . . tend to be nearer then' respective population parameters as n increases A ' , 3 are used to make inferences about their respective populations All of the choices above A None of the choices above QUESTION 5 A sample proportion is the number of successes in n trials is often expressed as a decimal or a percentage is denoted p follows a binomial distribution All of the choices above None of the choices above QUESTION 6 The standard error of the mean is a measure of random sampling error is the standard deviation of a sampling distribution decreases as n increases is part of the calculation for the margin of error All of the choices above ONone of the choices aboveQUESTION 7 The Central Limit Theorem A tells us that all sampling distributions become approximately normal as 71 increases regardless of the shape of the underlying population A does not apply to proportions '* , 1' suggests that only measures of central tendency are important A tells us we assume a distribution is approximately normal if n is greater than 10 regardless of the shape of the underlying population A All of the choices above 'A None of the choices above QUESTION 8 In each of the two sample size equations introduced in this module, E A n n n is the maxnnum allowable margln of error A '* , 1' is always rounded up A '1 -1' must be estimated when we do not know the population standard deviation '* , 1' is used when the population is considered effectively infinite A All of the choices above 'A None of the choices above QUESTION 9 The test for normality for the distribution of proportions is that n must be greater than or equal to 10 is required because we are using the normal approximation to calculate a confidence interval is not dependent on the sample proportion requires a lower n when p is lower than .5 All of the choices above ONone of the choices above QUESTION 10 Confidence intervals are sometimes called interval estimates become narrower when n increases make use of probability to manage random sampling error are constructed around estimators All of the choices above ONone of the choices aboveQUESTION 11 Assume that the following data were generated using a valid and appropriate methodology. XYZ Data Analytics, Inc. received properly completed surveys from 523 members of the target population for a new product. One hundred seventy nine of those respondents indicated they would buy the new product at the proposed price. Based on these data, construct an interval estimate of the population proportion of the target market that would buy the new product at the proposed price using a condence level of 92.5%. The lower boundary of the interval estimate is and the upper boundary is . Round your answers to four decimal places, and do not use leading zeros. The margin of error for the interval estimate is . Round your answer to four decimal places, and do not use a leading zero. Assuming the same p would result and keeping the condence level at 92.5%, the sample size required to assure a margin of error of no more than plus or minus 2 percentage points is . Assume that the population proportion is .5, round your answer to the appropriate level, and use a comma to separate 0005, e.g. 2,445 instead of 2445