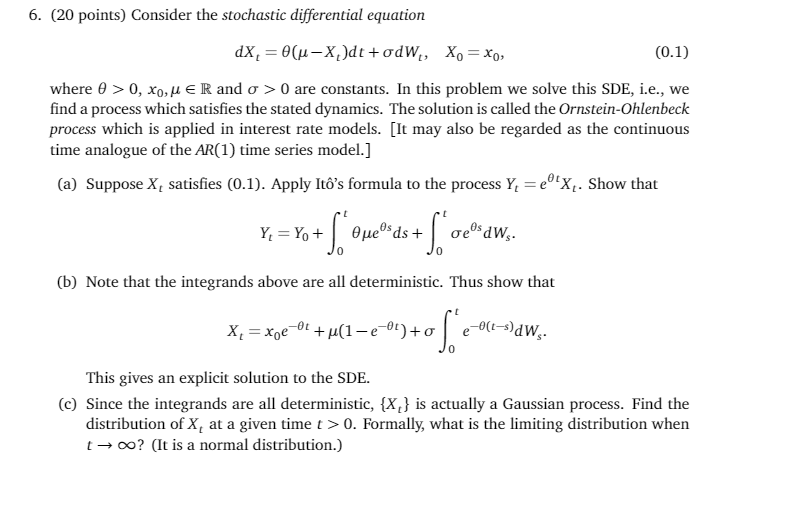
Please answer both question (a) (b) (c) in this problem

6. (20 points) Consider the stochastic differential equation dx, = 0(u-X,)dt+odw, Xo = xo, (0.1) where e > 0, xo, u R and o >0 are constants. In this problem we solve this SDE, i.e., we find a process which satisfies the stated dynamics. The solution is called the Ornstein-Ohlenbeck process which is applied in interest rate models. [It may also be regarded as the continuous time analogue of the AR(1) time series model.] (a) Suppose X, satisfies (0.1). Apply It's formula to the process Y, = ex. Show that Y = Yo + SO Oueds+ oedw.. So (b) Note that the integrands above are all deterministic. Thus show that x = xoe-ot + u(1-2-01)+o to e-01-sdw This gives an explicit solution to the SDE. (c) Since the integrands are all deterministic, {X,} is actually a Gaussian process. Find the distribution of X, at a given time t > 0. Formally, what is the limiting distribution when t 00? (It is a normal distribution.) 6. (20 points) Consider the stochastic differential equation dx, = 0(u-X,)dt+odw, Xo = xo, (0.1) where e > 0, xo, u R and o >0 are constants. In this problem we solve this SDE, i.e., we find a process which satisfies the stated dynamics. The solution is called the Ornstein-Ohlenbeck process which is applied in interest rate models. [It may also be regarded as the continuous time analogue of the AR(1) time series model.] (a) Suppose X, satisfies (0.1). Apply It's formula to the process Y, = ex. Show that Y = Yo + SO Oueds+ oedw.. So (b) Note that the integrands above are all deterministic. Thus show that x = xoe-ot + u(1-2-01)+o to e-01-sdw This gives an explicit solution to the SDE. (c) Since the integrands are all deterministic, {X,} is actually a Gaussian process. Find the distribution of X, at a given time t > 0. Formally, what is the limiting distribution when t 00? (It is a normal distribution.)