PLEASE answer the question above use the picture below for reference 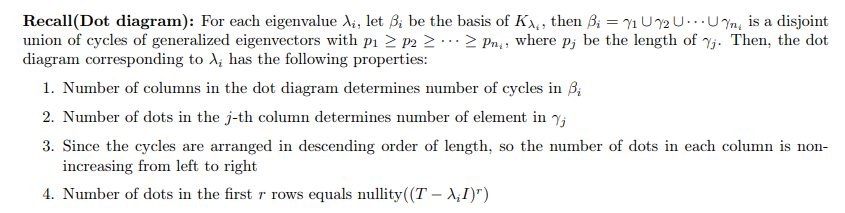
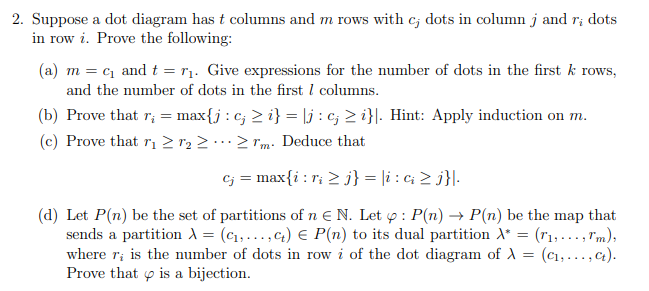
2. Suppose a dot diagram has t columns and m rows with c; dots in column j and r; dots in row i. Prove the following: (a) m = q and t = r;. Give expressions for the number of dots in the first k rows, and the number of dots in the first I columns. (b) Prove that r; = max{j:c; i} = 1;:c;> i}]. Hint: Apply induction on m. (c) Prove that r > r2 > ... > Im. Deduce that G = max{i : rij} = li: Gj}| (d) Let P(n) be the set of partitions of n E N. Let 4: P(n) + P(n) be the map that sends a partition 1 = (1,...,C) P(n) to its dual partition 1* = (1,...,m), where r; is the number of dots in row i of the dot diagram of = (C1, ...,C). Prove that y is a bijection. Recall(Dot diagram): For each eigenvalue li, let B, be the basis of KX, then B; = 71 72 U... U is a disjoint union of cycles of generalized eigenvectors with p > p2 > ... > Pn, where pj be the length of . Then, the dot diagram corresponding to li has the following properties: 1. Number of columns in the dot diagram determines number of cycles in Bi 2. Number of dots in the j-th column determines number of element in 7j 3. Since the cycles are arranged in descending order of length, so the number of dots in each column is non- increasing from left to right 4. Number of dots in the first r rows equals nullity((T ;I)") 2. Suppose a dot diagram has t columns and m rows with c; dots in column j and r; dots in row i. Prove the following: (a) m = q and t = r;. Give expressions for the number of dots in the first k rows, and the number of dots in the first I columns. (b) Prove that r; = max{j:c; i} = 1;:c;> i}]. Hint: Apply induction on m. (c) Prove that r > r2 > ... > Im. Deduce that G = max{i : rij} = li: Gj}| (d) Let P(n) be the set of partitions of n E N. Let 4: P(n) + P(n) be the map that sends a partition 1 = (1,...,C) P(n) to its dual partition 1* = (1,...,m), where r; is the number of dots in row i of the dot diagram of = (C1, ...,C). Prove that y is a bijection. Recall(Dot diagram): For each eigenvalue li, let B, be the basis of KX, then B; = 71 72 U... U is a disjoint union of cycles of generalized eigenvectors with p > p2 > ... > Pn, where pj be the length of . Then, the dot diagram corresponding to li has the following properties: 1. Number of columns in the dot diagram determines number of cycles in Bi 2. Number of dots in the j-th column determines number of element in 7j 3. Since the cycles are arranged in descending order of length, so the number of dots in each column is non- increasing from left to right 4. Number of dots in the first r rows equals nullity((T ;I)")








